Solving sin(alpha+beta)=sin(alpha)+sin(beta)

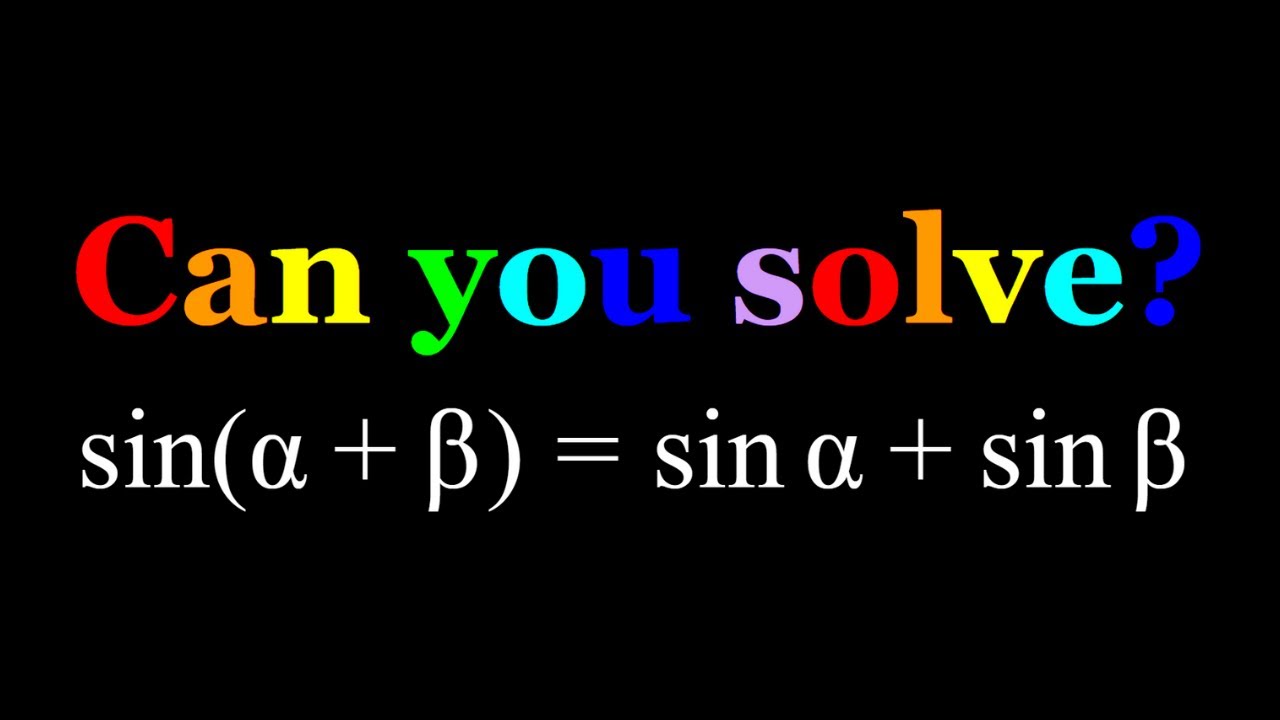
Показать описание

If you need to post a picture of your solution or idea:
#TrigonometryProblems #TrigonometricEquations

EXPLORE 😎:

PLAYLISTS 🎵 :

Рекомендации по теме

Комментарии

Nicely symmetric solution. What's about cos (alfa + beta) = cos alfa + cos beta ?
P.S. I am trying to solve this variant, but it seems to be too hard :)

panPetrff

From the the given expression : sin(A-B) = sinA - sinB then add the 2 expressions and apply the law of addition of 2 sines :
sin(A+B) + sin(A-B) = 2sinA = 2sinAcosB implies sinA(cosB -1) = 0 etc...
Remark : given expression is symmetric with respect to A and B, and trivial solutions : A=B=0, A=0 ...

WahranRai

As we can see sin(a + b) = sinacosb + sinbcosa so that cosa = 1 and cosb = 1 and we can finally find the answer is a = b = k2pi. Anyway, your answer is really nice ! Keep it up !

vanphi

sin(a+b)=sinacosb +sinbcosa. This is always less than equal to sina+sinb. That's bcoz cosa, cosb=<1. Thus in our case, cosa=cosb=1. So, we can find the general expressions for a and b.

titassamanta

nice solution. i always look forward to see your video. always coming with good ideas.

kpt

Solving before the video from the thumbnail -

sin(α+β) = sinβcosα + cosβsinα
Let cosα=1 and then sinα must be 0 making the following statement true -
sin(α+β) = sinβ + sinα
α=n2π where n ∈ *Z*
Because sinβ equals itself.
Alternatively, β=n2π where n ∈ *Z* by the same reasoning.

Time to see what I missed, it's always something. 👍😀

Edit - oh - sinθ=-sin(-θ) symmetry on either side of the cos axis. Obviously. Well - I always miss something. Thanks for the problem!

Ni

the sum of sins can turn into 2 times sin of half the sum of angles times cos half the difference of angles. and the sin of sum of angles can turn into 2 times sin of half the sum of angles times cos half the sum of angles. then take out the common factor of 2 times sin and equate to zero and solve. and then equate the cos of half the sum of angles to cos of half the difference of angles and solve

magick

Kanalın daha fazla büyümesi dileğiyle <3

dollynoob

Sin(a+b) = sinacosb + sinbcosa so cosa = cosb = 1.
So a = kpie
And b = kpie

damiennortier

Why not written the (2π-β, β)?
(Only (α, 0) & (0, β) case is so)

jarikosonen

I just went the other way around, by squaring both sides and simplifying all the way down 😅

franciscoj.f.

I dont understad where the expresion come from.

arturovinassalazar

I solved this replacing sin and cos using the euler's formula

ΒασιληςΚεκεσης

At the end of the video you made a confusing explanation for the solution alpha, 2pi-alpha.

valentinodrachuk

alpha = 0, n*pi, beta = 0, n*pi n both odd and even

sanjuiyengar

Comments from. F...If square on both sides the nature of the equation changes... without squaring on both sides it's to be solved.

visweswararoach

1:42

I'm struggling to figure out what Mike Pence has to do with trigonometry.

diedoktor

I have a simpler answer: if I sin with my GF then I sin and she also sins and we both sin together. Problem solved 😈

e

Solving sin(alpha+beta)=sin(alpha)+sin(beta)

Solving sin(alpha+beta)=sin(alpha)+sin(beta)

How to find sin(alpha + beta) when given sin(alpha) and cos(beta)

sin(alpha + beta) + sin(alpha - Beta) = 2sinacosB

✅ ✅ Have you seen this Sin(Alpha + Beta) Visualization?? ✔️👀

AMBQID134 | Question with Solution | Trigonometry | Sin Alpha Cos Alpha Sin Beta Cos Beta | AMBPi49

Prove by vector methods that `sin(alpha+beta)=sin alphacos beta+cos alphasin beta`

Proof of formula Sin(α+β)=SinαCosβ+CosαSinβ and Sin(α-β)=SinαCosβ-CosαSinβ

`(sin(alpha+beta))(sin(alpha-beta))=(a+b)(a-b)` then `(tan alpha)(tan | Class 12 Maths | Doubtnut

If `sin(alpha+beta) = 1` and `sin(alpha-beta) =1/2` where `0 lt=alpha,beta lt= pi/2` then find...

To Prove Cos(α-β), Sin(α-β), Cos(α+β), Sin(α+β) formulas using Vector Method

When mathematicians get bored (ep1)

If `alpha+beta-gamma=pi` and `sin^2alpha+sin^2beta-sin^2gamma=lambda sinalpha sinbeta cosgamma

Solve | sin 30 degree | using calculator (Casio fx-991MS)

If alpha + beta = pi/2 prove that (cos alpha + i * sin alpha)(cos beta + i * sin beta) = i

Find cos(α-β) given sin(α) and sin(β) and quadrants

if cos alpha + beta =0, then sin alpha-beta is#shorts #exemplar #class10 MCQ#trigonometry

Let `cos(alpha+beta)=(4)/(5)` and let `sin(alpha-beta)=(5)/(13)`, where `0 le alpha`, `beta=(p

sin alpha and sin beta are the solution of a cos theta + b sin theta=c The value of sinalpha sinbeta

IIT Bombay CSE 😍 #shorts #iit #iitbombay

MULTIPLE & SUBMULTIPLE ANGLES PART 11

Relation b/w Trigonometrical functions | sin cos tan cot sec | #short | #shorts | #trigonometry

🤣Modi ji ne to math ki esi taisi kar diye🤣🙆🏻‍♂️ #shorts #youtubeshorts #viral

`(sinalpha+sinbeta-sin(alpha+beta))/(sinalpha+sinbeta+sin(alpha+beta))=tan(alpha/2)tan(beta/2)`

If sin(alpha + beta) / sin(alpha - beta) = a + b / a - b then a tan beta = b tan alpha @EAG