filmov
tv
Solving for sine with no constraints
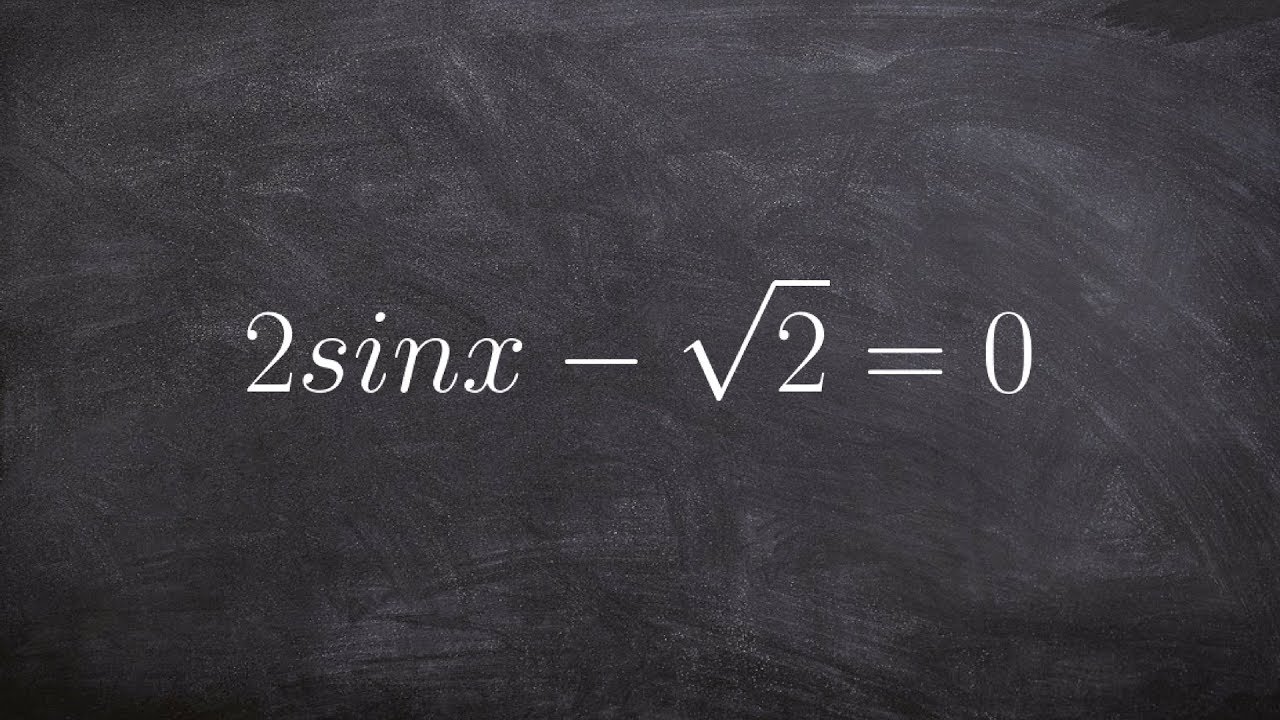
Показать описание
👉 Learn how to solve trigonometric equations. There are various methods that can be used to evaluate trigonometric identities, they include by factoring out the GCF and simplifying the factored equation. Another method is to use a trigonometric identity to reduce and then simplify the given equation. Simpler trigonometric equations involving one trigonometric function can simply be solved by inverse operations.
When solving a trigonometric equation it is usually useful to identify which method best evaluates the given equation and apply the method. Because trigonometric functions are sinusoidal in nature (continuous and repetitive), a trigonometric equation may contain many solutions depending on the domain of consideration.
Organized Videos:
✅ Solve Trigonometric Equations
✅ Solve Trigonometric Equations by Factoring GCF
✅ Solve Trigonometric Equations by Zero Product Property
✅ Solve Trigonometric Equations by Factoring
✅ Solve Trigonometric Equations by Taking the Square Root
✅ Solve a Trig Equation with Half Angles
✅ Solve a Trigonometric Equations with Multi Angles Squared
✅ Solve Trigonometric Equations all Solutions and on an Interval
✅ Solve Trigonometric Equations with Multi Angles
✅ Solve Trigonometric Equations Learn About
Connect with me:
#analytictrig #brianmlogan
When solving a trigonometric equation it is usually useful to identify which method best evaluates the given equation and apply the method. Because trigonometric functions are sinusoidal in nature (continuous and repetitive), a trigonometric equation may contain many solutions depending on the domain of consideration.
Organized Videos:
✅ Solve Trigonometric Equations
✅ Solve Trigonometric Equations by Factoring GCF
✅ Solve Trigonometric Equations by Zero Product Property
✅ Solve Trigonometric Equations by Factoring
✅ Solve Trigonometric Equations by Taking the Square Root
✅ Solve a Trig Equation with Half Angles
✅ Solve a Trigonometric Equations with Multi Angles Squared
✅ Solve Trigonometric Equations all Solutions and on an Interval
✅ Solve Trigonometric Equations with Multi Angles
✅ Solve Trigonometric Equations Learn About
Connect with me:
#analytictrig #brianmlogan
Комментарии
 0:06:42
0:06:42
 0:21:12
0:21:12
 0:04:55
0:04:55
 0:03:35
0:03:35
 0:09:53
0:09:53
 0:08:35
0:08:35
 0:05:36
0:05:36
 0:06:10
0:06:10
 0:07:47
0:07:47
 0:09:39
0:09:39
 0:07:41
0:07:41
 0:00:27
0:00:27
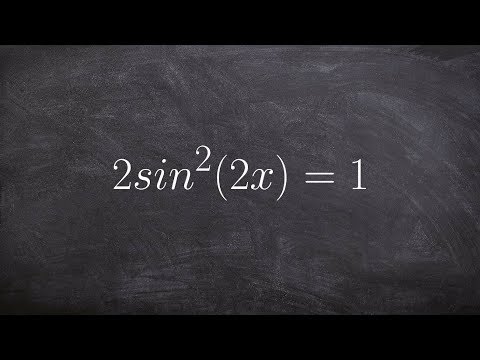 0:04:08
0:04:08
 0:00:49
0:00:49
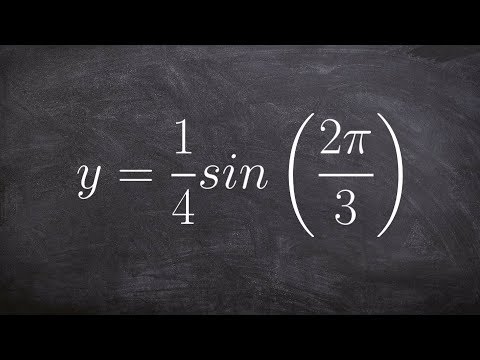 0:03:48
0:03:48
 0:10:01
0:10:01
 0:21:27
0:21:27
 0:04:35
0:04:35
 0:12:48
0:12:48
 0:04:01
0:04:01
 0:12:39
0:12:39
 0:05:22
0:05:22
 0:07:00
0:07:00
 0:18:15
0:18:15