Math 203 Lecture 26 - Triple integrals and surface area using double integrals

preview_player
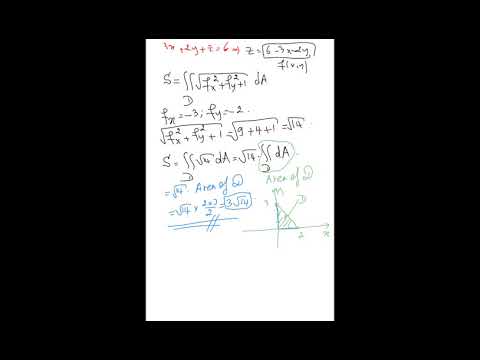
Показать описание
Description coming soon!
  1. Jhevon Smith
  2. Integral (Literature Subject)
  3. Surface Area
  4. Mathematics (Field Of Study)
  5. Multiple Integral
  6. triple integral
Рекомендации по теме
Math 203 Lecture 1:32:45

Math 203 Lecture 26 - Triple integrals and surface area using double integrals

MATH 203, LECTURE 1:20:35

MATH 203, LECTURE 26 System of Linear Differential Equations 1

Math 203 Lecture 0:49:17

Math 203 Lecture 27 - Cylindrical Coordinates

calc203 lecture 26 1:54:17

calc203 lecture 26

Math 2B. Calculus. 0:49:45

Math 2B. Calculus. Lecture 26. Representing Functions as Power Series

Math 203 26/08/2021 0:39:53

Math 203 26/08/2021

Math203_Lecture17(Surface area using 0:42:24

Math203_Lecture17(Surface area using double integrals)

Math 203 Lecture 1:15:58

Math 203 Lecture 25 - Mass, center of mass and introduction to triple integrals

Math 203 Lecture 0:20:06

Math 203 Lecture 21 - Double integrals over general regions prelecture

NEWYES Calculator VS 0:00:14

NEWYES Calculator VS Casio calculator

Microeconomics Theory I 0:39:11

Microeconomics Theory I - Lecture 26 (ECON - 203)

Math 203 Lecture 1:28:07

Math 203 Lecture 29 - Spherical Coordinates conclusion and Sequences part 1

Math 203 Lecture 1:11:08

Math 203 Lecture 3 - Vectors - their geometry, arithmetic and applications

Math 203 Lecture 1:13:48

Math 203 Lecture 23 - Double integrals over general regions and with polar coordinates

SAT Math 203 0:30:06

SAT Math 203 - Polynomials

😁 Playing 🐍Snake🐍 0:00:47

😁 Playing 🐍Snake🐍 game on calculator 😜 [official video] #shorts #viral #casio

math 203 fall 0:05:08

math 203 fall 2021 hypotheses test week

Mechanics of Materials-Lecture-26-Maximum 0:34:47

Mechanics of Materials-Lecture-26-Maximum Normal and Maximum Shear Stress States

Math 1207-R03 Lecture 1:24:14

Math 1207-R03 Lecture 26 - Intro to 3D Space (after sketching some conics)

Genius IQ Test-Maths 0:00:24

Genius IQ Test-Maths Puzzles | Tricky Riddles | Maths Game | Paheliyan with Answers | Tricky Paheli

Math 201 Lecture 1:09:39

Math 201 Lecture 26 - Derivatives and the shapes of graphs part 2 (with a little curve sketching)

Math 203 Lecture 1:33:13

Math 203 Lecture 4 - The dot product and the cross product - part 1

Chem 203. Organic 0:55:07

Chem 203. Organic Spectroscopy. Lecture 15. Coupling Involving Other Nuclei

Math 203 Lecture 1:25:33

Math 203 Lecture 24 - Double integrals with polar coordinates conclusion and series corner part 3

Комментарии
Автор

58:28 thats pretty darn cool. Could that be applied to every double/triple integral we've done so far?

kpopisthebestful
Автор

Also for that trick, 58:28, it wont work if the function is addition, like x+y+z dxdydz. And does this work for triple integrals

kpopisthebestful
Автор

I'm a bit confused on how to interpret a triple integral that holds a f(x, y, z) inside. The answer is in four dimensions so is it describing some other quantity of the object (given a respective formula) such as temperature or.. like what does it mean?

For example: A few videos back for an object with non-neglible thickness, the center of mass formula gives us a density function for the whole solid. But the answer is a number, is it an average number or a total number?

UnforsakenXII