Maximum Likelihood Estimator of Exponential Distribution Statistics

Statistics and Bayesian Statistics and Point Estimation.
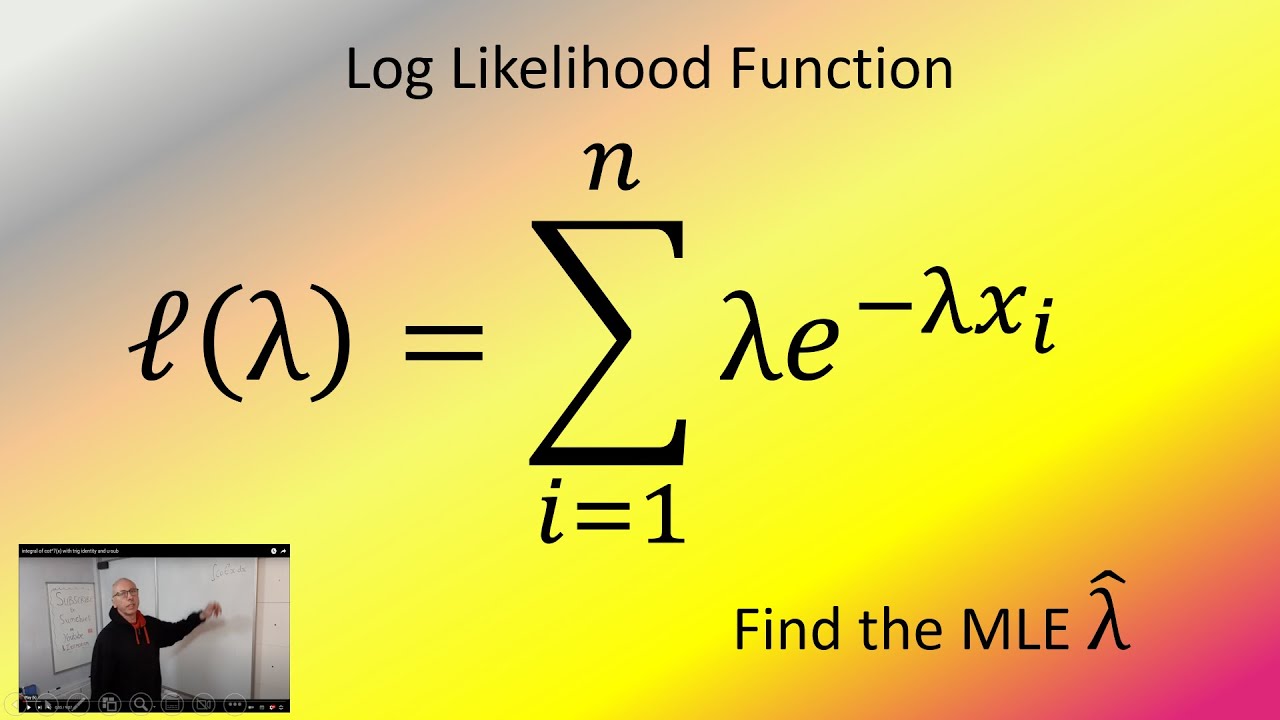
This video shows how to find the candidate for the Maximum Likelihood Estimate of lambda from the Exponential Distribution.
Next, we find the log likelihood function with respect to lambda. This will be easier to differentiate in the next few stages.
The log likelihood is then differentiated with respect to lambda, and we set l'(lambda)=0.
This function f(x|lambda) is a cumulative distribution function.
This is for x greater than 0 and lambda is greater than 0
This gives us our candidate for the MLE of lambda. also, known as hat lambda.
To check if this is a suitable candidate, we take the second derivative of the log likelihood function and see that it is less than zero always. If so, it is a suitable candidate.
#statistics
#logarithm
#discretemaths
#probabilityandstatistics
#stats
#probability_distribution
#bernoulli
#binomial_distribution
#poissondistribution
#exponential