filmov
tv
What is Circular Motion & Centripetal Acceleration in Physics? - [1-4-14]
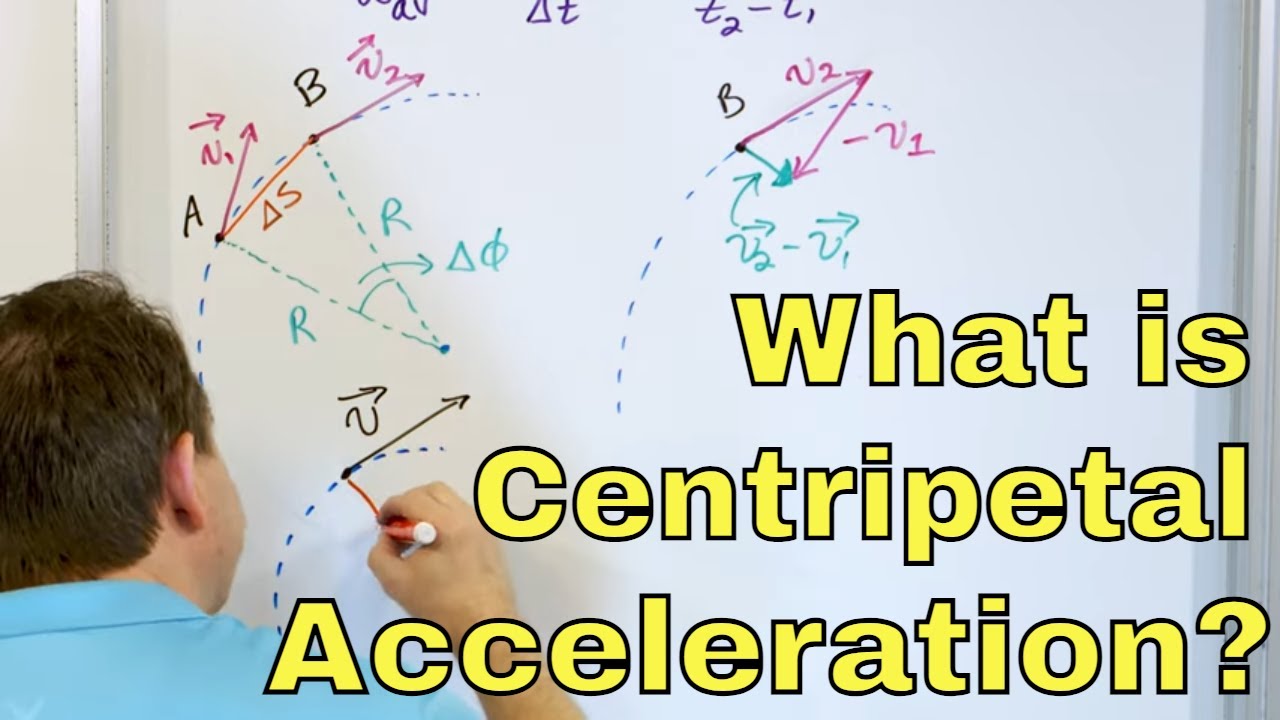
Показать описание
In this lesson, you will learn about the concept of uniform circular motion and how it gives rise to the idea of centripetal acceleration. We will use the equation for the acceleration of an object in circular motion to solve problems, then we will perform the vector arithmetic to derive this equation and prove that it is true. Centripetal acceleration and circular motion are used in all orbital mechanics calculations, any rotating mechanical object, and it is even used in the simple models of the atomic orbitals of the electrons that surround atoms.
Uniform Circular Motion: Crash Course Physics #7
What Is Circular Motion? | Physics in Motion
Understanding Circular Motion
Uniform Circular Motion Formulas and Equations - College Physics
Uniform Circular Motion and Centripetal Force
Uniform Circular Motion
What is Centripetal force?
The Science of Circular Motion: Real-life Examples and Explanations!
What is Circular Motion & Centripetal Acceleration in Physics? - [1-4-14]
Circular Motion - GCSE & A-level Physics
A Level Physics Revision: All of Circular Motion (in under 20 minutes! )
Circular Motion - GCSE Physics
Uniform Circular Motion
Centripetal Force
Circular Motion | GCSE Physics | Doodle Science
UNIFORM CIRCULAR MOTION | Animation
Circular Motion | A-Level Physics | Doodle Science
Uniform Circular Motion - IB Physics
What is Uniform circular Motion? Physics
Class 9 - Physics - Chapter 3 - Lecture 7 - 3.4 Uniform Circular Motion - Allied Schools
Uniform Circular Motion Class 11
Circular Motion | Chapter 8 | Motion | Class 9 Science | NCERT
Introduction to Circular Motion: Angular & Linear Velocity
1D10.50 Simple Harmonic Motion and Uniform Circular Motion
Комментарии
 0:09:54
0:09:54
 0:08:44
0:08:44
 0:15:36
0:15:36
 0:12:43
0:12:43
 0:06:12
0:06:12
 0:10:24
0:10:24
 0:06:24
0:06:24
 0:04:54
0:04:54
 0:42:00
0:42:00
 0:19:01
0:19:01
 0:16:29
0:16:29
 0:04:02
0:04:02
 0:09:14
0:09:14
 0:01:46
0:01:46
 0:01:53
0:01:53
 0:07:31
0:07:31
 0:04:35
0:04:35
 0:14:02
0:14:02
 0:13:07
0:13:07
 0:11:44
0:11:44
 0:26:43
0:26:43
 0:04:53
0:04:53
 0:12:39
0:12:39
 0:00:26
0:00:26