filmov
tv
Calculus 3 -- Cross product -- Practice

Показать описание
0:00 Introduction
0:47 Problem 1
7:40 Problem 2
16:32 Problem 3
23:13 Problem 4
Calculus 3 Lecture 11.4: The Cross Product
Cross Product of Two Vectors Explained!
Cross products | Chapter 10, Essence of linear algebra
The Vector Cross Product
Calculus 3: The Cross Product (Video #4) | Math with Professor V
Calculus 3: Vector Calculus in 3-D (18 of 35) What is a Cross Product?
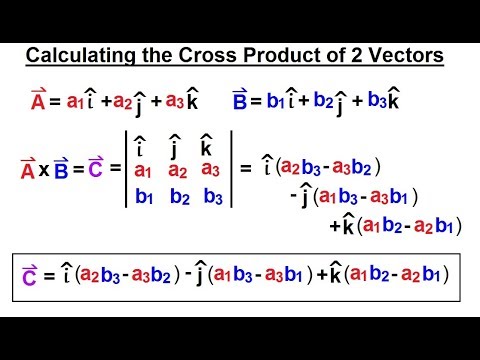
Calculus 3: Vector Calculus in 3-D (19 of 35) How to Calculate the Cross Product of 2 Vectors?
Calculus 3: Vector Calculus in 3-D (24 of 35) A Real World Example of a Cross Product
Complex: real differentiability and complex linearity in the plane, 9-3-24 part 1
ALL of calculus 3 in 8 minutes.
Cross Product - Calculus 3
12.4 Cross product | Anas Abu Zahra
Calculus 3: Vector Calculus in 3-D (25 of 35) Area of a Parallelogram Using the Cross Product
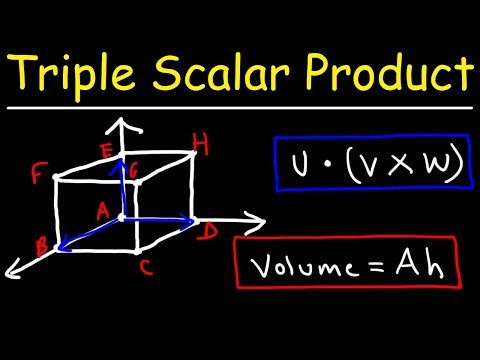
Volume of a Parallelepiped Using The Triple Scalar Product Calculus 3
Geometrically Defining the Cross Product | Multivariable Calculus
Area of a Parallelogram Using Two Vectors & The Cross Product
Calculus III: The Cross Product (Level 1 of 9) | Geometric Definition
Finding Areas Using the Cross Product (Calculus 3)
Calculus 3: Vector Calculus in 3-D (22 of 35) Theorems of Vector Products
Calculus 3 - The Dot Product
Calculus III: The Cross Product (Level 3 of 9) | Examples I
Calculus III: Cross Product
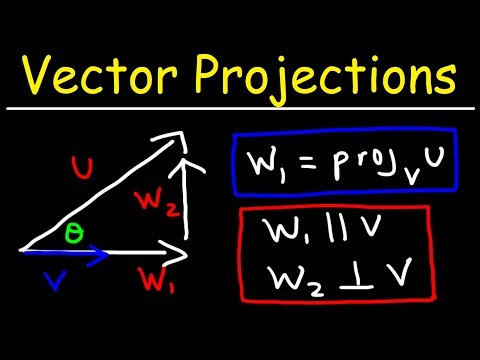
Calculus 3 - Vector Projections & Orthogonal Components
Calculus 3 -- Cross product -- Overview
Комментарии
 2:11:12
2:11:12
 0:13:47
0:13:47
 0:08:54
0:08:54
 0:06:46
0:06:46
 0:34:45
0:34:45
 0:03:29
0:03:29
 0:02:47
0:02:47
 0:06:42
0:06:42
 0:59:51
0:59:51
 0:08:10
0:08:10
 0:01:00
0:01:00
 0:31:44
0:31:44
 0:04:30
0:04:30
 0:11:40
0:11:40
 0:05:13
0:05:13
 0:06:41
0:06:41
 0:09:22
0:09:22
 0:14:31
0:14:31
 0:06:51
0:06:51
 0:13:34
0:13:34
 0:10:17
0:10:17
 0:26:59
0:26:59
 0:12:14
0:12:14
 0:38:18
0:38:18