Calculus 3: Green's Theorem (10 of 21) With a Conservative Vector Field: Ex. 2

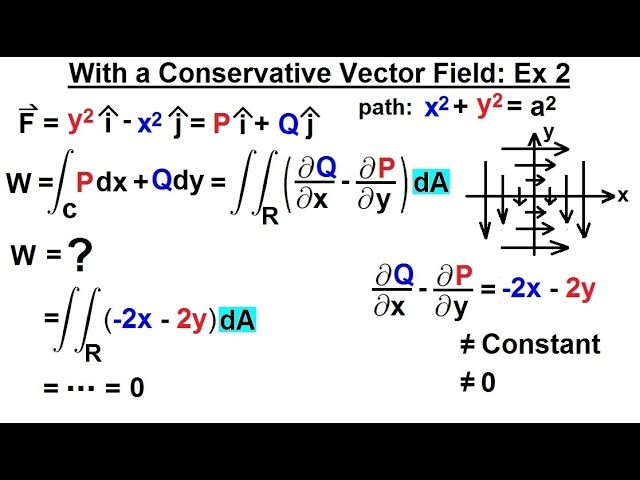
Показать описание

In this video I will find the work done in a conservative vector field by integrating along the path x^2+y^2= a^2. Ex. 2

Next video in the series can be seen at:

Рекомендации по теме

Комментарии

I am very happy to live with your lectures. Thanks a lot!!!

davidkwon

Tauseef Ahamad pointed out that Prof. Biezen made an error at the end. He made another error in stating that if the integrand (dq/dx-dp/dy) is constant, then the integral is zero. Just wanted to make that clear for the viewers scratching their heads.

jameswilson

The conclusion is wrong.The line integral is zero only for the given circular path.If some other closed path is selected, the line integral would not be zero. But for conservative fields, line integral around any arbitrary closed path is always zero.Hence the given field is not conservative.

abcd

for a vector field to be conservative, isn't there a potential function for the field in question. in this case the field has no potential function, from what i understand.

Yh-gucw

There are 2 errors in this video: when he changed to polar, he should have set x=rcos(theta), y= rsin(theta) I.e, you must integrate x+y over all of R, not just on the curve AND, the conclusion drawn is incorrect. All conservative vector fields will yield 0 work on a closed path but 0 work on any particular closed path does not imply the field is conservative.

chriswinchell

Isnt that the rcos(theta) into the x instead of acos(theta)?, for y as the same

jason

Quick question... so I didn't change to polar coordinates... just left as cartesian. got the same integrand as you -2x - 2y, but my bounds of integration were different... -sqrt(a^2 - x^2) <= y <= sqrt(a^2 - x^2) and 0 <= x <= a... I ended up with a very different answer: -4/3(a^2)^(3/2)... confirmed in wolfram alpha... (integrate [-2x - 2y] from y=-sqrt(a^2 - x^2) to sqrt(a^2 - x^2) and x=0 to a). Since the path is x^2 + y^2 = a^2, it's easy enough to get y as a function of x. Polar coords is an obvious chose given the symmetry, but where did I go off the rails here?

pipertripp

Calculus 3: Green's Theorem (10 of 21) With a Conservative Vector Field: Ex. 2

Calculus 3: Green's Theorem (10 of 21) With a Conservative Vector Field: Ex. 2

Calculus 3: Green's Theorem (21 of 21) More Examples 4

Green's Theorem, explained visually

Green's theorem example 1 | Multivariable Calculus | Khan Academy

Calculus 3: Green's Theorem (18 of 21) Using Green's Theorem to Find Area

Calc 3: Green's Theorem (Step by Step)

Green's Theorem

||Green's Theorem|| Calculus|| by Howard Anton||10th Edition|| Exercise 15.4|| Question no.3||

Vector integration Green's theorem best & easy example(PART-1)

Calc 3: Green's Theorem (TI-nSpire CX CAS)

Calculus 3: Green's Theorem (8 of 21) Graphical Representation of a Vector Field

Green's Theorem in Simple Way | Vector Calculus | Mathematics 2 New Syllabus | Maths 2 GTU

Green's Theorem #calculus #vectorcalculus

Calculus 3: Green's Theorem (11 of 21) With a NON-Conservative Vector Field: Ex. 2

|| Vector Calculus || Lecture-10 || Green's Theorem || Dr Sonendra Gupta ||

Calculus 3: Green's Theorem (9 of 21) A Conceptual Look

Calc 3: Green's Theorem (Solved by Hand)

Calculus 3: Green's Theorem (13 of 21) With a NON-Conservative Vector Field: Ex. 3 (Line Integr...

Multivariable Calculus - Using Green’s Theorem

Green's Theorem Example | Calculus 3 | Vector Calculus

Calculus 3: Green's Theorem (6 of 21) With a Conservative Vector Field

Calculus 3, Session 34 -- Green's Theorem

Divergence, Flux, and Green's Theorem // Vector Calculus

Green's Theorem|| Calculus|| by Howard Anton||10th Edition|| Exercise #15.4|| Question no.1||