Real Analysis | Uniform Convergence and Continuity

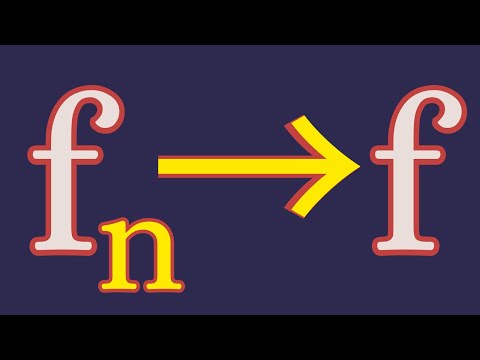
We prove that if a sequence of continuous functions converges uniformly, then its limit is also a continuous function. We also prove the Cauchy Criterion for uniformly convergent sequences of functions.

If you are going to use an ad-blocker, considering using brave and tipping me BAT!

Books I like:

Abstract Algebra:

Differential Forms:

Number Theory:

Analysis:

Calculus:

My Filming Equipment:

[lvlanson]

Very nicely explained, thank you Michael!

[alexmason]

I really love your channel, it is midnight by the way.🙂

[kevin]

Another good video. One small problem, the "<" you claimed in 17:51 should be a "<=", that is, a "less-than-or-equal-to, " since you're taking the limit. Though, it's not big of a deal since you can take epsilon to be epsilon/2. Then, the proof is fine.

[Evan-nebu]

Hello Micheal, i really like this playlist, and I have a question: will talk about (maybe not going to deep) measure theory?

[TheMauror]

I missed the real analysis videos. Great video though!!

[alphalunamare]

Very Lucid! I am retired now, it's a long time since I studied Mathematics but I find your presentation so easy to follow, especially the way you explain the what goes on between the lines of the proof, something you rarely find in either books or the average lecturer. - well in those day's at least. They should treble your Salary :-)

[heberfarnsworth]

Question. When you use continuity of f_N at a you assume that the epsilon doesn't depend on N. Is that a problem?

[CM_France]

Hi,

For fun:
12 "let's go ahead and", including 3 "so let's go ahead and do that", and 1 "so now let's go ahead and do that",
1 "let's may be go ahead and write that down",
1 "I want to go ahead and",
1 "I can go ahead and",

6 "great",

1 "now the thing I want to do",
1 "now what we are going to do",
1 "now I want to notice".

[monku]

Doesnt fn(x) = x^n converge uniformly to f where f maps [0, 1) to 0 and 1 to 1, but still isn’t continuous?

[jonaskoelker]

So something struck me:

Pointwise convergence says for all epsilon > 0 for all x exists N in IN such that
[insert conclusion(x, N, epsilon)]
Uniform convergence says for all epsilon > 0 exists N in IN such that for all x:
[insert conclusion(x, N, epsilon)]

Another way of saying this is:

View N as a function of epsilon and x, N = g(epsilon, x).
Fix a particular epsilon and project out g_epsilon(x).

In pointwise convergence, g_epsilon can be any function of x.
In uniform convergence, g_epsilon must be a constant function of x.

Viewed this way, a question naturally arises: the requirements are at two extremes. What if we impose much more intermediate requirements on g_epsilon, like monotonicity, continuity, differentiability? Oh wait g_epsilon: IR -> IN.

How about this:
for every x and every epsilon > 0
there exists delta > 0 and N in IN such that
for every y
if |y-x| < delta then [insert conclusion(y, N, epsilon)]

I feel tempted to call this local uniformity:
uniform convergence lets us conclude conclusion(y, N, epsilon) given conclusion(x, N, epsilon) for _every_ y.
Locally uniform convergence lets us conclude conclusion(y, N, epsilon) only when y is sufficiently close to x.

I think local uniformity is sufficient for many purposes, since many of the properties we want to prove—chiefly continuity and differentiability—are local in some way (e.g. pointwise).

I suspect most proofs that assume uniform convergence become proofs built on local uniformity if you let delta' = min(delta1, delta2) as an additional proof step.

Does anyone know if this concept has been explored? It should be easier to prove than global uniformity since global implies local, and thus more function sequences should be locally uniform, and thus we can conclude continuity, differentiability etc. about a greater number of functions.

Has my idea been tried and found wanting?

[laurensiusfabianussteven]

is it true that the proof for continuity of uniform convergent sequence fn(x) can be extended to if and only if statement?

[learnmatheasily]

Q.1. Prove or give a counter example that uniform continuity preserves convergence
of sequences.
Q. 2. Prove or give a counter example that continuity preserves the length of an
interval.
Q. 3. Let 𝑓(𝑥) = [𝑥 + 1] − 𝑥 − 1, ∀𝑥 ∈ 𝑅
Where [𝑥] represents the smallest integer ≥ 𝑥. Determine all points of discontinuity
and continuity of the function.
Q. (4). Consider 𝑓(𝑥) = sin(𝑥), 𝑥 ∈ [
−𝜋
4
,
5𝜋
4
] (10)
(a). Determine whether Rolle’s theorem or the Lagrange’s Mean value theorem is
applicable here. Explain
(b). Apply the theorem that is applicable and find all values of c that satisfy the
statement. Also draw a rough sketch of what you get in this discussion. (you can use
calculator to compute values of inverse trigonometric functions)
Q. 5. Prove or give a counter example that differentiable function over an interval is
bounded.
Q. 6. Consider 𝑓(𝑥) =
𝑥
3
3
−
3𝑥
2
2
+ 2𝑥 + 1.
Use derivatives to separate the regions where function is increasing or decreasing.
Q. 7. Let 𝑓(𝑥) be derivable over [𝑎, 𝑏] such that 𝑓
′
(𝑎) = 10 and 𝑓
′
(𝑏) = 20 . Prove
that there exist 𝑐 ∈ (𝑎, 𝑏) such that 𝑓
′
(𝑐) = 15.

[rektator]

This essentially shows that the metric space (C(X, Y), e), of continuous functions from a compact space X to a complete metric space (Y, d), equipped with the supremum metric e [e(f, g) := sup_{x\in X}d(f(x), g(x)) for f, g\in C(X, Y)) defines a complete metric space. The completeness of C(X, Y) is important for example in the proof of Existence and Uniqueness theorem of first order differential equations. The completeness of C(X, Y) makes it possible to use Banach fixed point theorem to find solutions to the differential equations when the underlying vector field of the differential equation is Lipschitz continuous.

[kurax]

I really Like these Videos. Im so bad when it comes to creating ideas for proofs
although i already knew how to do the first two its amazing to see such content

[ashimchakraborty]

Can someone please solve the problem??
A sequence a_n is defined by a_1=1, a_2=12, a_3= 20, and a_(n+3) = 2a_(n+2) + 2a_(n+1) − a_n,
n > 0. Prove that, for every n, the integer 1 + 4 a_n a_(n+1) is a square.

[ethannguyen]

Oh don’t mind me. I’m just waiting for him to get demonetized for saying “left” and “right.”