filmov
tv
Calculus students: a required classic -- solid of revolution
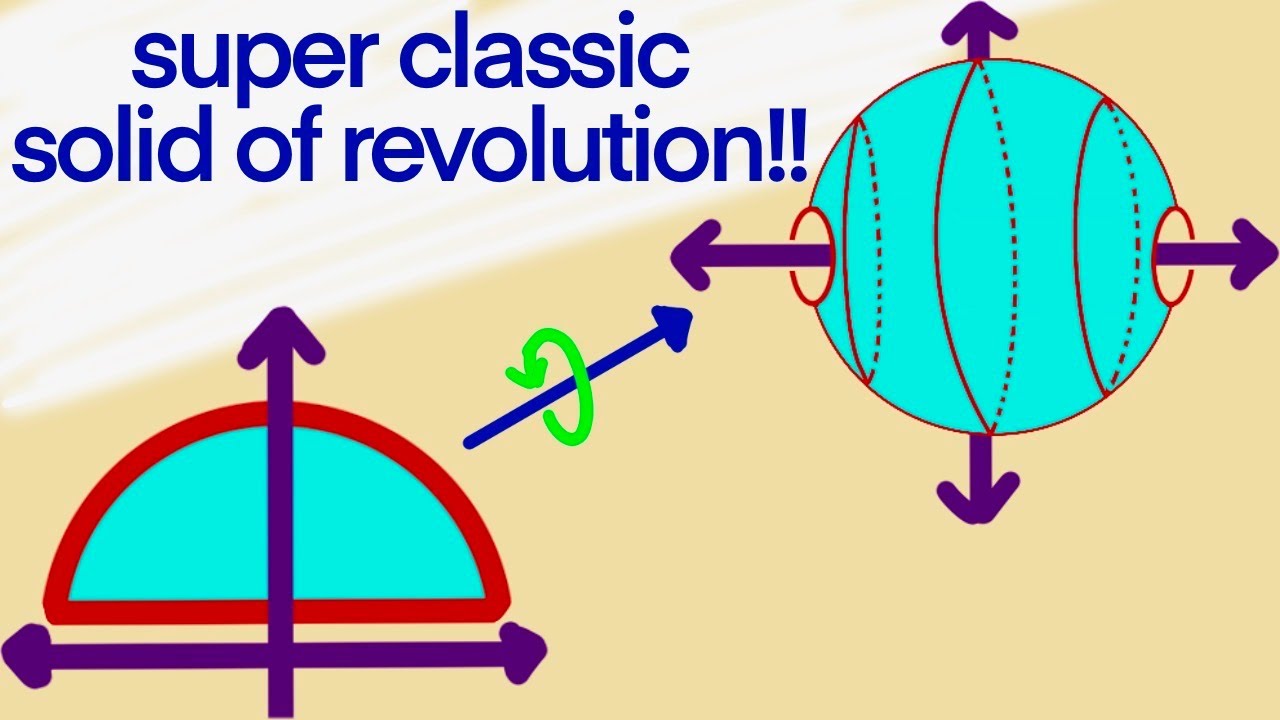
Показать описание
🌟Support the channel🌟
🌟my other channels🌟
🌟My Links🌟
🌟How I make Thumbnails🌟
🌟Suggest a problem🌟
Calculus students: a required classic -- solid of revolution
Most Calculus Students Can't Solve This
A Treatise on Advanced Calculus by Franklin 1st Edition 1940
generalizing a classic Calculus limit.
Mastering Calculus with the 1968 Classic: Basic Calculus
Calculus is Required
Calculus SPEEDRUN (U-Sub)!!
Solutions from my first Calculus 1 exam
Language of Limits and Continuity: Holes, Jumps, Asymptotes, and Wild Behavior
The Best Way to Learn Calculus
Classic AP calculus problems!
How calculus students entertained themselves in the early 2000’s
Classic Tricky AP Calculus AB Problem (given f', answer about f)
Calculus for Everyone: Students value Calculus
Calculus BC’s first and only group quiz #nycschools #calculus #highschool
Gabriel's Horn -- Classic Calculus
AP Calculus AB vs BC: Which Class is Best For You (in 10 minutes)?
LEGENDARY integral in less than 1 minute #maths #calculus #integration #stem #education
Calculus: A New Horizon
Infinitesimal Calculus by Lamb #shorts
Math and Calculus Students be like 👍 #Shorts
Jokes!!! #jokes #calculus #funnyvideo #funny #funnyshorts #funnyvideos #math #mathematics #maths
Differentiation|| #shorts #gujcet #kcet #keam #jeemains #bitsat #bseb12th #cbse12th #calculus #nda
Calculus Review 2 #Shorts #math #maths #mathematics #calculus #education #learn
Комментарии
 0:10:01
0:10:01
 0:11:36
0:11:36
 0:00:53
0:00:53
 0:08:37
0:08:37
 0:09:01
0:09:01
 0:14:39
0:14:39
 0:01:00
0:01:00
 0:24:42
0:24:42
 1:03:20
1:03:20
 0:10:11
0:10:11
 0:01:16
0:01:16
 0:00:51
0:00:51
 0:09:46
0:09:46
 0:00:34
0:00:34
 0:00:18
0:00:18
 0:00:53
0:00:53
 0:10:37
0:10:37
 0:00:41
0:00:41
 0:00:58
0:00:58
 0:00:45
0:00:45
 0:00:14
0:00:14
 0:00:06
0:00:06
 0:00:58
0:00:58
 0:00:15
0:00:15