AP Precalculus Practice Test: Unit 3 Question #29 Transforming a Tangent Function on a Graph

My AP Precalculus Practice Tests are carefully designed to help students build confidence for in-class assessments, support their work on AP Classroom assignments, and thoroughly prepare them for the AP Precalculus exam in May.

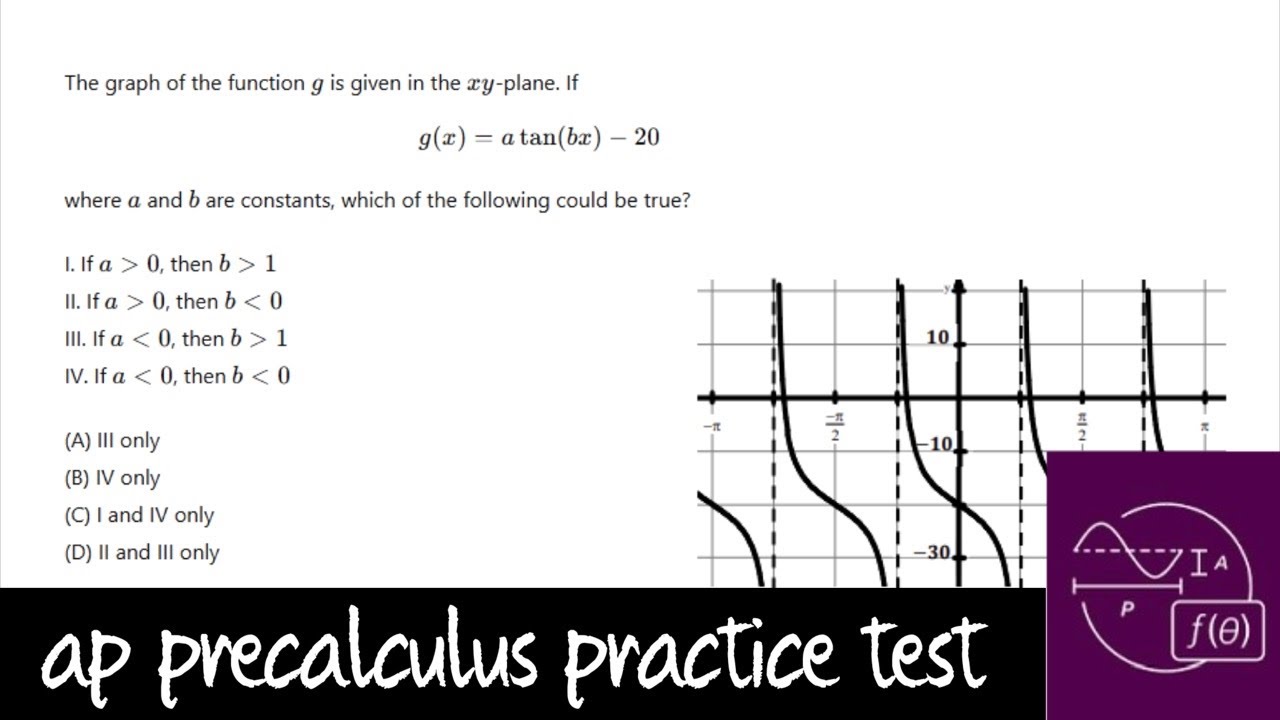
**AP Precalculus Practice Test: Unit 3, Question #29** involves describing the transformation of a tangent function on a graph. Tangent functions are expressed as \( y = a \tan(b(x - h)) + k \), where each parameter alters specific properties of the graph.

---

### **Key Transformations of a Tangent Function**
1. **Vertical Stretch or Compression (\(a\))**:
- The value of \(a\) determines the steepness of the graph.
- Larger values of \(a\) increase the steepness (vertical stretch).
- Smaller values of \(a\) decrease the steepness (vertical compression).
- Negative values of \(a\) reflect the graph over the horizontal axis.

2. **Horizontal Stretch or Compression (\(b\))**:
- The value of \(b\) affects the period of the tangent function.
- The period is calculated as \( \frac{\pi}{b} \).
- Larger values of \(b\) shorten the period (horizontal compression).
- Smaller values of \(b\) lengthen the period (horizontal stretch).

3. **Horizontal Shift (\(h\))**:
- The value of \(h\) shifts the graph left or right.
- Positive values of \(h\) shift the graph to the right.
- Negative values of \(h\) shift the graph to the left.

4. **Vertical Shift (\(k\))**:
- The value of \(k\) shifts the graph up or down.
- Positive values of \(k\) shift the graph upward.
- Negative values of \(k\) shift the graph downward.

---

### **Example Problem**
Transform \( y = \tan(x) \) into \( y = -2 \tan(3(x - \frac{\pi}{4})) + 1 \).

1. **Vertical Stretch and Reflection**:
- The coefficient \(a = -2\) causes a vertical stretch by a factor of 2 and reflects the graph over the horizontal axis.

2. **Horizontal Compression**:
- The coefficient \(b = 3\) compresses the graph horizontally, reducing the period to \( \frac{\pi}{3} \).

3. **Horizontal Shift**:
- The term \( (x - \frac{\pi}{4}) \) shifts the graph to the right by \( \frac{\pi}{4} \).

4. **Vertical Shift**:
- The constant \(+1\) shifts the graph upward by 1 unit.

---

### **Description**:
"The transformation involves a vertical stretch by a factor of 2, a reflection over the horizontal axis, and a horizontal compression that reduces the period to \( \frac{\pi}{3} \). Additionally, the graph shifts to the right by \( \frac{\pi}{4} \) units and moves upward by 1 unit. The resulting function is \( y = -2 \tan(3(x - \frac{\pi}{4})) + 1 \)."

I have many informative videos for Pre-Algebra, Algebra 1, Algebra 2, Geometry, Pre-Calculus, and Calculus. Please check it out:

/ nickperich

Nick Perich
Norristown Area High School
Norristown Area School District
Norristown, Pa

#math #algebra #algebra2 #maths #math #shorts #funny #help #onlineclasses #onlinelearning #online #study