filmov
tv
Probability Theory 13 | Independence for Random Variables

Показать описание
Please consider to support me if this video was helpful such that I can continue to produce them :)
🙏 Thanks to all supporters! They are mentioned in the credits of the video :)
This is my video series about Probability Theory. I hope that it will help everyone who wants to learn about it.
This video is about probability theory, also known as stochastics, stochastic processes or statistics. I keep the title in this general notion because I want cover a lot of topics with the upcoming videos.
Here we talk about independence again, namely about independent random variables. First we discuss two random variables and then infinitely many random variables.
#ProbabilityTheory
#Analysis
#Calculus
#Mathematics
I hope that this helps students, pupils and others. Have fun!
(This explanation fits to lectures for students in their first and second year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)
Probability Theory 13 | Independence for Random Variables
Probability Theory 13 | Independence for Random Variables [dark version]
Probability Theory 9 | Independence for Events
Introduction to Probability, Basic Overview - Sample Space, & Tree Diagrams
Probability Theory 13 What is Conditional Probability?
Probability Theory Lecture 13
Probability Theory Lecture 8 - Independent Events Part 1
Independent Events (Basics of Probability: Independence of Two Events)
Probability Part 1: Rules and Patterns: Crash Course Statistics #13
Independence (probability theory)
Conditional and Independent random variables | Lecture 13 | Probability Course
Mod-01 Lec-13 INDEPENDENCE CONTD.
What is independence (probability)?
Conditional probability and independence | Probability | AP Statistics | Khan Academy
Probability Theory (Independent Random Variables)
Independence test for probability
Probability Theory 16 Independence of Events
13B Conditional probability & independence
13. Probability Theory ESSFAR
How Casinos Exploit Our Limited Understanding of Statistics & Probability | Neil deGrasse Tyson
Conditional probability
Probability Theory: 2.5 Independence (I/II)
Probability Theory - Summary of Lecture 13
Probability of Complementary Events & Sample Space
Комментарии
 0:10:15
0:10:15
 0:10:15
0:10:15
 0:11:26
0:11:26
 0:16:59
0:16:59
 0:30:43
0:30:43
 0:24:34
0:24:34
 0:11:46
0:11:46
 0:21:25
0:21:25
 0:12:01
0:12:01
 0:09:43
0:09:43
 0:03:38
0:03:38
 0:41:05
0:41:05
 0:01:25
0:01:25
 0:04:07
0:04:07
 0:26:16
0:26:16
 0:04:57
0:04:57
 0:14:23
0:14:23
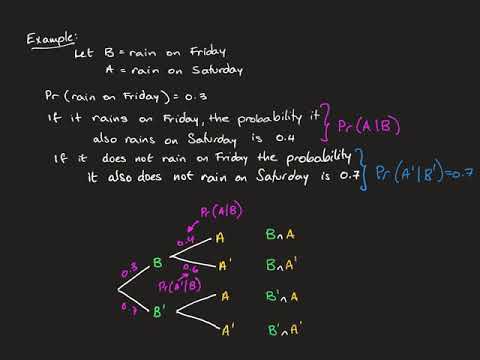 0:15:58
0:15:58
 2:56:41
2:56:41
 0:01:00
0:01:00
 0:00:05
0:00:05
 0:18:13
0:18:13
 0:02:32
0:02:32
 0:05:48
0:05:48