filmov
tv
Find the remainder when 3^287 is divided by 23| Theory of Congruence| Number Theory @MathsAnalysis
Показать описание
Find the remainder when 3^287 is divided by 23.
Find the remainder when 3 to the power 287 is divided by 23.
जब 3^287 को 3 से भाग देंगे तो शेषफल क्या होगा।
Number Theory || Maths Analysis || Theory of Congruence
#NumberTheory #MathsAnalysis #TheoryOfCongruence #BScMaths
Find the remainder when 3 to the power 287 is divided by 23.
जब 3^287 को 3 से भाग देंगे तो शेषफल क्या होगा।
Number Theory || Maths Analysis || Theory of Congruence
#NumberTheory #MathsAnalysis #TheoryOfCongruence #BScMaths
0:04:24
Find the remainder when 3^287 is divided by 23| Theory of Congruence| Number Theory @MathsAnalysis
0:09:35
How to find remainder by using modulo function // DSSSB tgt / pgt maths Htet tgt / pgt maths
0:03:43
How to Find the Remainder With the Help of Congruence | Number Theory
0:00:29
If (27)^999 is divided by 7, then the remainder is:
0:02:34
Number theory :Remainder finding
0:00:50
Remainder when 3^2022 is divided by 5 #shorts #mathshorts #remaindertrick
0:25:49
#6 Remainder Theorem (Part 6) | Fermat's little theorem - Remainder in 5 sec if divided by pri...
0:11:12
Finding remainder by using congruence, Number Theory, Lecture-56 by Prof. TM Qadri
0:21:09
Lec 16 problems of divisibility and remainder using theory of congruence #numbertheory
0:00:20
Multiples of 8 😎 #Shorts #math #maths #mathematics
0:20:17
Lec 15 finding remainder and divisibility using theory of congruence #numbertheory #higherarithmetic
0:08:42
Solving Fermat's Little Theorem The Easy Way.😀
0:16:20
4 6 congruence problem
0:05:20
Find a Remainder Using Congruences: 3^(123)/7 (Two Versions)
0:03:14
The remainder when 3^21 is divided by 5 is
0:03:24
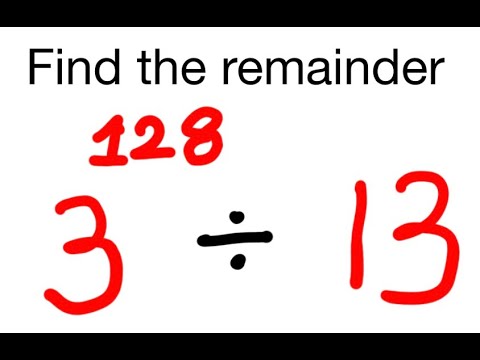
Find the remainder when 3^128 is divided by 13
0:00:25
Cube Root | How to find cube root | Cube Root Kaise Nikale | Maths Tricks #shorts #cubetricks
0:03:31
Math olympiad | Number Theory Problem | What is the remainder when 3^12 + 5^12 is divided by 13 ?
0:00:36
find the remainder when 4^23 divided by 3
0:06:09
By using congruence, find the remainder when 3^101 is divided by 10. Number Theory, Lecture-55
0:01:00
Find the remainder when the sum s= 1!+2!+3!+.. +1000! is divisible by 8.
0:06:12
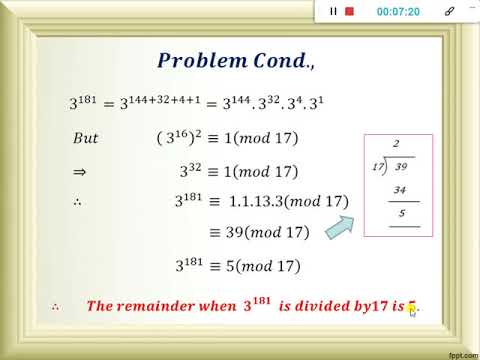
Find the Remainder when 3^247 is divided by 17 | Remainder Theorem
0:03:50
Find remainder when 3^2021 is divided by 31 | Remainder by Fermat's Theorem
0:00:07
Divisibility Rules l Mathematics Divisibility Rules
Комментарии