AP Precalculus Practice Test: Unit 2 FRQ #2 TI-84+, Exponential Regression, Population

My AP Precalculus Practice Tests are carefully designed to help students build confidence for in-class assessments, support their work on AP Classroom assignments, and thoroughly prepare them for the AP Precalculus exam in May.

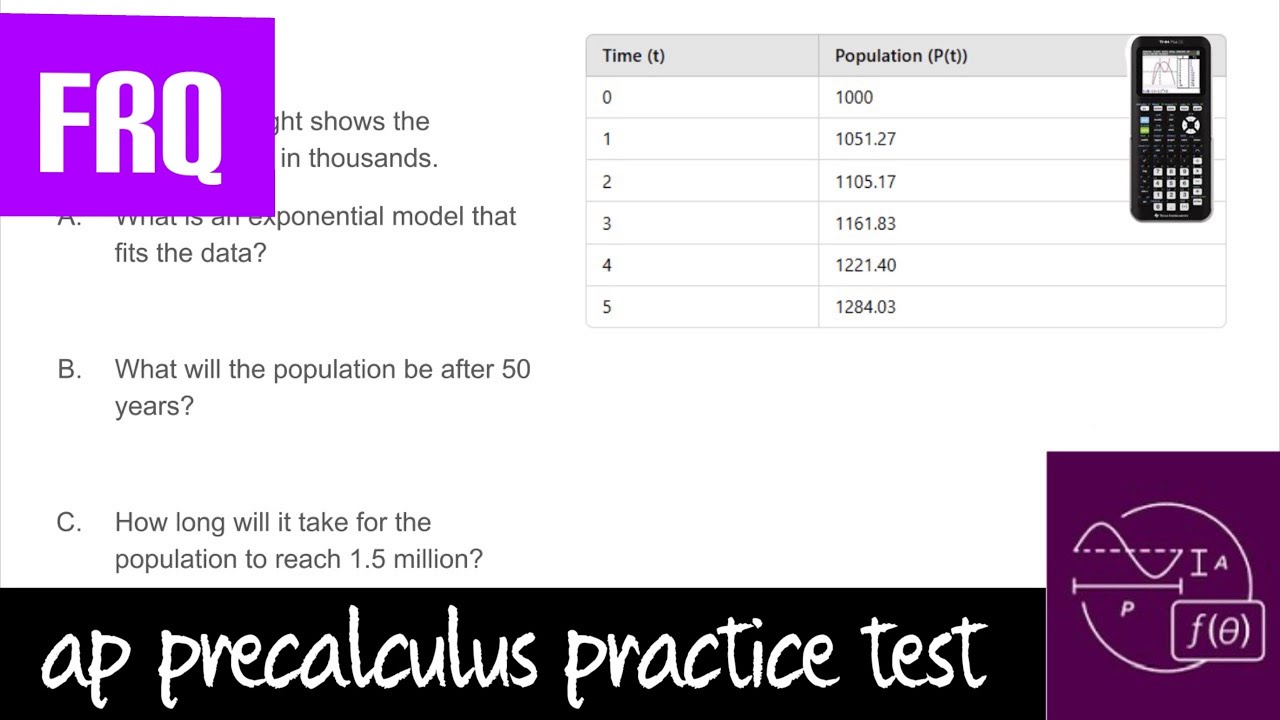
### AP Precalculus Practice Test: Unit 2 FRQ #2 - TI-84+, Exponential Regression, Population

This problem typically involves using a TI-84+ calculator to perform exponential regression on data, typically population data, and then interpreting the results.

---

### Problem Overview:
You're given a table of data (such as population growth over time) and asked to find an exponential regression model using the TI-84+. The exponential model has the form:

\[
y = ab^x
\]

Where:
- \( y \) is the dependent variable (e.g., population),
- \( x \) is the independent variable (e.g., time),
- \( a \) is the initial value (the value of \( y \) when \( x = 0 \)),
- \( b \) is the growth rate.

### Steps to Solve:

1. **Input Data into the TI-84+**:
- Press the **STAT** button.
- Select **1:Edit** to enter your data.
- Input the values of \( x \) (independent variable) in **L1** and the corresponding \( y \) (dependent variable) values in **L2**.

2. **Perform Exponential Regression**:
- After entering the data, press **STAT** again.
- Scroll over to **CALC** and choose **0: ExpReg** (Exponential Regression).
- For **Xlist**, use **L1**, and for **Ylist**, use **L2**.
- Press **Enter** to get the regression results.

3. **Interpret the Results**:
The TI-84+ will display the regression model in the form:

\[
y = a \cdot b^x
\]

Where:
- \( a \) is the initial value (population at \( x = 0 \)),
- \( b \) is the growth factor (rate of change of population).

The calculator will also give you the **correlation coefficient** (denoted \( r \)), which indicates how well the model fits the data. A value of \( r \) close to 1 suggests a strong fit.

4. **Use the Model for Predictions**:
- Once you have the exponential regression equation, you can use it to predict future values by plugging in the appropriate \( x \)-value (time).

---

### Example:

**Given Data**:

| Year (x) | Population (y) |
|----------|----------------|
| 0 | 1000 |
| 1 | 1200 |
| 2 | 1440 |
| 3 | 1728 |
| 4 | 2073.6 |

1. **Enter the Data**:
- Press **STAT**, then **1: Edit**.
- Input the years in **L1** and the populations in **L2**.

2. **Perform Exponential Regression**:
- Press **STAT**, then move to **CALC** and select **0: ExpReg**.
- Choose **L1** for the **Xlist** and **L2** for the **Ylist**, then press **Enter**.

3. **Interpret Results**:
Suppose the calculator gives the following output:
\[
y = 1000 \cdot 1.2^x
\]

- Here, \( a = 1000 \) (the initial population when \( x = 0 \)),
- \( b = 1.2 \) (the growth factor, meaning the population increases by 20% each year).

4. **Prediction**:
To predict the population in year 5, substitute \( x = 5 \) into the model:

\[
y = 1000 \cdot 1.2^5 \approx 2488.32
\]

So, the population in year 5 is approximately **2488**.

---

### Summary:
- Use the TI-84+ to perform exponential regression on the given data.
- The regression model has the form \( y = ab^x \), where \( a \) is the initial population and \( b \) is the growth rate.
- Use the model to predict future values by substituting \( x \) with the desired time value.

I have many informative videos for Pre-Algebra, Algebra 1, Algebra 2, Geometry, Pre-Calculus, and Calculus. Please check it out:

/ nickperich

Nick Perich
Norristown Area High School
Norristown Area School District
Norristown, Pa

#math #algebra #algebra2 #maths #math #shorts #funny #help #onlineclasses #onlinelearning #online #study