filmov
tv
Find the Cross Product of Two Vectors (Easy Shortcut with i j k)
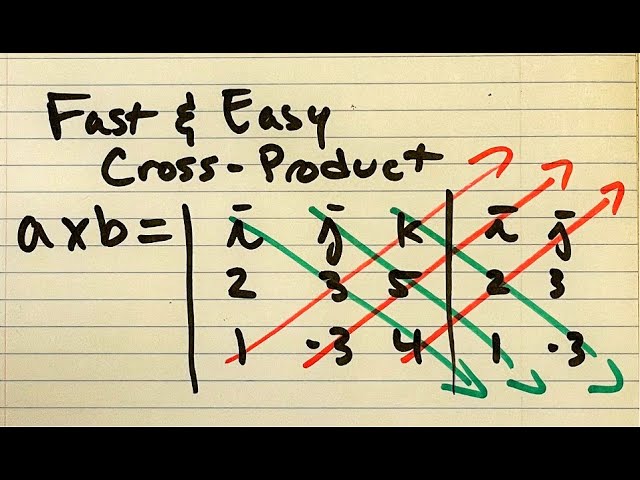
Показать описание
Is the matrix determinant method too much work? Need something simpler to calculate those pesky cross products? Or do you just want a consistent way to calculate them? If any of those are a 'yes,' then this video is for you.
Physics, engineering, math and even computer science all use cross products. If you’re studying in a STEM field, it’s very useful to know how to compute this.
Do you have another method you use to calculate cross products? Do you actually *like* finding the cross product with matrices? Maybe you have another neat math shortcut you use. Let me know in the comments! Stay tuned for more videos, too.
Song credit:
Space Racer - Bad Snacks
#math #mathematics #algebra #vectors #calculus #mattmath
Physics, engineering, math and even computer science all use cross products. If you’re studying in a STEM field, it’s very useful to know how to compute this.
Do you have another method you use to calculate cross products? Do you actually *like* finding the cross product with matrices? Maybe you have another neat math shortcut you use. Let me know in the comments! Stay tuned for more videos, too.
Song credit:
Space Racer - Bad Snacks
#math #mathematics #algebra #vectors #calculus #mattmath
Cross Product of Two Vectors Explained!
The Vector Cross Product
Find the Cross Product of Two Vectors (Easy Shortcut with i j k)
How to Find the Cross Product of Two Vectors
Cross products | Chapter 10, Essence of linear algebra
Find the Cross Product of Unit Vectors
Cross product, determinant method
How to Calculate the Vector Cross Product
Geometric Interpretation of the Cross Product
Cross Product of Two Vectors | Physics
Calculus 3 Lecture 11.4: The Cross Product
What is the CROSS PRODUCT and how to find the cross product of two vectors
The Cross Product
Find the Vector cross product of two vectors:
Area of a Parallelogram Using Two Vectors & The Cross Product
How to Find the Cross Product of Unit Vectors
Cross Product of Two Vectors Formula (3D Example)
Given the Cross Product , Find Angle Between Vectors
Application of the Cross Product: Torque
How do we generalize the cross product to other dimensions?
Ex: Find the Cross Product of Two Vectors
Normal Vector and Cross Product
Vector cross product signs
How to Calculate the Cross Product of Two Vectors (THE EASY WAY!)
Комментарии
 0:13:47
0:13:47
 0:06:46
0:06:46
 0:10:41
0:10:41
 0:03:48
0:03:48
 0:08:54
0:08:54
 0:02:18
0:02:18
 0:03:32
0:03:32
 0:41:33
0:41:33
 0:26:56
0:26:56
 0:10:37
0:10:37
 2:11:12
2:11:12
 0:10:20
0:10:20
 0:08:05
0:08:05
 0:05:51
0:05:51
 0:06:41
0:06:41
 0:02:16
0:02:16
 0:02:45
0:02:45
 0:03:37
0:03:37
 0:07:42
0:07:42
 0:07:48
0:07:48
 0:05:52
0:05:52
 0:02:06
0:02:06
 0:00:42
0:00:42
 0:03:08
0:03:08