Boussinesq approximation (water waves) | Wikipedia audio article

This is an audio version of the Wikipedia Article:


00:01:03 1 Boussinesq approximation
00:02:41 2 Original Boussinesq equations
00:07:46 2.1 Derivation
00:07:53 2.2 Linear frequency dispersion
00:08:04 3 Boussinesq-type equations and extensions
00:08:15 4 Further approximations for one-way wave propagation
00:09:46 5 Numerical models
00:14:19 6 Notes
00:15:55 7 References
00:17:05 Further approximations for one-way wave propagation
00:17:59 Numerical models



Listening is a more natural way of learning, when compared to reading. Written language only began at around 3200 BC, but spoken language has existed long ago.

Learning by listening is a great way to:
- increases imagination and understanding
- improves your listening skills
- improves your own spoken accent
- learn while on the move
- reduce eye strain

Now learn the vast amount of general knowledge available on Wikipedia through audio (audio article). You could even learn subconsciously by playing the audio while you are sleeping! If you are planning to listen a lot, you could try using a bone conduction headphone, or a standard speaker instead of an earphone.

Listen on Google Assistant through Extra Audio:
Other Wikipedia audio articles at:
Upload your own Wikipedia articles through:
Speaking Rate: 0.9830981425693882
Voice name: en-AU-Wavenet-B


"I cannot teach anybody anything, I can only make them think."
- Socrates


SUMMARY
=======
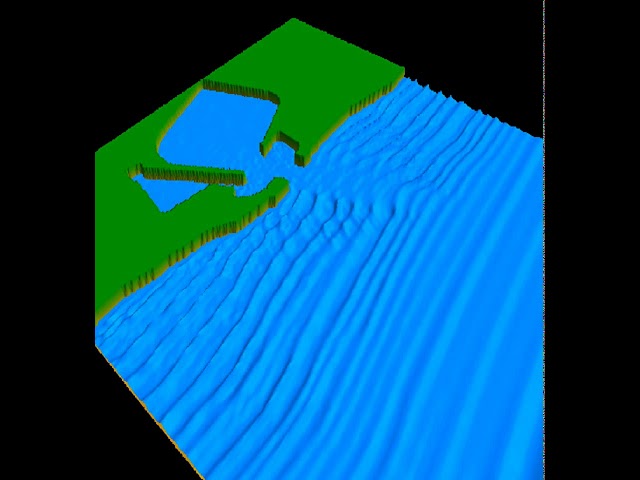
In fluid dynamics, the Boussinesq approximation for water waves is an approximation valid for weakly non-linear and fairly long waves. The approximation is named after Joseph Boussinesq, who first derived them in response to the observation by John Scott Russell of the wave of translation (also known as solitary wave or soliton). The 1872 paper of Boussinesq introduces the equations now known as the Boussinesq equations.The Boussinesq approximation for water waves takes into account the vertical structure of the horizontal and vertical flow velocity. This results in non-linear partial differential equations, called Boussinesq-type equations, which incorporate frequency dispersion (as opposite to the shallow water equations, which are not frequency-dispersive). In coastal engineering, Boussinesq-type equations are frequently used in computer models for the simulation of water waves in shallow seas and harbours.
While the Boussinesq approximation is applicable to fairly long waves – that is, when the wavelength is large compared to the water depth – the Stokes expansion is more appropriate for short waves (when the wavelength is of the same order as the water depth, or shorter).