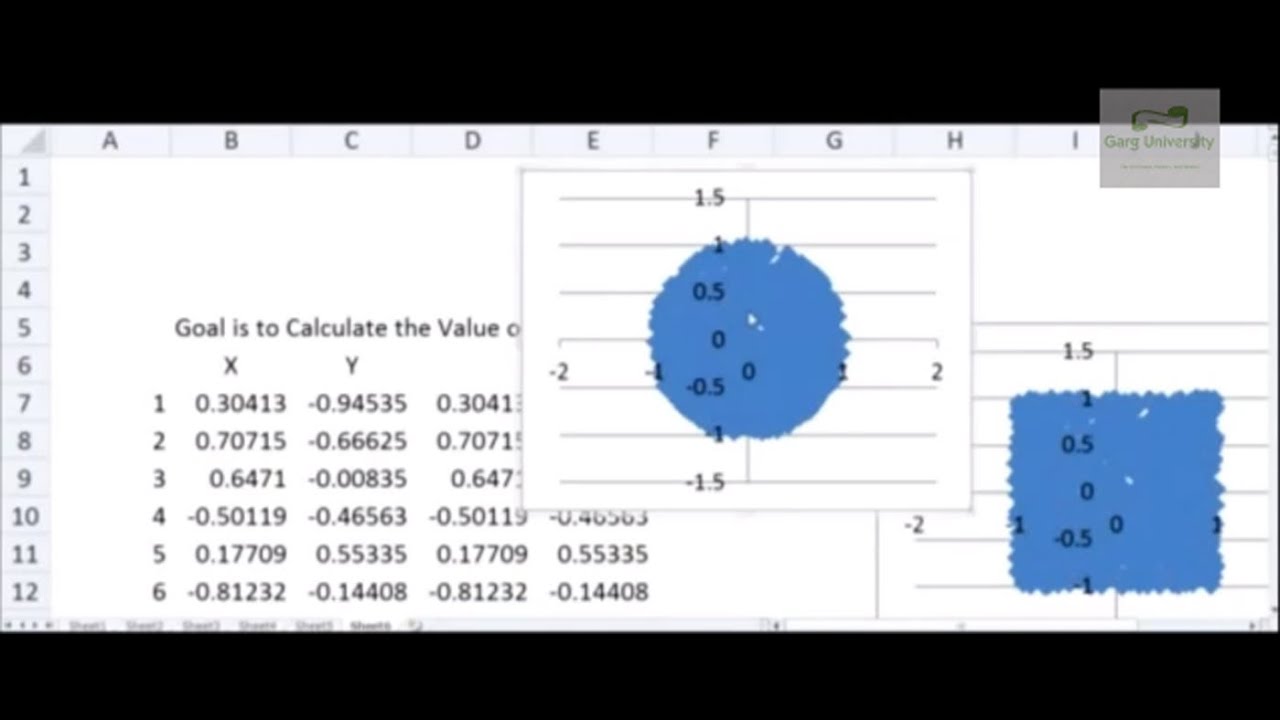
Simple Monte Carlo Simulation to Calculate Value of Pi using Excel

The number π (/paɪ/) is a mathematical constant that is the ratio of a circle's circumference to its diameter, and is approximately equal to 3.14159. It has been represented by the Greek letter "π" since the mid-18th century, though it is also sometimes written as pi. π is an irrational number, which means that it cannot be expressed exactly as a ratio of two integers (such as 22/7 or other fractions that are commonly used to approximate π); consequently, its decimal representation never ends and never settles into a permanent repeating pattern. The digits appear to be randomly distributed, although no proof of this has yet been discovered. π is a transcendental number -- a number that is not the root of any nonzero polynomial having rational coefficients. The transcendence of π implies that it is impossible to solve the ancient challenge of squaring the circle with a compass and straight-edge.

For thousands of years, mathematicians have attempted to extend their understanding of π, sometimes by computing its value to a high degree of accuracy. Before the 15th century, mathematicians such as Archimedes and Liu Hui used geometrical techniques, based on polygons, to estimate the value of π. Starting around the 15th century, new algorithms based on infinite series revolutionized the computation of π, and were used by mathematicians including Madhava of Sangamagrama, Isaac Newton, Leonhard Euler, Carl Friedrich Gauss, and Srinivasa Ramanujan.

[samvilla]

Thank you so much, this is so informative and clearly explained!

[punitasaxena]

Nicely explained. Should you not take the condition to be "less than or equal to 1". Because in simulation the condition has to include equality also