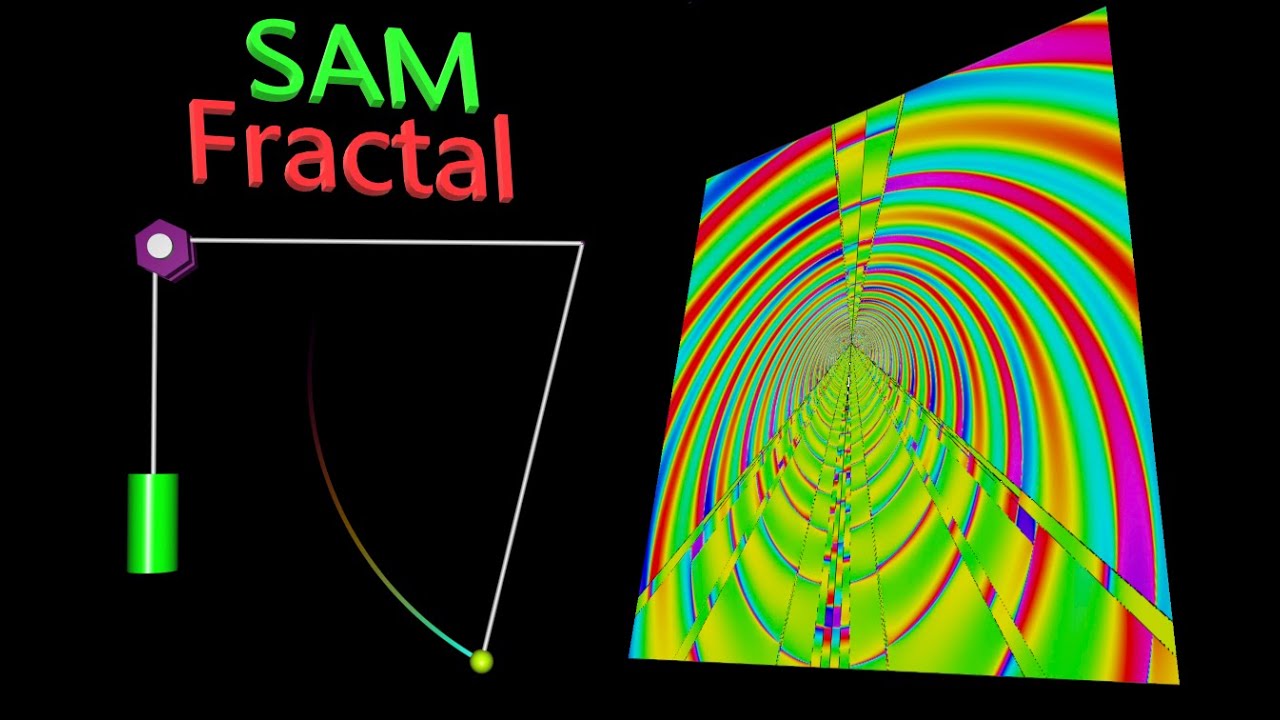
Fractals of the swinging Atwood's machine

Simulation of the swinging Atwood's machine (SAM) consisting of a weight with mass M that moves vertically connected with a rope to a swinging bob with mass m both being pulled by gravity. The dynamics of the system depends on the mass ratio M/m, with the ratios 1.75, 3 and 5 being examined in this video.

The video demonstrates the evolution of the bob angle with various initial positions and zero initial momenta. A canvas pixel at any initial position will be colored according to the bob's angle around the pivot after a set time. The time evolution of the full canvas is also displayed for the thee mass ratios.

The SAM is a Hamiltonian system which is integrable only for the mass ratio 3. Mass ratios different from 3 yield irregular orbits that will eventually approach the pivot. Upon approaching the pivot with an numerical energy error of more than 1e-4, the data are disregarded and depicted with black pixels on the canvas.

The simulations were performed using high order explicit symplectic integrators with the SAM being treated as a nonseparable Hamiltonian system with two degrees of freedom.

[benjaminlum]

I find it very interesting how for mass ratios of 1.75 and 5, there are very distinct radial lines where a slight change in initial angle results in a completely different ending region, whereas for mass ratio of 3 there is no sharp change, but 2 gradual changes that are vertical through the whole plane. Nothing immediately screams "resonance" to me here, so I'm perplexed at why only a ratio of 3 results in this behaviour, or which is the norm and which is the anomily. Love your smimulations though!

[Zymplectic]

Hamiltonian:
0.5*(p1^2 + p2^2 + (M*(p1*q2 - p2*q1)^2)/(m*(q1^2 + q2^2)))/(M + m) + M*g*(L0 + sqrt(q1^2 + q2^2)) + g*m*q2
where (q1, q2) are Cartesian coordinates. Cartesian coordinates are uncommon for this system, but yields roughly two to three times faster numerical integration when compared to the more common Hamiltonian using angle and radius (see wikipedia).

[black-king-]

Wow. What paper you based for doing the fractal?. Nice work!

[Spaceman]

I've never heard of that machine before