filmov
tv
🔶21 - Continuity and Discontinuity of a Function

Показать описание
🔶21 - Continuity and Discontinuity of a Function
In this video, we shall prove whether a function is continuous or not at a point a.
A function is continuous at a point a, if
a. f(a) is defined
b. the left hand limit = right hand limit
c. general limit of f(x) = f(a)
if even one condition is violented, the function is not continuous hence, discontinuous at a
The limit (L)of a function is what the function approaches, when a number x gets very close to a. The limit of a function does not need to be defined when x = a, the only interest is how the function is defined when x gets much closer to a.
L = lim f(x) as x approaches a.
for the limit of a function to exist, the left hand limit should be equal to the right hand limit. We shall solve tons of examples
00:00 - Continuity
02:30 - Discontinuity
06:33 - Question 1
10:40 - Question 2
13:52 - Question 3
18:24 - Question 4
Visit channel Playlist for more videos on Engineering mathematics, applied electricity and Basic Mechanics. Kindly support and Subscribe
Thank you
In this video, we shall prove whether a function is continuous or not at a point a.
A function is continuous at a point a, if
a. f(a) is defined
b. the left hand limit = right hand limit
c. general limit of f(x) = f(a)
if even one condition is violented, the function is not continuous hence, discontinuous at a
The limit (L)of a function is what the function approaches, when a number x gets very close to a. The limit of a function does not need to be defined when x = a, the only interest is how the function is defined when x gets much closer to a.
L = lim f(x) as x approaches a.
for the limit of a function to exist, the left hand limit should be equal to the right hand limit. We shall solve tons of examples
00:00 - Continuity
02:30 - Discontinuity
06:33 - Question 1
10:40 - Question 2
13:52 - Question 3
18:24 - Question 4
Visit channel Playlist for more videos on Engineering mathematics, applied electricity and Basic Mechanics. Kindly support and Subscribe
Thank you
🔶21 - Continuity and Discontinuity of a Function
#GeeklyHub What is Limit? Continuous and Discontinuous Functions, Limit Rules
Discontinuity: The Four Types of Discontinuities You Need to Know
Continuity on an Interval
Limits to define continuity
Continuous Function | Continuity of a Function 1
continuity and Discontinuity Q N 21
Continuity/Discontinuity of a function
Continuity and Discontinuity Examples
Continuity at a point | Limits and continuity | AP Calculus AB | Khan Academy
Piecewise Function Continuity Find A and B for Continuity and Discontinuity Q15 p53 MCV4U
Continuity and Discontinuity
Calculus - 1 Old Exams Questions on: ( Continuity and Discontinuity )
Issues of Human Development (Nature vs. Nurture,Continuity vs. Discontinuity & Stability vs. Cha...
Examples of Continuity and Discontinuity
Continuity and Discontinuity
Classify as Removable, Jump, or Infinite Discontinuity | Calculus: Limits and Continuity
Discontinuous Functions: Infinite vs. Removable Discontinuities
21. Explain why the function is discontinuous at the given number a. Sketch the graph of the
2nd Year Math, Ch 1 - Continuity of Functions Exercise 1.4 - 12th Class Maths
Continuity and Discontinuity of Functions
Continuity and Intermediate Value Theorem (Calculus 1)
Types of Discontinuity (Removable, Jump, Infinite) | Calculus: Limits and Continuity
Determining Continuity and Discontinuity on a Piece-wise Function (Type 2)
Комментарии
 0:22:14
0:22:14
 0:07:55
0:07:55
 0:05:09
0:05:09
 0:13:36
0:13:36
 0:11:14
0:11:14
 0:08:38
0:08:38
 0:02:34
0:02:34
 0:04:03
0:04:03
 0:25:53
0:25:53
 0:08:16
0:08:16
 0:05:47
0:05:47
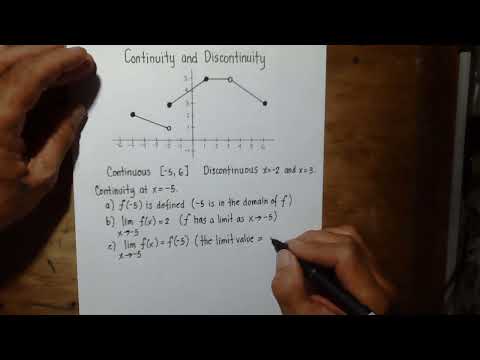 0:07:58
0:07:58
 0:07:21
0:07:21
 0:04:08
0:04:08
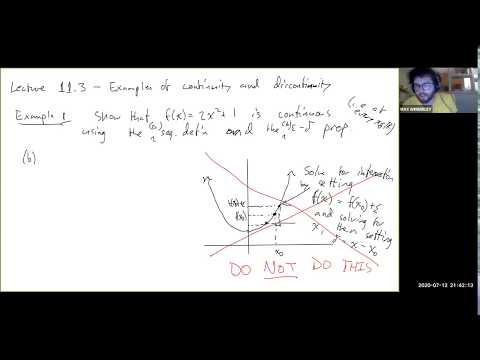 0:22:10
0:22:10
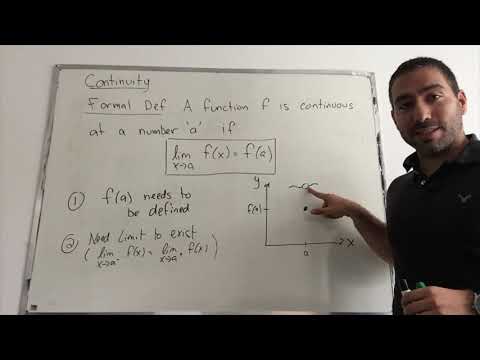 0:26:51
0:26:51
 0:16:01
0:16:01
 0:11:55
0:11:55
 0:02:51
0:02:51
 0:21:35
0:21:35
 0:05:32
0:05:32
 0:10:37
0:10:37
 0:11:45
0:11:45
 0:09:06
0:09:06