filmov
tv
Graphing Hyperbolas in Standard Form

Показать описание
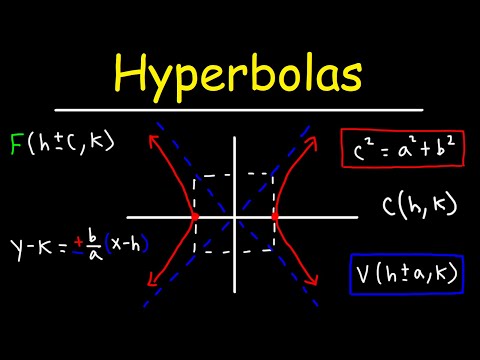
I introduce the basic structure of hyperbolas discussing how to locate the vertices, foci, transverse axis, conjugate axis, asymptotes, etc. I finish by working through multiple examples.
Hyperbolas - Conic Sections
Convert to a hyperbola to standard form to find foci, vertices, center and asymptotes
How to graph a hyperbola in standard form
Graphing a hyperbola in standard form
Graphing a hyperbola from an equation in standard form
Graphing Hyperbolas in Standard Form
Graphing a Hyperbola in Standard Form
How to Write a Hyperbola in Standard Form
Graphing Conic Sections Part 4: Hyperbolas
Graph hyperbola not in standard form
Learn how to graph a hyperbola
Schwanekamp Writing Hyperbolas in Standard form and Graph
Graphing a Hyperbola from Standard Form
write equation of hyperbola in standard form and graph it
How to write the equation of the hyperbola in the original form. grade 11 and grade 12 functions
Complete the square then graph and identify parts of an hyperbola
Graphing Hyperbolas From Standard Form
Graphing a hyperbola given its equation in general form
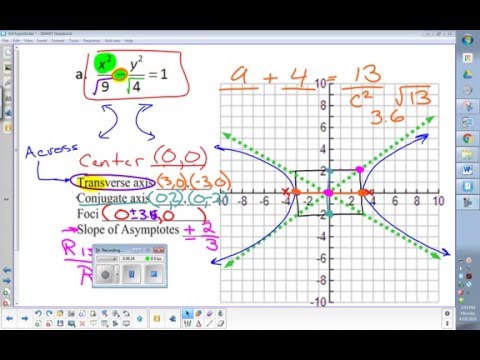
Graphing a hyperbola given its equation in standard form
Graphing Hyperbolas in Standard Form
Graphing Hyperbolas
Graphing Hyperbolas Given the Equation in Standard Form
Pre-Calculus Graphing Hyperbola
Conic Sections: The Hyperbola part 1 of 2
Комментарии
 0:34:50
0:34:50
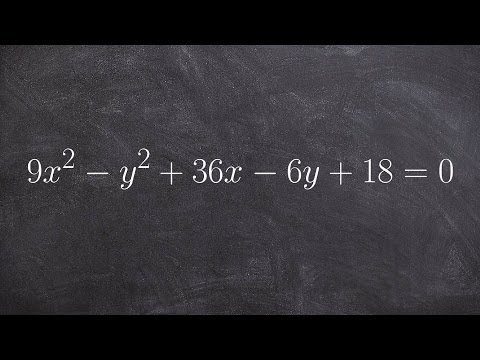 0:09:10
0:09:10
 0:05:08
0:05:08
 0:03:48
0:03:48
 0:05:47
0:05:47
 0:36:53
0:36:53
 0:09:26
0:09:26
 0:03:28
0:03:28
 0:05:57
0:05:57
 0:09:54
0:09:54
 0:09:29
0:09:29
 0:08:46
0:08:46
 0:01:00
0:01:00
 0:10:47
0:10:47
 0:07:00
0:07:00
 0:07:02
0:07:02
 0:07:26
0:07:26
 0:08:44
0:08:44
 0:07:08
0:07:08
 0:27:53
0:27:53
 0:08:27
0:08:27
 0:08:38
0:08:38
 0:06:16
0:06:16
 0:10:05
0:10:05