filmov
tv
Elliptic Curve Cryptography Overview
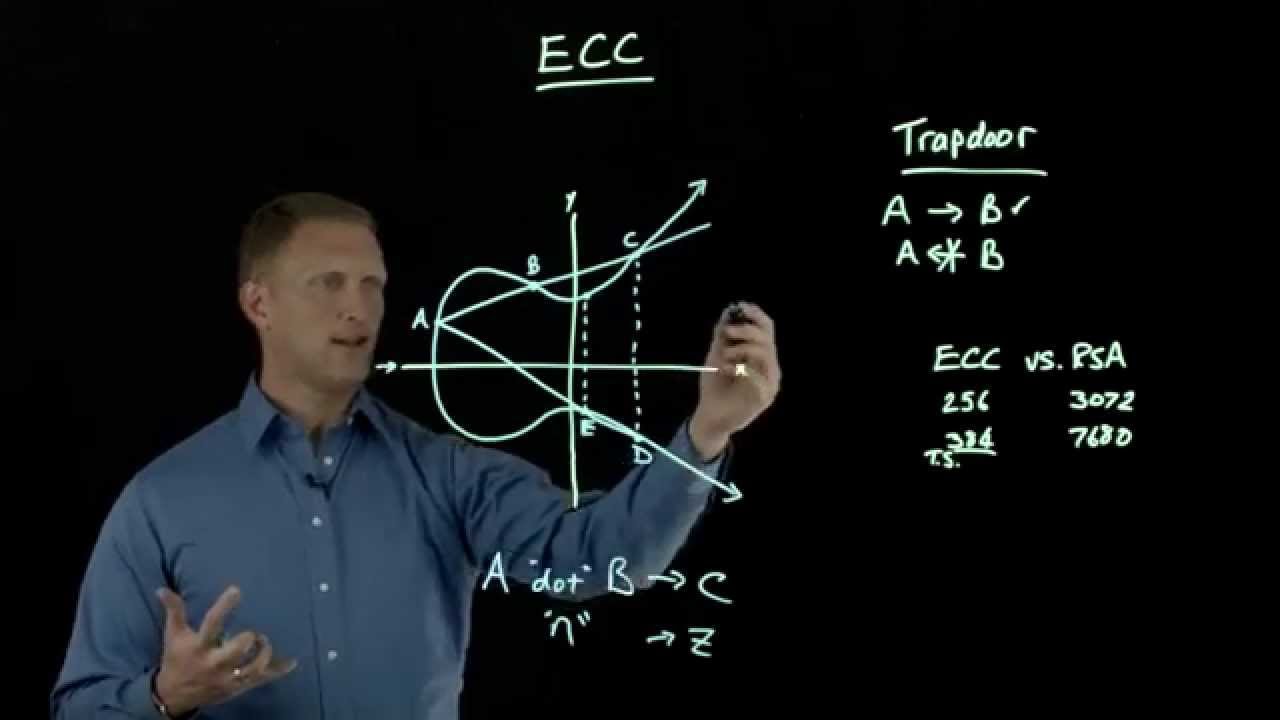
Показать описание
John Wagnon discusses the basics and benefits of Elliptic Curve Cryptography (ECC) in this episode of Lightboard Lessons.
Corrections:
02:24 As we all know, a prime number only has itself and 1 as factors. So, if you multiply two numbers together, the resultant number will at least have the two numbers you multiplied as factors…thus not making it prime. Technically speaking, the product of the two prime numbers in RSA is called a “semiprime” number because its only factors are 1, itself, and two prime numbers.
Corrections:
02:24 As we all know, a prime number only has itself and 1 as factors. So, if you multiply two numbers together, the resultant number will at least have the two numbers you multiplied as factors…thus not making it prime. Technically speaking, the product of the two prime numbers in RSA is called a “semiprime” number because its only factors are 1, itself, and two prime numbers.
Elliptic Curve Cryptography Overview
Elliptic Curves - Computerphile
Elliptic Curve Cryptography Tutorial - Understanding ECC through the Diffie-Hellman Key Exchange
13 Elliptic Curve Cryptography Overview
Elliptic Curve Cryptography in less than 5 minutes
Math Behind Bitcoin and Elliptic Curve Cryptography (Explained Simply)
Using Elliptic-Curve Cryptography (ECC) To Protect Websites - Part 1
Elliptic Curve Cryptography
PUBLIC KEY CRYPTOSYSTEMS
Elliptic Curve Cryptography Tutorial - An Introduction to Elliptic Curve Cryptography
The Basics of Elliptic Curve Cryptography (ECC)
Introduction to Elliptic Curve Cryptography
Elliptic Curve Cryptography | ECC in Cryptography and Network Security
3 Common Forms of Elliptic Curve Cryptography
Curves which make Bitcoin possible.
Elliptic Curve Cryptography: Points on Curves
Elliptic Curve and Quantum Cryptography - CompTIA Security+ SY0-401: 6.1
18 Diffie Hellman vs Elliptic Curve Cryptography
Elliptic Curve Cryptography for Developers - First Chapter Summary
Elliptic Curve Cryptography
Overview of Elliptic Curve Isogenies Based Public Key Cryptography Assumptions
Neutral Element in Elliptic Curve Cryptography: Exploring the Point at Infinity
Intro to Elliptic Curve Cryptography | ECC
Elliptic Curve Cryptography (EEC) - Nick Gonella
Комментарии
 0:11:29
0:11:29
 0:08:42
0:08:42
 0:11:34
0:11:34
 0:01:54
0:01:54
 0:04:44
0:04:44
 0:11:13
0:11:13
 0:07:22
0:07:22
 0:17:10
0:17:10
 0:05:02
0:05:02
 0:09:34
0:09:34
 0:12:16
0:12:16
 0:21:40
0:21:40
 0:14:32
0:14:32
 0:04:02
0:04:02
 0:07:45
0:07:45
 0:11:14
0:11:14
 0:02:27
0:02:27
 0:02:51
0:02:51
 0:03:46
0:03:46
 0:15:18
0:15:18
 0:43:22
0:43:22
 0:03:16
0:03:16
 0:09:29
0:09:29
 0:28:17
0:28:17