filmov
tv
Lecture 15: Corner layers

Показать описание
So far we've been discussing boundary layers. But other kinds of layers (meaning regions of rapid variation in a function or its derivatives) can sometimes occur on the interior of a region. In this lecture and the next we'll look at examples of such interior layers. Our first example is a "corner layer" in which the solution y undergoes a rapid change in its derivative, creating a kink or "corner" that becomes increasingly sharp as the small parameter epsilon tends to zero. In the course of the analysis, we'll run into special functions called "parabolic cylinder functions" -- these arise in quantum mechanics, fluid dynamics, and many other parts of science and engineering.
Lecture 15: Corner layers
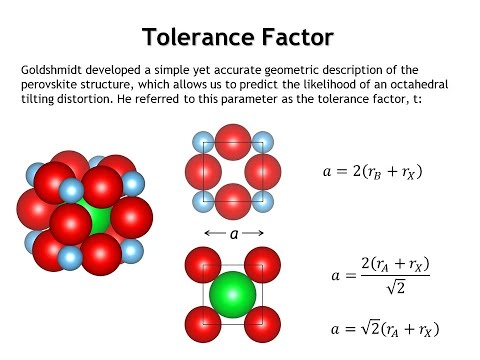
Lecture 15 Ternary and Quaternary Structures
IIT Bombay Lecture Hall | IIT Bombay Motivation | #shorts #ytshorts #iit
Lecture 15 (EECS4404E) - CNNs (Part II) - LeNet, AlexNet, Inception, and ResNet
Can you solve this 150 years old puzzle? #shorts
The 15 Puzzle, The Rubik's Cube, and Lights Out (Algebra 1: Lecture 17 Video 1)
Geology 15 (Faults, Folds, and Joints)
Lecture 15: Clustering
Lecture 14: Location and thickness of boundary layers
CS480/680 Lecture 16: Convolutional neural networks
Lecture 15
Gas Dynamics: Lecture 15: Numerical Techniques for Supersonic Flow, Elements of Hypersonic Flow
Engineering Geology And Geotechnics - Lecture 15
Lecture 15 | Efficient Methods and Hardware for Deep Learning
Lecture 15 - Semantic Segmentation
CEEN 341 - Lecture 15 - Elastic Settlement and Primary Consolidation Settlement
Geophysical Fluid Dynamics (ESP-GFD) Lecture 15
Structure Of The Earth | The Dr. Binocs Show | Educational Videos For Kids
Daniel Urban Kiley Lecture: James Corner
Lecture 15 Case Study of Deep Generative Models: Text Generation
How to Remember Every Muscle in the Head and Face | Corporis
*ENGR 102 Lecture 15: Review of In-Class Exercise 5 (2024.02.28)
HetSys Course: Lecture 15: Collaborative Computing (Fall 2022)
Lecture 16 - Working With Layers - Art 186 - Spring 2022
Комментарии
 1:11:24
1:11:24
 0:23:06
0:23:06
 0:00:12
0:00:12
 0:49:27
0:49:27
 0:00:57
0:00:57
 0:26:13
0:26:13
 1:11:32
1:11:32
 1:17:38
1:17:38
 1:19:55
1:19:55
 1:15:18
1:15:18
 0:32:44
0:32:44
 1:17:59
1:17:59
 2:14:53
2:14:53
 1:16:52
1:16:52
 0:39:25
0:39:25
 0:57:19
0:57:19
 1:41:12
1:41:12
 0:03:13
0:03:13
 1:44:51
1:44:51
 1:05:20
1:05:20
 0:13:16
0:13:16
 0:06:35
0:06:35
 1:19:33
1:19:33
 0:51:39
0:51:39