filmov
tv
Complex Analysis: Inverse Laplace Transform 1/sqrt(s)
Показать описание
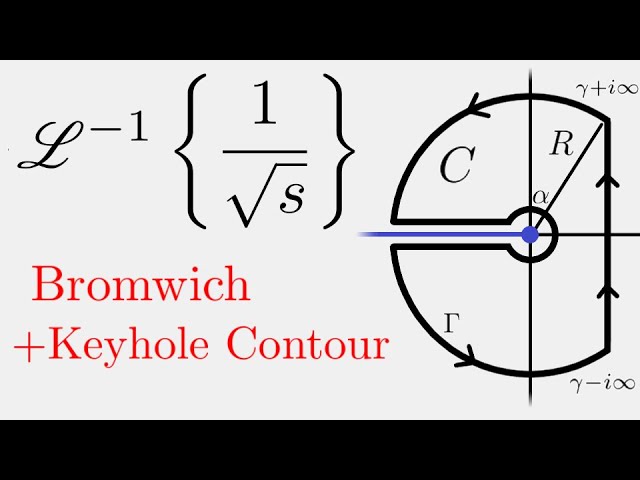
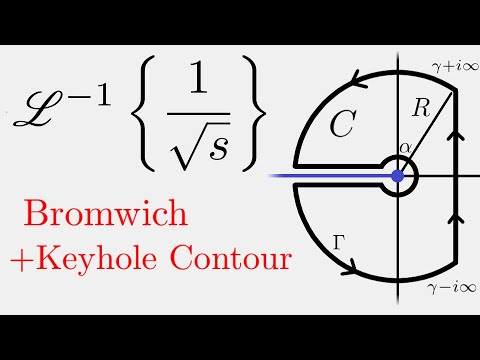
Today, we evaluate the inverse Laplace transform of 1/sqrt(s) using a combination of the Bromwich and keyhole contour.
Complex Analysis L13: Bromwich Integrals and the Inverse Laplace Transform
Inverse laplace Transformation Browmwich Contours
Inverse Laplace Transform Using The Residue Theorem
Complex Analysis: Inverse Laplace Transform 1/sqrt(s)
How to solve differential equations
PG2 Complex Analysis -- Inverse Laplace Transfrom Bromwich Integral
Inverse Laplace Transform
ME565 Lecture 6: Inverse Laplace Transform and the Bromwich Integral
Crank Nicholson method in numerical methods 🔥 #secondyearengineeringmaths
The inverse Laplace Transform | Differential Equations | LetThereBeMath |
me75 - lec22 - Inverse Laplace Transform
Complex Analysis L07: Analytic Functions Solve Laplace's Equation
me75 - lec23 - Inverse Laplace Transform
Inverse Laplace transform by contour integration
Inverse Laplace Transform Example
The Inverse Laplace Transform
Inverse Laplace Transform the proper way, using Residue theorem.
Class 03 Laplace Transform: Complex Numbers
Table of Laplace transform
Inverse Laplace Transform of 1/(s - 4) #shorts
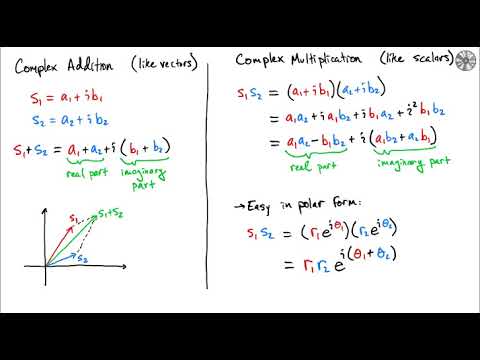
Why care about complex analysis? | Essence of complex analysis #1
How to Find the Inverse Laplace Transform of 1/s^4 | #shorts
Laplace Transform of e^(4t) #shorts
Laplace transform l Formulae l Inverse Laplace transform
Комментарии
 0:50:33
0:50:33
 0:12:05
0:12:05
 0:04:24
0:04:24
 0:16:36
0:16:36
 0:00:46
0:00:46
 0:19:17
0:19:17
 0:11:29
0:11:29
 0:48:49
0:48:49
 0:04:36
0:04:36
 0:13:40
0:13:40
 0:32:08
0:32:08
 0:41:15
0:41:15
 0:12:54
0:12:54
 0:52:11
0:52:11
 0:09:49
0:09:49
 0:48:36
0:48:36
 0:14:34
0:14:34
 0:09:29
0:09:29
 0:00:05
0:00:05
 0:00:19
0:00:19
 0:03:55
0:03:55
 0:00:19
0:00:19
 0:00:17
0:00:17
 0:00:13
0:00:13