filmov
tv
How to find the square root of a complex number

Показать описание
-----------------------------
-----------------------------
#math #algebra #mathbasics
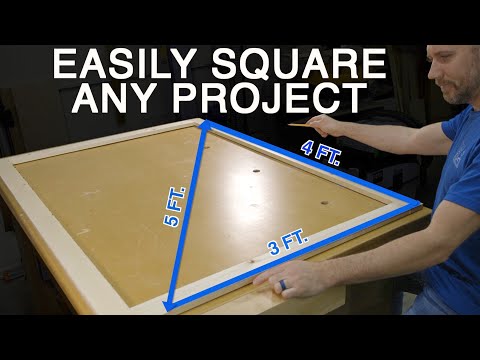
3-4-5 Triangle Method For Finding Square
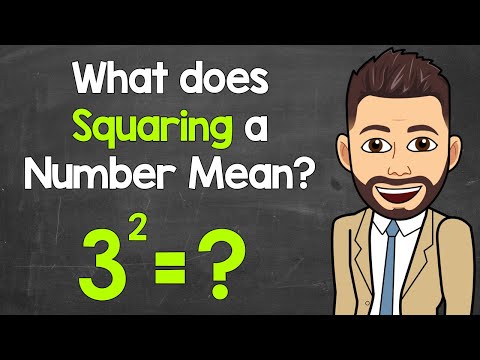
How to Square a Number | What Does Squaring a Number Mean? | Exponents | Math with Mr. J
Find Square of Any Number in 2 Seconds | Vedic Maths Tricks For Fast Calculation #vedicmaths #shorts
How to Find the Area of a Square | Math with Mr. J
How to square any numbers in your head - fast mental math trick
Learn to Square any 2 digit number I Math Tricks and Tips
Quick Square Pattern Trick|#shorts#math#youtubeshorts#ytshorts #vedicmathstricksforfastcalculation
Shortcut to find square of any number | Best trick on the internet #howtofindsquare #square
Square Root Math Hack
Short Tricks on Square Root in 5 Seconds #shorttricks #shorttricksmaths #shorts
#MATHS TRICK OF FINDING #SQUARE MORE THAN 100 - #tricks #shorts
1-1000 SQUARE in 2 SECONDS| Super-Fast Square TRICK |Vedic Maths TRICKS |SHORTCUT| By Chandan Venna
1-1000 Square in 5 Seconds | Square Trick | Vedic Maths | Vedic Maths Tricks
1-1000 Square in 5 Seconds | Square Trick | Vedic Maths | Vedic Maths Tricks
Area of square | How to find area of the square #shorts
How to Approximate Square Root of a Number
How To Find The Square Root of Large Numbers Mentally
How To Calculate Square Roots - Numerals That Changed Math Forever
How find the Square Root of a Perfect Square Number
Square root in 3 seconds - math trick
How to find Square Root of Perfect Square Number? | Best Square Root Tricks | Math Tricks | Letstute
How to do Square-up Diagonal Calculations without Formulas | Construction Master 5
Square Shortcut Trick🔥| Square 21-30 Tricks #math #shorts #shortvideo #short #trending #vedicmaths...
Square Root | How to find square root | How to calculate square root #shorts #squaretricks
Комментарии
 0:05:49
0:05:49
 0:03:12
0:03:12
 0:00:52
0:00:52
 0:02:48
0:02:48
 0:05:25
0:05:25
 0:07:10
0:07:10
 0:00:34
0:00:34
 0:04:20
0:04:20
 0:00:23
0:00:23
 0:00:45
0:00:45
 0:00:28
0:00:28
 0:15:05
0:15:05
 0:00:58
0:00:58
 0:14:07
0:14:07
 0:00:30
0:00:30
 0:00:52
0:00:52
 0:12:37
0:12:37
 0:10:16
0:10:16
 0:00:59
0:00:59
 0:09:46
0:09:46
 0:04:57
0:04:57
 0:00:53
0:00:53
 0:00:19
0:00:19
 0:01:00
0:01:00