filmov
tv
Complex Hyperbolic Trigonometry Proofs: sin(iz) = isinh(z) and sinh(iz) = isin(z)
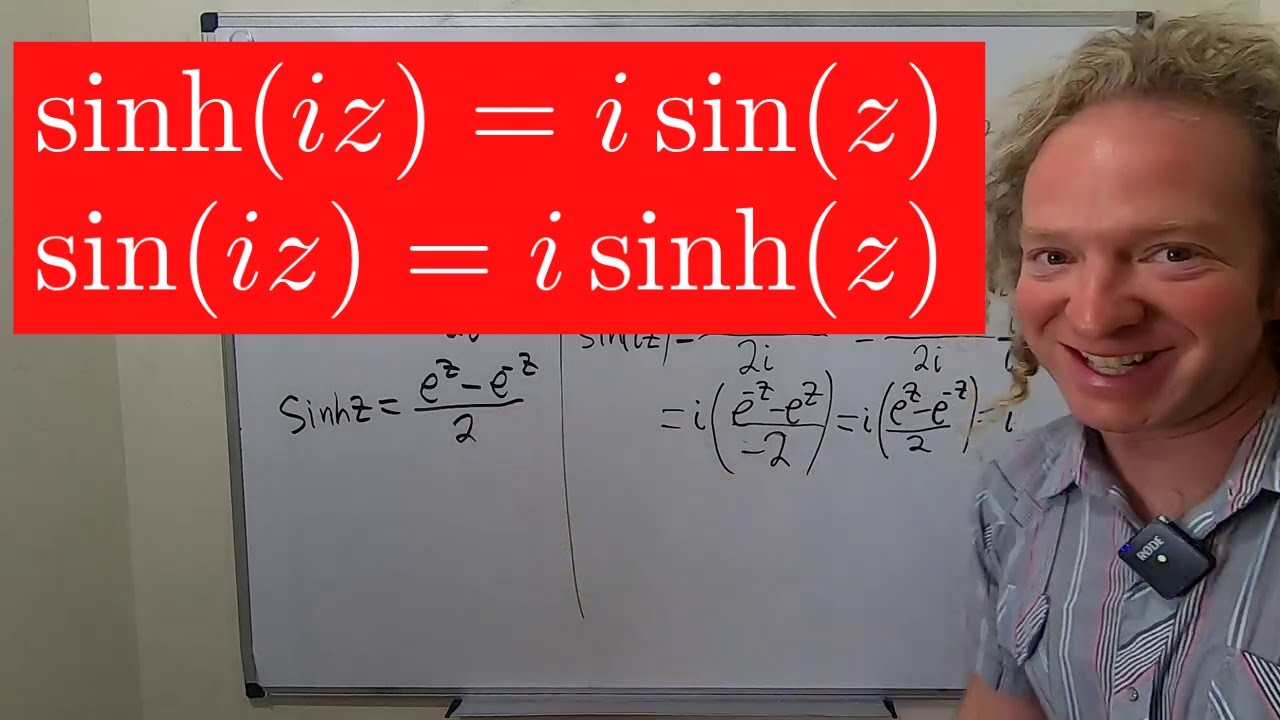
Показать описание
In this video I prove some cool identities relating the complex sine and hyperbolic sine functions.
I hope this video helps someone.
I hope this video helps someone.
Complex Hyperbolic Trigonometry Proofs: sin(iz) = isinh(z) and sinh(iz) = isin(z)
Complex Hyperbolic Trigonometry Proofs: cos(iz) = cosh(z) and cosh(iz) = cos(z)
Complex definitions of sine and cosine
Hyperbolic Sin(Z) and Cos(Z) part 1#Complex analysis
Proof of Complex Trigonometric Functions sin(iz) = isinh(z) & cos(iz) = cosh(z)
relation between trigonometric and hyperbolic function
Complex Hyperbolic Trig Identies Sihn(z) and Cosh(z)
Complex trigonometric functions
Differentiating Trigonometric Identities Cos z and Sin z for Complex z
Hyperbolic Trigonometric Proof
VO Week 26 3 Proving hyperbolic identities
Hyperbolic Identities
Complex Analysis Exponential, Trigonometric and Hyperbolic Functions Example 3
Complex Analysis Exponential, Trigonometric and Hyperbolic Functions Example 1
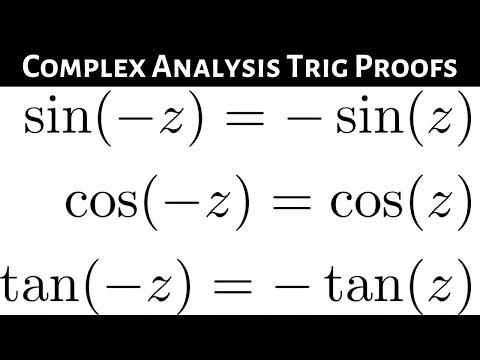
Proof that sin(-z) = -sin(z), cos(-z) = cos(z), and tan(-z) = -tan(z) for Complex Values of z
Prove that Sin ix=i Sin hx
#mathcompulsory | to proof trigonometry eqation in hyperbolic functions | proof for Cosz and Sinz |
Complex Analysis Exponential, Trigonometric and Hyperbolic Functions Example 2
Trigonometric function of a complex number || sin z
PROOF; sin(ix)=i sin(hx).
Complex Analysis cosh z
Complex hyperbolic functions
Complex Analysis 05: Trigonometric and Hyperbolic Equations
Prove that Cos ix= Coshx
Комментарии
 0:06:20
0:06:20
 0:04:24
0:04:24
 0:05:16
0:05:16
 0:07:48
0:07:48
 0:02:47
0:02:47
 0:07:13
0:07:13
 0:08:31
0:08:31
 0:09:01
0:09:01
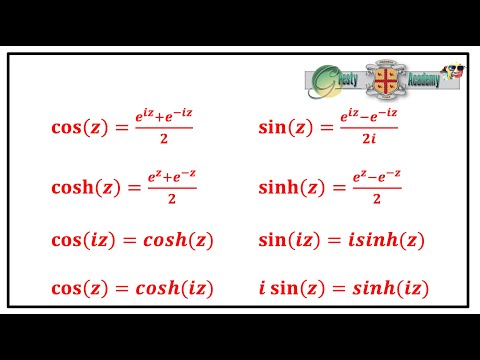 0:11:18
0:11:18
 0:07:09
0:07:09
 0:05:45
0:05:45
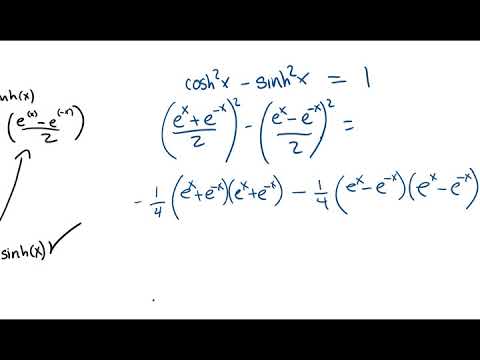 0:08:01
0:08:01
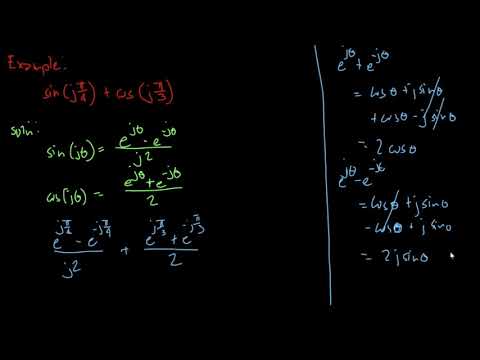 0:08:58
0:08:58
 0:06:43
0:06:43
 0:05:52
0:05:52
 0:01:01
0:01:01
 0:19:08
0:19:08
 0:06:44
0:06:44
 0:02:09
0:02:09
 0:03:24
0:03:24
 0:05:06
0:05:06
 0:05:02
0:05:02
 0:13:33
0:13:33
 0:00:57
0:00:57