filmov
tv
Given Terminal Point on Unit Circle, find six Trigonometric Function Values
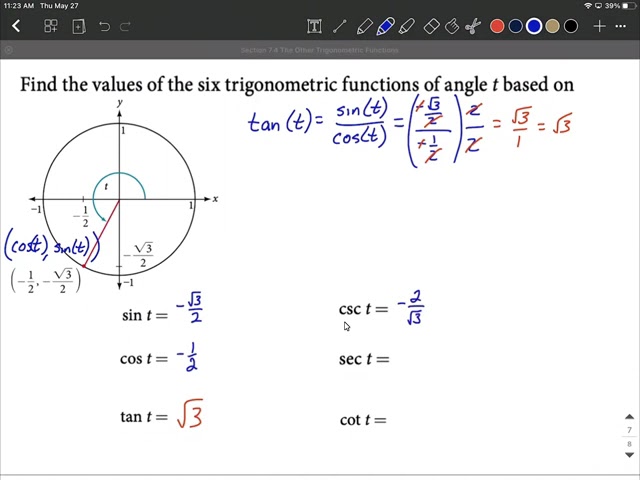
Показать описание
In this example, we are given a terminal point on the unit circle and must find the six trigonometric function values that go along with the corresponding angle. We can pick out cosine and sine based on the ordered pair that is given (cos(t),sin(t)). Then we use a quotient identity to find tangent as it is sine over cosine tan(t)=sin(t)/cos(t). After we have sine, cosine, and tangent, we use the reciprocal identities to find cosecant csc(t), secant sec(t), and cotangent cot(t).
 0:06:28
0:06:28
 0:06:58
0:06:58
 0:07:05
0:07:05
 0:04:19
0:04:19
 0:04:08
0:04:08
 0:01:51
0:01:51
 0:00:34
0:00:34
 0:05:35
0:05:35
 0:09:54
0:09:54
 0:04:44
0:04:44
 0:08:12
0:08:12
 0:31:56
0:31:56
 0:06:15
0:06:15
 0:03:46
0:03:46
 0:05:26
0:05:26
 0:01:56
0:01:56
 0:04:46
0:04:46
 0:13:39
0:13:39
 0:05:05
0:05:05
 0:07:42
0:07:42
 0:10:48
0:10:48
 0:02:21
0:02:21
 0:07:18
0:07:18
 0:01:00
0:01:00