Calc 1, Lec 17A: Newton's Method, Sqrt(2) Existence & Irrationality, Product Rule w/ Infinitesimals

(0:00) This week (Lectures 17, 18, and 19) is the core of differential calculus (& lecture plan).
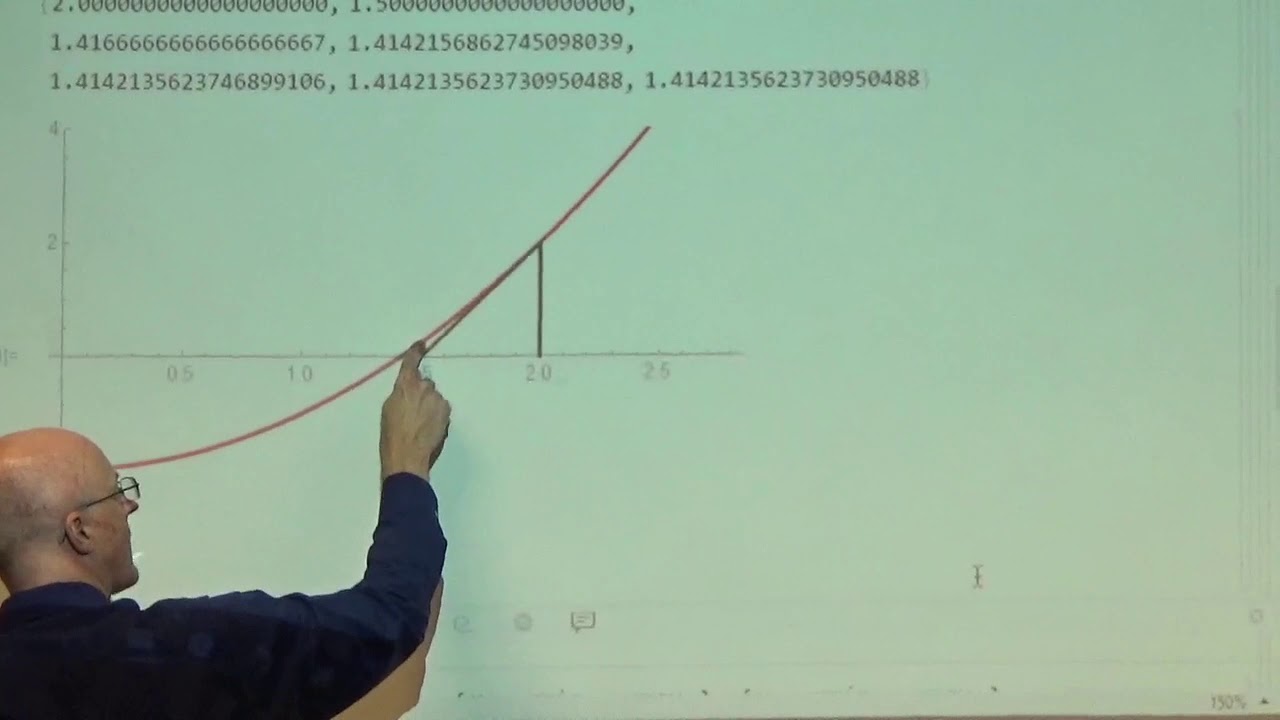
(0:52) Newton's method review (solve for the x-intercept of the tangent line equation). Estimate Sqrt(2) by estimating the positive root of f(x) = x^2 - 2.
(6:27) NewtonPlot code in Mathematica to show the successive approximations (iterates) and the graph. Also zoom in on the root by using Manipulate.
(9:55) Does Sqrt(2) exist? It's irrational, so its digits go on forever and ever without a repeating pattern. So how is it possible to "know" it by squaring its decimal representation?
(12:11) If it does exist, it is irrational. Go through the beautiful proof that Sqrt(2) is irrational. It's a proof by contradiction. Rumor has it that a Pythagorean was killed for this discovery.
(15:24) Proving its existence requires the Completeness Axiom and is done in Real Analysis.
(17:21) Derive the Product Rule with Infinitesimal Calculus.
(24:22) Memorizing the Product Rule as "left d(right) plus right d(left)", as done in 3Blue1Brown's "Essence of Calculus" series.
(25:18) Example: Differentiate f(x) = x*sin(x) and check with Mathematica, both symbolically and with graphs.
(28:28) Revenue from sales of skateboards when the demand function value and derivative value are knowns. Use the Product Rule. The derivative is positive, so revenue (incoming money) will increase if the price is raised.