filmov
tv
Integrate (sinx)^5 (cosx)^3 by u-substitution
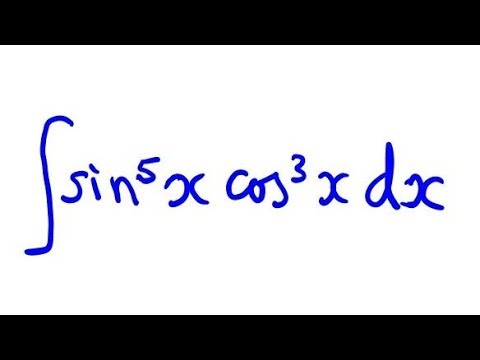
Показать описание
How to integrate a product of powers of sin and cos where one of the powers is odd
Integrate (sinx)^5 (cosx)^3 by u-substitution
Integral of sin(x)/(5 + 3 cos x) dx
Integrating sinx*(cosx)^3 using a simple guess about the 'chain rule backwards'.
Integral sin(x)*sqrt(cos(x), informally (chain rule backwards), and formally (using u-substitution).
Integral cosx*(sin(x))^5 informal method (chain rule backwards) and u-substitution with u=sin(x).
Trigonometric Integrals
How REAL Men Integrate Functions
Integrate ( sin x )^5 by u-substitution
Integral of cos x/(5 - 3 sin x) dx
Integration with u-substitution the Integral of sin(x)/(3 + cos(x))^2
Integral of cos(x)/sin^5(x) (substitution)
Integration of product of trig functions by substitution sinx sqrt (cosx + 1)
Integration of sin^5(x/3)cos(x/3)dx
Integral of sin^5(x)
evaluate `intdx/(3+5cosx)`
Integrate cos(x)*sin(x)^3 using the U substitution method | Integral Of Trigonometric Functions
∫sech(x) dx. MIT Integration Bee 2017, Question 3, Qualifying Exam. Method 1: substitute u = eˣ
∫cos(x).cos(sin(x)).cos(sin(sin(x))) dx. MIT Integration Bee 2019 (Question 7, Qualifying Exam).
Use substitution to find the indefinite integral of x^2 cos(x^3 + 5)dx
Integral (sinx)^3 cosx dx
Integral of sin^3(x)cos^5(x) - Integral example
Integral of sinxcosx | #shorts #integral #youtubeshorts
IIT Bombay CSE 😍 #shorts #iit #iitbombay
Evaluate the Integral cos^3 x sin x dx with u substitution u = cos x
Комментарии
 0:00:35
0:00:35
 0:02:13
0:02:13
 0:05:58
0:05:58
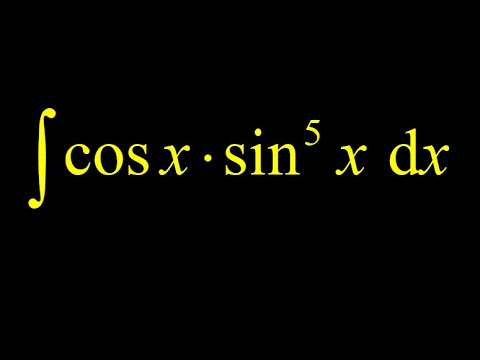 0:02:41
0:02:41
 0:31:29
0:31:29
 0:00:35
0:00:35
 0:07:02
0:07:02
 0:00:53
0:00:53
 0:02:06
0:02:06
 0:01:23
0:01:23
 0:02:05
0:02:05
 0:02:58
0:02:58
 0:04:38
0:04:38
 0:08:50
0:08:50
 0:02:16
0:02:16
 0:00:36
0:00:36
 0:01:00
0:01:00
 0:01:25
0:01:25
 0:01:25
0:01:25
 0:02:32
0:02:32
 0:00:22
0:00:22
 0:00:11
0:00:11
 0:02:46
0:02:46