filmov
tv
25.1 Force is the Derivative of Potential

Показать описание
MIT 8.01 Classical Mechanics, Fall 2016
Instructor: Dr. Peter Dourmashkin
License: Creative Commons BY-NC-SA
Instructor: Dr. Peter Dourmashkin
License: Creative Commons BY-NC-SA
25 1 Force is the Derivative of Potential
What is a derivative? An example from physics.
Find Derivative of Composite Function with Prime Notation Chain Rule
5 simple unsolvable equations
Finding the Derivative of a Polynomial Function | Intro to Calculus #shorts #math #maths
Derivative of a function using the Quotient Rule
Vector calculus 1. Gradient and Directional derivative 1.
Calculus - HOW TO: Recognize Limit as Derivative (Moderate Level, Part 1)
Transformer chapter 19 class 12 new physics book | Transformer formula derivation | Types of transfo
Derivative at a point using the definition of derivatives
Visual physics derivation! (Centripetal acceleration)
Q4 Rate of Change of Pollution in 16 Years Derivative Applications AP Calculus
Find the Derivative of the Vector Valued Function Calculus
Find the Composite function whose derivative is given Calculus TIPS
Lecture 25: Frechet Derivative
100 calculus derivatives (ultimate derivative tutorial)
w = re^-r, find the derivative
Maximum Height and Velocity h(t) = -5t^2 + 30t + 1 Derivative Application
the simple derivative in physics.wmv
Work energy principle from the derivative of kinetic energy. #physics #classicalmechanics
SIMPLE IDENTITY! For Derivative of INVERSE TAN X
Physics 68 Lagrangian Mechanics (6 of 25) Simple Harmonic Motion: Method 1
📚 How to find the maximum value of a directional derivative in 2D (Question 2)
Concavity and Second Derivative Test
Комментарии
 0:03:59
0:03:59
 0:22:42
0:22:42
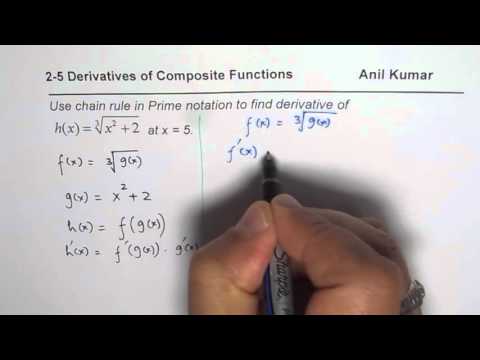 0:05:21
0:05:21
 0:00:50
0:00:50
 0:01:01
0:01:01
 0:12:27
0:12:27
 0:12:13
0:12:13
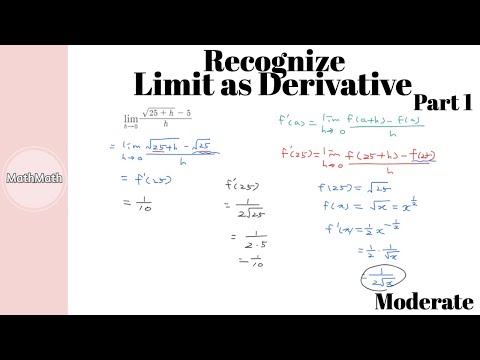 0:12:20
0:12:20
 0:25:23
0:25:23
 0:08:29
0:08:29
 0:08:47
0:08:47
 0:03:33
0:03:33
 0:02:05
0:02:05
 0:05:07
0:05:07
 0:15:29
0:15:29
 6:38:13
6:38:13
 0:01:03
0:01:03
 0:05:56
0:05:56
 0:10:06
0:10:06
 0:01:01
0:01:01
 1:48:34
1:48:34
 0:09:05
0:09:05
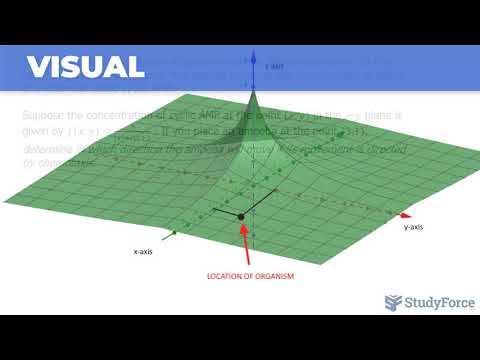 0:02:42
0:02:42
 0:29:31
0:29:31