Manifolds #7: Cotangent Space

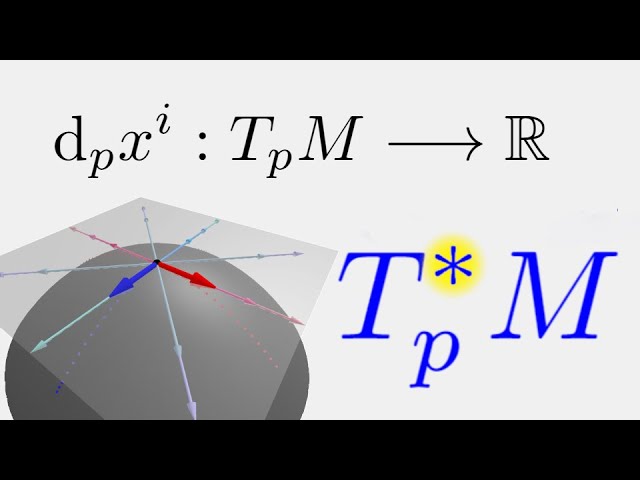
Today, we define the cotangent vector space at a point on a manifold and construct the dual basis by using the gradient operator.

[anshuagrawal]

Amazing!!! Could you please continue posting more videos on Manifolds? 🙏🙏🙏

[Amplituhedron]

Great series, are you going to discuss Pullback and Pushforward ? Seems you already discuss it without explicitly mentioning it ?

[Czeckie]

definitely interested in the construction of bundles. One thing that always bugs me is that I don't understand the point of these covectors. We already have the tangent map sending tangent vectors to tangent vectors, differential is then just a special case of this. And I don't see the utility of introducing new objects for it. I feel like I am missing something. Maybe the killer app is somehow in the next step, when we consider 1-forms as sections of the cotangent bundle?

[wesroberts]

This is great stuff! I work in quantum geometry, and I'm finding more and more that I really need to go back and learn these concepts formally. Your videos have been perfect for getting my feet under me. Do you happen to recommend any textbooks that are in the same spirit as your lectures? Thanks!

[jewbaby]

Excellent videos ^_^ May I ask what book if any this material comes from? Your notation looks like that of Frederic Schuller’s GR course at the international winter school and I’ve always wanted to get a copy of the text if it exists. Thanks for sharing!

[luaiderar]

I think I saw you outside melbourne central. Apparently you're my friends calc 1 tutor at unimelb lmaoo.

[aritelramos]

Queremos sabes de vos! Dónde estas? Como estás? 🧉🫂🇦🇷

[juniorcyans]

Alright, now I’m sure that last time you did use a carrot 🥕 for the mic!

[tulliolevicivita]

Are you preparing a curvature lesson??