filmov
tv
Volume of a Cone - An Unusual Proof
Показать описание
We look at an interesting derivation of the formula for the volume of a (right circular) cone. Adapted from the proof in this article:
Pargeter, A. (1976). The Volume of a Cone. The Mathematical Gazette, 60(413), 203-203. doi:10.2307/3617479
00:00 Intro
00:11 Setup
00:56 Idea of the proof
03:05 Proof
Pargeter, A. (1976). The Volume of a Cone. The Mathematical Gazette, 60(413), 203-203. doi:10.2307/3617479
00:00 Intro
00:11 Setup
00:56 Idea of the proof
03:05 Proof
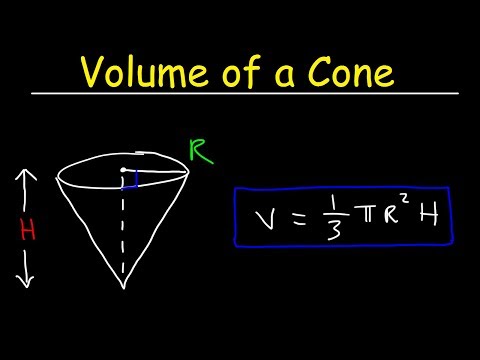
Volume of a Cone
Volume of a Cone | Math with Mr. J
Volume of a Cone | MathHelp.com
Volume of a cone | Perimeter, area, and volume | Geometry | Khan Academy
Why is the volume of a cone 1/3*pi*r^2*h? Here's a proof with the disc method! Calculus basics
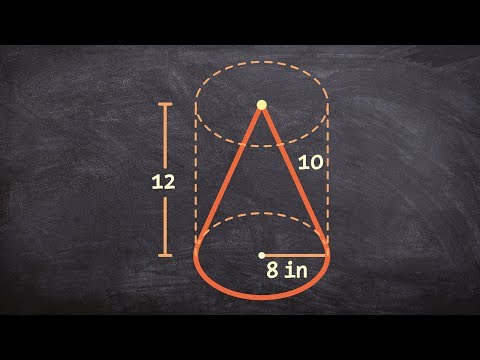
How to find the VOLUME OF A CONE
GCSE Maths - How to find the Volumes of Cones and Pyramids #113
Cylinder, Cone, and Sphere Volume
MEASUREMENTS | PERIMETER, SURFACE AREA & VOLUME | GRADE 10 & 11
Volume and Surface Area of a Cone & Lateral Area Formula- Basic Geometry
Prisms and Pyramids [ACT 3]: How Many Cones Does It Take To Fill a Cylinder?
Learn how to determine the volume of a cone
Lesson 8.3 Volume of a Cone
Find the Volume of Any Shape Using Calculus
Volume of a Cone - VividMath.com
How to Find the Volume of a Cone
A curious proof of cone's volume #SoME1 #SummerofMathExposition #3blue1Brown #geometry #cone
Easy math. Deriving the formula for the volume of a cone #ibmath #igcse #ibexam #math #ipmath
Volume of a Cone and Pyramid - How to Find (Formula)
Math Antics - Volume
Visualizing the Volume of a Sphere Formula | Deriving the Algebraic Formula With Animations
GCSE Maths - How to Find the Volume of a Frustum #114
Conical Volume (1 of 2: Derivation of the Volume of a Cone through Solids of Revolution)
Mensuration -Volume of the Cone
Комментарии
 0:01:32
0:01:32
 0:09:59
0:09:59
 0:02:44
0:02:44
 0:05:44
0:05:44
 0:07:10
0:07:10
 0:02:27
0:02:27
 0:02:31
0:02:31
 0:02:32
0:02:32
 0:58:08
0:58:08
 0:13:47
0:13:47
 0:00:36
0:00:36
 0:03:54
0:03:54
 0:07:31
0:07:31
 0:14:41
0:14:41
 0:02:19
0:02:19
 0:03:35
0:03:35
 0:08:09
0:08:09
 0:00:48
0:00:48
 0:03:00
0:03:00
 0:12:36
0:12:36
 0:03:12
0:03:12
 0:03:52
0:03:52
 0:08:36
0:08:36
 0:03:03
0:03:03