filmov
tv
derivative of hyperbolic functions examples with solutions (part-2)#derivatives#calculus#math#cosh2x
Показать описание
#derivatives
#differentiation#sinhx#coshx#tanhx#sechx#cosechx#cothx#sinh3x#cosh2x#tanhx^2
In perfect learning hub this video tutorial we will explain derivative of hyperbolic functions and how to find derivatives of hyperbolic trig functions examples and solutions.
Hyperbolic functions are analogs of the ordinary trigonometric functions defined for the hyperbola rather than on the circle.
Formulas for hyperbolic functions are:
Sinhx= [e^(x) – e^(-x)]/2 ,
Coshx= [e^(x) + e^(-x)]/2 ,
tanhx= [e^(x) – e^(-x)]/[e^(x) + e^(-x)] ,
Cosechx= 2/[e^(x) – e^(-x)] ,
Sechx= 2/[e^(x) + e^(-x)] ,
Cothx= [e^(x) + e^(-x)]/[e^(x) – e^(-x)] , here x is an element of set of real numbers.
Basically this hyperbolic functions derivatives in Hindi and urdu is especially for the calculus,mathematics students of class 12, fsc, ics, csbe,nscrt. We try to focus on derivatives class 12 math and explained chapter 2 completely. And also for the university students who are studying calculus, multivariable calculus, business mathematics etc.
So according to this video we can differentiate our questions by using derivative rules or formulas of hyperbolic functions.
derivative rules of hyperbolic functions are:
• d [ Sin(h(x)) ] / dx = [ Cos(h(x)) ] . d(x)/d(x) here x is an angle and dx/dx represents angle’s derivative with respect to x.
because we know:
d [ Sin(h(x)) ] / dx = d[(e^(x) – e^(-x))/2] /dx
= 1/2. [(e^(x) – e^(-x)(-1)]
= 1/2. [(e^(x) + e^(-x)]
= [Cos(h(x))].d(x)/d(x)
In this way these all rules make.
• d [ Cos(h(x)) ] / dx = [ Sin(h(x)) ] . d(x)/d(x)
• d [ tan(h(x)) ] / dx = [ Sec(h(x))^2 ] . d(x)/d(x)
• d [ Cot(h(x)) ] / dx = [ - Cosec(h(x))^2 ] . d(x)/d(x)
• d [ Sec(h(x)) ] / dx = [ - Sec(h(x)).tan(h(x)) ] . d(x)/d(x)
• d [ Cosec (h(x)) ] / dx = [ - Cosec(h(x)).Cot(h(x)) ] . d(x)/d(x)
d/d(x) represents derivative with respect to variable x means we are differentiate here with respect to x in this formula.
In this video we will solve 3 questions related to the topic.So that you people have a better understanding and can easily solve every type of related question to this topic like derivative of hyperbolic functions proof.
Questions are:
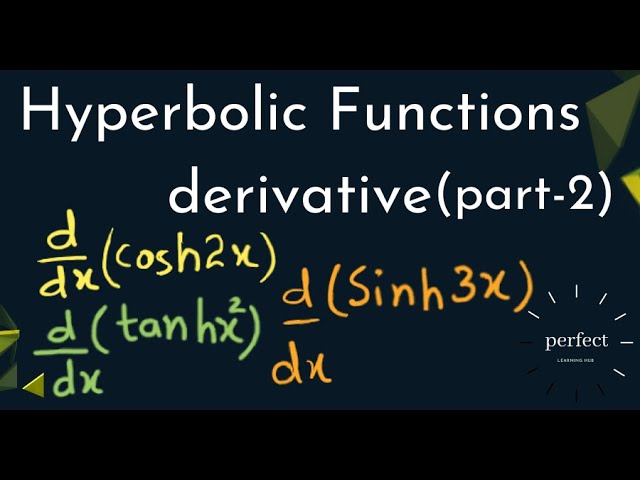
Differentiate this function with respect to X:
1. If y =Cosh(2x), dy/dx=?
2. If y =Sinh(3x), dy/dx=?
3. If y =Tanh(x)^2, dy/dx=?
We will solve these questions by using basic rules.
In our previous video (part 1) we explained that what are hyperbolic functions derivatives and the basic rules for the derivatives of hyperbolic functions and how to find these basic rules.
If you have not watch this video please watch that video first and then after it this video will be more beneficial for you.
link of the video is:
Questions are explained in the video. In this way you can easily solve all related questions by using this concept.
#differentiation#sinhx#coshx#tanhx#sechx#cosechx#cothx#sinh3x#cosh2x#tanhx^2
In perfect learning hub this video tutorial we will explain derivative of hyperbolic functions and how to find derivatives of hyperbolic trig functions examples and solutions.
Hyperbolic functions are analogs of the ordinary trigonometric functions defined for the hyperbola rather than on the circle.
Formulas for hyperbolic functions are:
Sinhx= [e^(x) – e^(-x)]/2 ,
Coshx= [e^(x) + e^(-x)]/2 ,
tanhx= [e^(x) – e^(-x)]/[e^(x) + e^(-x)] ,
Cosechx= 2/[e^(x) – e^(-x)] ,
Sechx= 2/[e^(x) + e^(-x)] ,
Cothx= [e^(x) + e^(-x)]/[e^(x) – e^(-x)] , here x is an element of set of real numbers.
Basically this hyperbolic functions derivatives in Hindi and urdu is especially for the calculus,mathematics students of class 12, fsc, ics, csbe,nscrt. We try to focus on derivatives class 12 math and explained chapter 2 completely. And also for the university students who are studying calculus, multivariable calculus, business mathematics etc.
So according to this video we can differentiate our questions by using derivative rules or formulas of hyperbolic functions.
derivative rules of hyperbolic functions are:
• d [ Sin(h(x)) ] / dx = [ Cos(h(x)) ] . d(x)/d(x) here x is an angle and dx/dx represents angle’s derivative with respect to x.
because we know:
d [ Sin(h(x)) ] / dx = d[(e^(x) – e^(-x))/2] /dx
= 1/2. [(e^(x) – e^(-x)(-1)]
= 1/2. [(e^(x) + e^(-x)]
= [Cos(h(x))].d(x)/d(x)
In this way these all rules make.
• d [ Cos(h(x)) ] / dx = [ Sin(h(x)) ] . d(x)/d(x)
• d [ tan(h(x)) ] / dx = [ Sec(h(x))^2 ] . d(x)/d(x)
• d [ Cot(h(x)) ] / dx = [ - Cosec(h(x))^2 ] . d(x)/d(x)
• d [ Sec(h(x)) ] / dx = [ - Sec(h(x)).tan(h(x)) ] . d(x)/d(x)
• d [ Cosec (h(x)) ] / dx = [ - Cosec(h(x)).Cot(h(x)) ] . d(x)/d(x)
d/d(x) represents derivative with respect to variable x means we are differentiate here with respect to x in this formula.
In this video we will solve 3 questions related to the topic.So that you people have a better understanding and can easily solve every type of related question to this topic like derivative of hyperbolic functions proof.
Questions are:
Differentiate this function with respect to X:
1. If y =Cosh(2x), dy/dx=?
2. If y =Sinh(3x), dy/dx=?
3. If y =Tanh(x)^2, dy/dx=?
We will solve these questions by using basic rules.
In our previous video (part 1) we explained that what are hyperbolic functions derivatives and the basic rules for the derivatives of hyperbolic functions and how to find these basic rules.
If you have not watch this video please watch that video first and then after it this video will be more beneficial for you.
link of the video is:
Questions are explained in the video. In this way you can easily solve all related questions by using this concept.
0:10:08
0:11:03
0:12:48
0:18:27
0:02:15
0:07:34
0:15:29
0:00:08
0:09:45
0:07:55
0:00:52
0:15:19
1:12:00
0:04:21
0:03:57
0:01:12
0:29:39
0:08:41
0:01:52
0:10:14
0:00:58
0:01:22
0:00:45
0:15:32