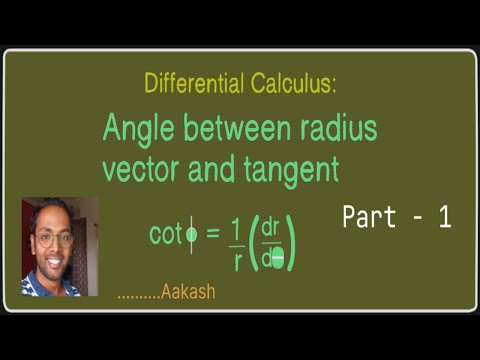
Angle Between Radius Vector and tangent: Solution | r^m=a^m(cos⁡(mθ)+sin⁡(mθ))

In this video, we dive deep into the fascinating world of polar coordinates and the relationship between the radius vector and tangent line. We start with the expression for the radius in terms of angular parameters, rm=am(cos⁡(mθ)+sin⁡(mθ))
r_m = a_m (\cos(m\theta) + \sin(m\theta))
rm​=am​(cos(mθ)+sin(mθ)),
and demonstrate how to derive the angle Φ\PhiΦ using logarithmic differentiation.

Join us as we:

Explore the derivation of the angle between the radius vector and the tangent line.

Use logarithmic properties to simplify our equations.
Differentiate with respect to 𝜃 to find the relationship between 𝑟 and 𝜃.
Discover the elegant expression for Φ in terms of mθ.
Find Angle blw radius Vector & Tangent.
rm = am (cos mθ + sin mθ)
take log on B.S
log rm = log am (cos mθ + sin mθ)
(log ab = log a + log b)
m log r = log am + log (cos mθ + sin mθ)
m log r = m log a + log (cos mθ + sin mθ)
differentiate w.r.t θ
m. 1/r . dr/dθ = m x 0 + 1/(cos mθ + sin mθ) . (-m sin mθ + m cos mθ)
m/r . dr/dθ = m cos mθ - m sin mθ / cos mθ + sin mθ
cot Φ = sin mθ + cos mθ / cos mθ - sin mθ
cot Φ = - tan mθ + 1 / 1 + tan mθ
cot Φ = cot (π/4 + mθ)
Φ = π/4 + mθ

Whether you're a student of mathematics or simply curious about polar coordinates, this video will enhance your understanding of the geometric concepts at play. Don't forget to like, subscribe, and hit the notification bell for more insightful content!

[darkedits]

Tq video was very helpful
The step by step explanation was amazing can u make a video on Maclaren series concept