filmov
tv
Short proof of Abel's theorem that 5th degree polynomial equations cannot be solved
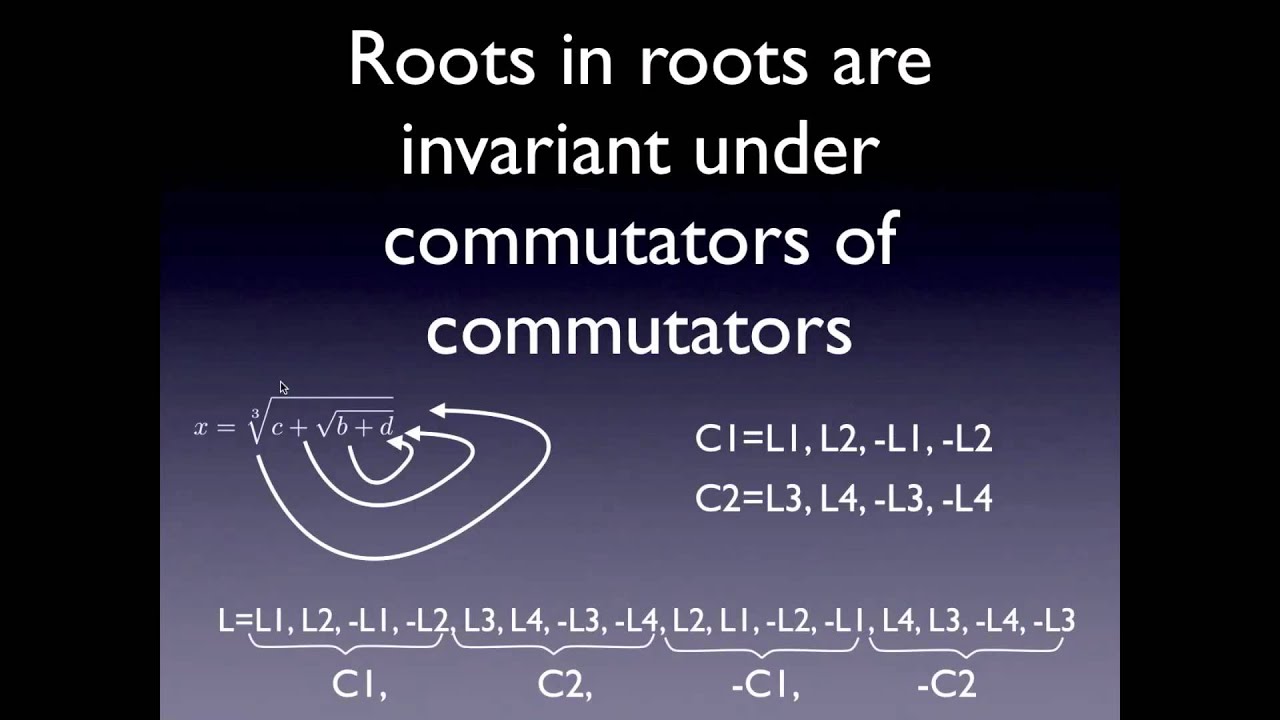
Показать описание
This is a shortened and slightly modified version of Arnold's proof. Familiarity with complex numbers is required to understand the proof.
Recommended further reading/viewing:
Recommended further reading/viewing:
Short proof of Abel's theorem that 5th degree polynomial equations cannot be solved
Differential Equation - 2nd Order (32 of 54) Abel's Theorem
Differential Equations | Abel's Theorem
Theorem: Abel's & Dirichlet's Tests || Semester:5th#utubeshorts #viralvideo #mathemati...
Differential Equations | Application of Abel's Theorem Example 1
Chapter 3: Abel's Theorem, it's idea, proof, and examples!
4.1 Abel's theorem
Abels theorem application
Mathematician Wins Abel Prize
What is...the Abel-Ruffini theorem?
How REAL Men Integrate Functions
Professor Earns 700k For Solving an Ancient Theorem
An Elementary Proof of Abel’s Theorem on Quintic Polynomials.
Abel's test | full video | link | #convergent
ABEL'S THEOREM complete analysis pattern iit jam +isi m math +ugc csir net +jee + iisc
'He just does math all day'
Abel Prize — The story
Niels ABEL 👨🎓 Mathematician
ABEL'S FORMULA | ABEL'S FORMULA DIFFERENTIAL EQUATIONS
Galois theory: Abel's theorem
Bro’s hacking life 😭🤣
But why is there no quintic formula? | Galois Theory
Abel–Ruffini theorem
'I loved every minute of it, however hard it had been'
Комментарии
 0:15:45
0:15:45
 0:03:52
0:03:52
 0:10:29
0:10:29
 0:00:16
0:00:16
 0:10:06
0:10:06
 0:19:50
0:19:50
 0:10:42
0:10:42
 0:19:59
0:19:59
 0:00:46
0:00:46
 0:32:44
0:32:44
 0:00:35
0:00:35
 0:01:07
0:01:07
 1:01:49
1:01:49
 0:00:15
0:00:15
 0:03:59
0:03:59
 0:00:11
0:00:11
 0:03:17
0:03:17
 0:05:23
0:05:23
 0:07:36
0:07:36
 0:27:53
0:27:53
 0:00:20
0:00:20
 0:11:59
0:11:59
 0:10:40
0:10:40
 0:03:31
0:03:31