Recent progress in peridynamic theory

This is a keynote lecture presented at the 2nd International Workshop on Plasticity, Damage and Fracture of Engineering Materials organized virtually from Middle East Technical University on 18-20 August 2021, Ankara, Turkey
Please contact the corresponding author for your comments/questions.

Corresponding (presenting) author: Erdoğan Madenci
Dep. Aerospace and Mechanical Engineering, The University of Arizona, USA
Authors: E. Madenci, D. Behera, P. Roy

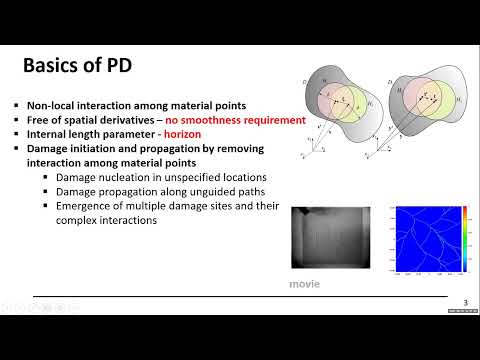
Abstract: Peridynamics, failure, polymers, viscoelasticity, creep, coupling with FEM. Peridynamic (PD) theory is a non-classical continuum theory based nonlocal interactions between the material points in a body. The interaction occurs between two material points over a finite distance referred to the horizon. The PD equation of motion is an integrodifferential equation, and the integrand is free of spatial derivatives of field variables. Therefore, it is applicable in presence of discontinuities in the domain. PD theory is primarily applied to predict crack initiation and propagation in materials. Cracking is achieved through removing the interactions (bond) between the material points.

The PD equations of motion can be broadly classified as Bond-Based (BB), Ordinary State-Based (OSB), and Non-Ordinary State-Based (NOSB) PD. In the BB-PD, the interaction of a pair of material points is independent of the influence of other points in their domain of interaction. However, the OSB- and NOSB-PD is dependent on the influence of other points within their individual domains of interaction. Among the three models, NOSB PD otherwise known as the correspondence model is attractive because of its ability to employ existing constitutive relations for material models.

The force density vectors in PD equilibrium equations are derived both material and geometric nonlinearities. The nonlocal deformation gradient tensor is computed in a bondassociated domain of interaction using the PD differential operator. The PD differential operator enables the construction of the nonlocal form of these equations by introducing an internal length parameter (horizon) that defines association among the points within a finite distance. This study presents recent developments in PD theory such as the direct imposition of local boundary conditions and its coupling with finite element method in ANSYS framework. Also, it presents PD simulations of finite elastic deformation and rupture in rubber like materials, soft polymers, viscoelastic adhesives in bonded lap joints, creep at high temperature and beam structures under quasi static loading conditions.