The characteristic polynomial is cooler than you thought!

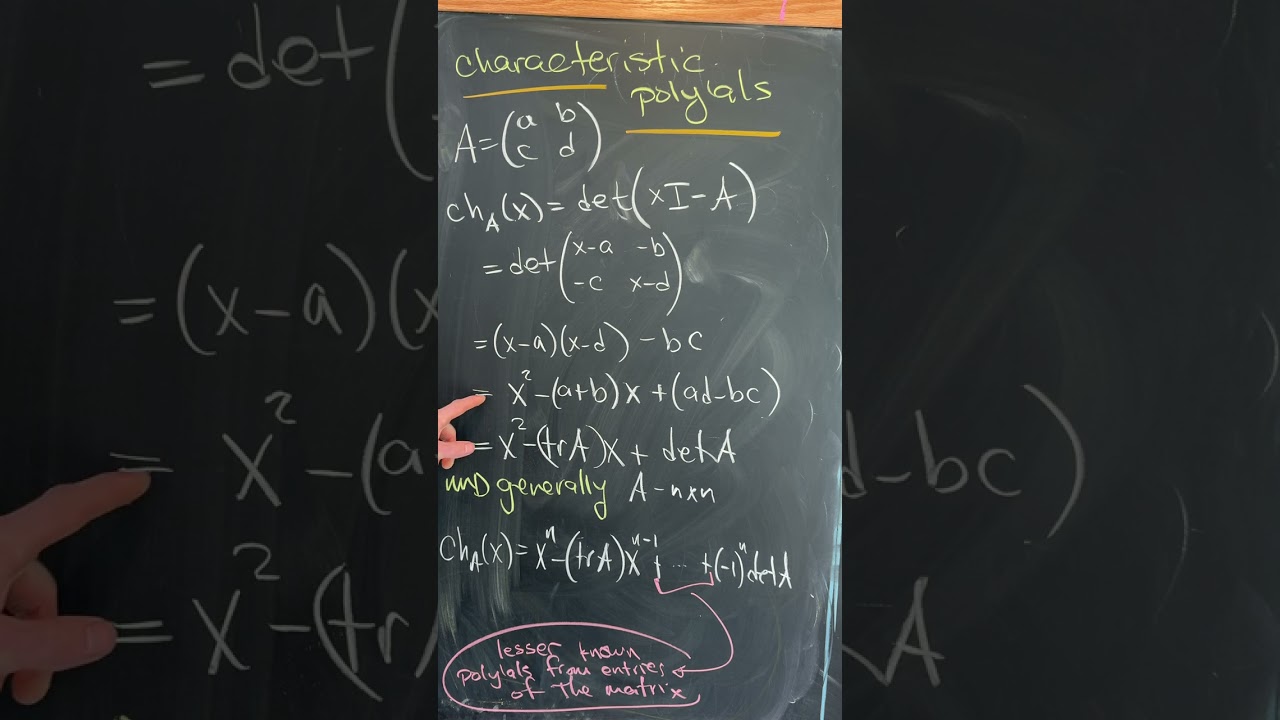
Показать описание

🌟Support the channel🌟

🌟my other channels🌟

🌟My Links🌟

🌟How I make Thumbnails🌟

🌟Suggest a problem🌟

Рекомендации по теме

Комментарии

What are those less known prefactors for e.g. 4×4? Do they have a specific name?

eiseks

If I remember correctly, the trace and the determinant are invariants of the matrix (they don't change value under linear transformations). Are those other coeficientes of the n×n characteristic polynomial also invariants?

InverseTachyonPulse

Cayley Hamilton theorem, it is fascinating. Also, an eigenvector solves the characteristic equation

joseivan

So if the eigenvalues span the eigenspace and the geometric multiplicity matches the algebraic multiplicity, we have a bijective relation between the eigenvalues and any arbitrary combination of the terms of a polynomial, as long as the dimensions of the eigenspace and the polynomial match. This indicates that the matrix is diagonalizable and that the characteristic equation provides a complete and direct representation of the eigenspace itself. If these conditions are not met, then you'll encounter residual terms (dependencies) that need to be addressed. I haven't seen this particular form of the characteristic equation before, but it makes sense given that the trace is just the sum of the eigenvalues counted with its algebraic multiplicity!

jaredroussel

I'd like to learn more about this, thanks! 😀

JJ_TheGreat

So basically the trace determines the threshold of its eigenvalues?

alexandershapiro

What is the "trace" of a matrix?
Thanks!

JJ_TheGreat

This has no business being in my feed. Good luck to you😂

sethoakes

Wow nice characteristic you have there.

joshgulrud

Do you think you know math when you pass your calculus class? Just wait for some guy on YouTube shorts to do algebra 💀

marshall

Michael, I know this equation has no answer
e^x=0
But if we use the Taylor series of the function, we will have a polynomial whose degree is infinity
x^(infinity) +....+x/2!+x/1!+1=0
I know that the fundamental theorem of algebra requires that this infinity has an answer But on the other hand, the equation should not have an answer Why is it like this?Shouldn't we use the Taylor series?

MortezaSabzian-dbsl

The characteristic polynomial is cooler than you thought!

The characteristic polynomial is cooler than you thought!

22. Ways to compute the characteristic polynomial of a hyperplane arrangement. Finite field method.

What is...the characteristic polynomial?

The Characteristic Polynomial: Part 1/2 'The Characteristic Polynomial'

Find the characteristic polynomial of the matrices

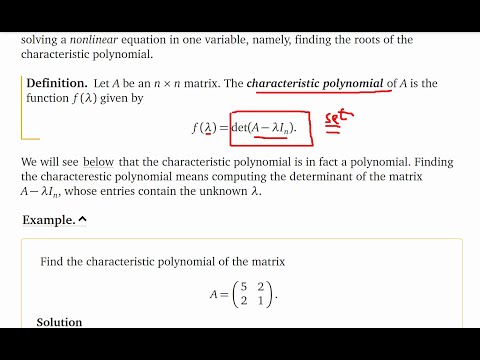
Section 5.2 The Characteristic Polynomial

Labtube-(Linear Algebra I)- Characteristic Polynomial of Matrices

8.7. Trace and Determinant from characteristic polynomial

SAT Prep || Understanding Polynomials part2

Labtube-(AMath1201)-Finding Eigenvalues: Characteristic Polynomials

20. The characteristic polynomial. Duality between zonotopes and hyperplane arrangements.

Definition and example of CHARACTERISTIC POLYNOMIAL

Linear Algebra Lecture 37: Characteristic Polynomial

Module 2 Finding the Periods of the Ducci Map as Orders of Polynomials

05 Polynomial Characteristics From Factored Equation

The Characteristic Polynomial: Part 5/5 'Sage'

The Characteristic Polynomial: Part 4/5 'Trace and Determinant'

(sec5.2) :The characteristic equation

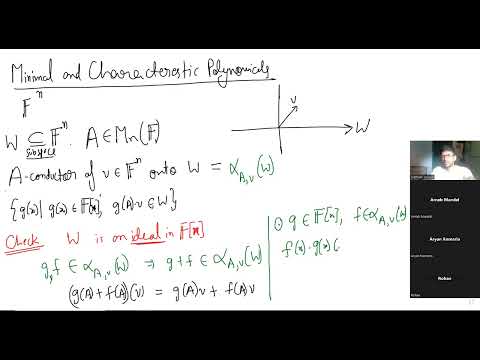
Session 10c: Defining the Minimal and Characteristic polynomial for Matrices

The characteristic polynomial of random matrices (...) - Eliott Paquett

A Second Order ODE | Solved using Characteristic Polynomial

A quick trick for computing eigenvalues | Chapter 15, Essence of linear algebra

The Characteristic Equation, part 4

Characteristics From Polynomial Equation K1