filmov
tv
Minimal Polynomials and Diagonal Form

Показать описание
Matrix Theory: Using minimal polynomials, we characterize matrices that can be put into diagonal form. That is, there exists a basis of eigenvectors for A iff the minimal polynomial factors into distinct linear factors. As an application, we show that any matrix that satisfies A^m=I (m positive) is diagonalizable over the complex numbers.
Minimal Polynomials and Diagonal Form
Minimal Polynomial of Block diagonal Matrix| Characteristic polynomial |Linear Algebra|Mathematics
Université de Paris gre math jordan canonical form minimal polynomial
Example of Minimal Polynomial
Lecture 29 : Minimal Polynomial and Jordan Canonical Form-I
The Minimal Polynomial
Lecture 30 : Minimal Polynomial and Jordan Canonical Form-II
Linear Algebra: 036 The Minimal Polynomial
Introduction to algebraic number theory. Class 3. V. Zhgun
MINIMAL POLYNOMIAL EXAMPLES | PROBLEM -1
Linear Algebra | Minimal Polynomial and Diagonalizability in One Shot by GP Sir
minimal polynomial linear algebra tifr 2018 geometric multiplicity
Johns Hopkins University gre math minimal polynomial jordan canonical form linear algebra
Linear Algebra : Minimal Polynomial Part-I
Linear Algebra: 041 When the Minimal Polynomial Does Not Split
Minimal polynomial for diagonalisable transformation
Minimal polynomial of block diagonal matrix
Relationship between Characteristic Polynomial & Minimal Polynomial | Diagonalizability
Characteristic Polynomial Of A Matrix In 10 Seconds | Matrices 4x4 & 5x5 @gajendrapurohit
Minimal Polynomial and Jordan Canonical Form-I
How to find the minimal polynomial of matrix| Example| Linear Algebra
Overview of Minimal Polynomials
Linear Algebra: 039 Schur's Theorem When the Minimal Polynomial Splits
Minimal polynomials
Комментарии
 0:16:56
0:16:56
 0:06:33
0:06:33
 0:02:55
0:02:55
 0:06:07
0:06:07
 0:31:00
0:31:00
 0:15:35
0:15:35
 0:25:58
0:25:58
 1:01:56
1:01:56
 2:42:45
2:42:45
 0:05:18
0:05:18
 0:58:42
0:58:42
 0:08:47
0:08:47
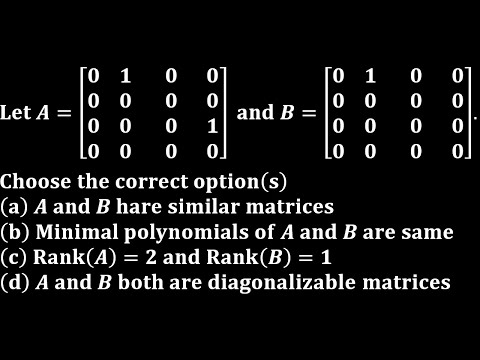 0:03:23
0:03:23
 0:14:16
0:14:16
 0:47:30
0:47:30
 0:09:38
0:09:38
 0:07:25
0:07:25
 0:15:01
0:15:01
 0:06:14
0:06:14
 0:31:00
0:31:00
 0:09:48
0:09:48
 0:16:42
0:16:42
 0:41:45
0:41:45
 0:14:52
0:14:52