filmov
tv
Amy Huang: Tensor Ranks and Matrix Multiplication Complexity
Показать описание
Amy Huang is a postdoc at Texas A&M
Tensor Ranks and Matrix Multiplication Complexity
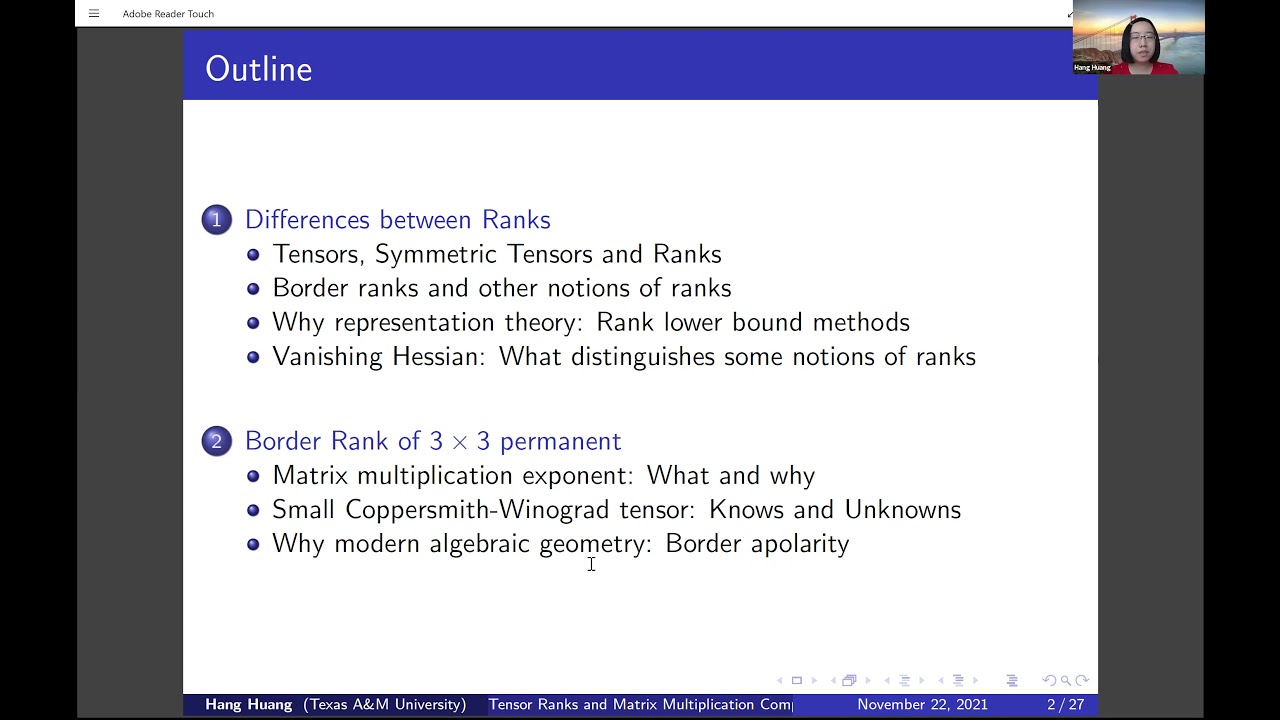
Abstract: Tensors are just multi-dimensional arrays. Notions of ranks and border rank abound in the literature. Tensor decompositions also have a lot of application in data analysis, physics, and other areas of science. I will try to give a colloquium-style talk surveying my recent two results about tensor ranks and their application to matrix multiplication complexity. The first result relates different notions of tensor ranks to polynomials of vanishing Hessian. The second one computes the border rank of 3×3 permanent. I will also briefly discuss the newest technique we used to achieve our results: border apolarity.
Tensor Ranks and Matrix Multiplication Complexity
Abstract: Tensors are just multi-dimensional arrays. Notions of ranks and border rank abound in the literature. Tensor decompositions also have a lot of application in data analysis, physics, and other areas of science. I will try to give a colloquium-style talk surveying my recent two results about tensor ranks and their application to matrix multiplication complexity. The first result relates different notions of tensor ranks to polynomials of vanishing Hessian. The second one computes the border rank of 3×3 permanent. I will also briefly discuss the newest technique we used to achieve our results: border apolarity.
0:51:27
0:21:39
0:05:48
1:15:49
0:28:53
0:16:21
1:00:49
1:01:01
0:48:37
0:19:22
0:57:33
1:03:32
1:01:01
0:02:04
2:06:34
0:22:02
1:03:03
1:01:54
1:05:03
0:31:14
0:22:01
0:43:54
1:02:07
0:54:33