filmov
tv
Hypothesis Testing: Conclusion (one sample t test) I Statistics 101 #6 | MarinStatsLectures
Показать описание
Hypothesis Testing: Conclusion (one sample t test): how to use a p value or critical value to reject or fail to reject a null hypothesis?
In the sixth video in Statistics 101 Hypothesis Testing series, we will learn how to use a p value or critical value to decide if you should reject or fail to reject a null hypothesis in the context of a one-sample t-test.
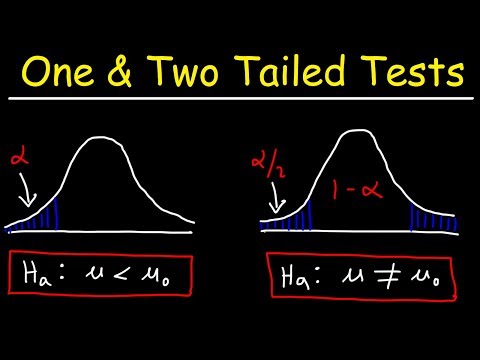
►We can decide whether to “reject our null hypothesis and claim evidence that the alternative hypothesis is likely true” or “fail to reject our null hypothesis and claim non-sufficient evidence to believe the alternative hypothesis to be true” by either comparing our p value to a significance level (alpha) or by comparing our test statistic to a critical value. A significance level (alpha) of 5% is commonly used, although this is not a “magic number”. If the p value is less than alpha we can reject our null, while if the p value is greater than alpha we fail to reject our null. Similarly, if the test statistic is greater than the critical value (in absolute value) we can reject our null, while if the test statistic is smaller than the critical value (in absolute value) we will fail to reject our null.
►A Critical Value or a Rejection Region can be applied to a test statistic to help us decide whether or not we believe the Null or Alternative hypothesis to be true. The larger the test statistic (in absolute value) the more evidence we have that the null is false and the alternative is true. But how large does the test statistic have to be before we believe the null to be false and the alternative to be true? The Critical Value can help us decide “how large is large enough for us to discredit the null and believe the alternative”?
►A p-value is another way for us to decide whether or not we will reject our null hypothesis and claim evidence that the alternative hypothesis is true. Recall that we begin the test by assuming the null hypothesis to be true, and we will only “reject this null” and “believe the alternative to be true” if our test statistic is large (and our sample statistic, the sample mean, is incompatible with the null hypothesis being true). The p value gives us an estimate of “how likely we would be to obtain the sample statistic (the sample mean in this case) we did in our sample, or one even further from the null hypothesized value, if in fact the null hypothesis were true. Informally, you can think of it as telling you “what is the probability of seeing what we did in our data, or something even more extreme, if the null is true”. In order to reject the null and claim evidence that the alternative is true, we want a small p value. The p value can be compared to a significance level (alpha) to make this decision.
►► Watch More:
Follow MarinStatsLectures
Our Team:
Content Creator: Mike Marin (B.Sc., MSc.) Senior Instructor at UBC.
Producer and Creative Manager: Ladan Hamadani (B.Sc., BA., MPH)
These videos are created by #marinstatslectures to support some courses at The University of British Columbia (UBC) (#IntroductoryStatistics and #RVideoTutorials for Health Science Research), although we make all videos available to the everyone everywhere for free.
Thanks for watching! Have fun and remember that statistics is almost as beautiful as a unicorn!
In the sixth video in Statistics 101 Hypothesis Testing series, we will learn how to use a p value or critical value to decide if you should reject or fail to reject a null hypothesis in the context of a one-sample t-test.
►We can decide whether to “reject our null hypothesis and claim evidence that the alternative hypothesis is likely true” or “fail to reject our null hypothesis and claim non-sufficient evidence to believe the alternative hypothesis to be true” by either comparing our p value to a significance level (alpha) or by comparing our test statistic to a critical value. A significance level (alpha) of 5% is commonly used, although this is not a “magic number”. If the p value is less than alpha we can reject our null, while if the p value is greater than alpha we fail to reject our null. Similarly, if the test statistic is greater than the critical value (in absolute value) we can reject our null, while if the test statistic is smaller than the critical value (in absolute value) we will fail to reject our null.
►A Critical Value or a Rejection Region can be applied to a test statistic to help us decide whether or not we believe the Null or Alternative hypothesis to be true. The larger the test statistic (in absolute value) the more evidence we have that the null is false and the alternative is true. But how large does the test statistic have to be before we believe the null to be false and the alternative to be true? The Critical Value can help us decide “how large is large enough for us to discredit the null and believe the alternative”?
►A p-value is another way for us to decide whether or not we will reject our null hypothesis and claim evidence that the alternative hypothesis is true. Recall that we begin the test by assuming the null hypothesis to be true, and we will only “reject this null” and “believe the alternative to be true” if our test statistic is large (and our sample statistic, the sample mean, is incompatible with the null hypothesis being true). The p value gives us an estimate of “how likely we would be to obtain the sample statistic (the sample mean in this case) we did in our sample, or one even further from the null hypothesized value, if in fact the null hypothesis were true. Informally, you can think of it as telling you “what is the probability of seeing what we did in our data, or something even more extreme, if the null is true”. In order to reject the null and claim evidence that the alternative is true, we want a small p value. The p value can be compared to a significance level (alpha) to make this decision.
►► Watch More:
Follow MarinStatsLectures
Our Team:
Content Creator: Mike Marin (B.Sc., MSc.) Senior Instructor at UBC.
Producer and Creative Manager: Ladan Hamadani (B.Sc., BA., MPH)
These videos are created by #marinstatslectures to support some courses at The University of British Columbia (UBC) (#IntroductoryStatistics and #RVideoTutorials for Health Science Research), although we make all videos available to the everyone everywhere for free.
Thanks for watching! Have fun and remember that statistics is almost as beautiful as a unicorn!
0:02:01
Hypothesis Testing: Conclusion (one sample t test) I Statistics 101 #6 | MarinStatsLectures
0:04:00
Hypothesis Testing - Introduction
0:13:34
Hypothesis Testing Problems - Z Test & T Statistics - One & Two Tailed Tests 2
0:05:03
Introduction to Type I and Type II errors | AP Statistics | Khan Academy
0:00:51
Hypothesis Testing 🔥 Explained in 60 Seconds
0:05:07
Making a Hypothesis Test Conclusion
0:01:34
Writing the Final Conclusion for the Hypothesis Testing
0:01:59
Statistical Significance, the Null Hypothesis and P-Values Defined & Explained in One Minute
1:48:33
Workshop on Research Methodology Software: EViews and JASP
0:06:01
Hypothesis Testing: the conclusion
0:01:32
Hypothesis Testing: the conclusion (a quick explanation)
0:05:41
One Tailed and Two Tailed Tests, Critical Values, & Significance Level - Inferential Statistics
0:09:36
Hypothesis Test Decision and Conclusion Steps
0:06:52
Hypothesis Testing - Null and Alternative Hypotheses
0:16:34
Hypothesis Testing Conclusion| Statistics Course Part 7
0:04:49
One Sample t-Test
0:00:42
Reject or Fail to Reject - Intro to Inferential Statistics
0:07:34
Statistics Hypothesis Testing Part1 (Formal Conclusion)
0:11:45
Tutorial 31- Hypothesis Test, Type 1 Error, Type 2 Error
0:02:23
Hypothesis Testing: One-tailed t test for mean
0:05:19
Hypothesis Tests: Decisions & Conclusions.mp4
0:02:10
Hypothesis Testing: Errors and Power (one sample t test) I Statistics 101 #7 | MarinStatsLectures
0:10:38
Lesson: One Sample Hypothesis Testing
0:07:58
P-values and significance tests | AP Statistics | Khan Academy
Комментарии