filmov
tv
Math 202 Lecture 8 - L'Hôpital's rule part 2 and intro to integration by parts
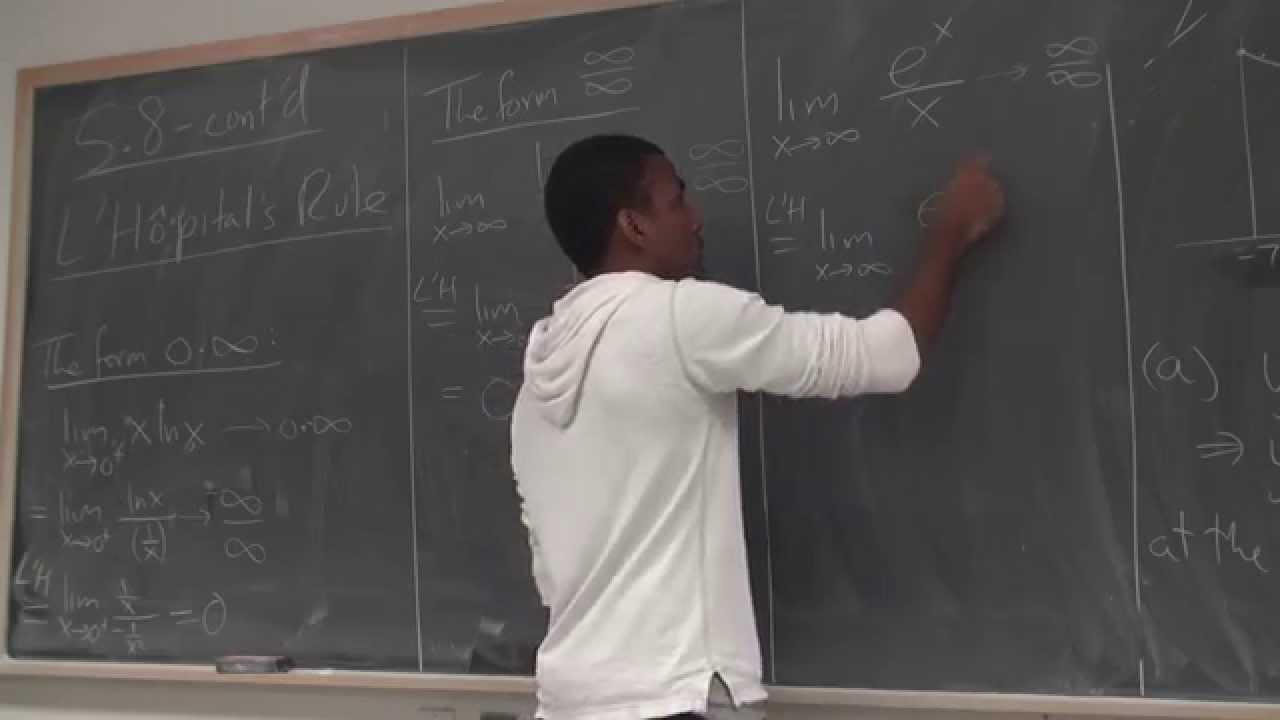
Показать описание
In today's lecture, we first go over a few homework problems regarding hyperbolic trig functions and their derivatives and integrals. We then wrap up our discussion on L'Hôpital's rule, where we do many more examples--in particular, examples that have varying powers that invite the help of logarithms and exponentials to deal with.
At the end of the class, we introduce a new integration technique, the reverse of the product rule--integration by parts. This technique allows us to integrate functions inaccessible by the basic rule and integration by substitution. We derive the formula for integration by parts and do one example. We shall refine the method and do more examples next time.
At the end of the class, we introduce a new integration technique, the reverse of the product rule--integration by parts. This technique allows us to integrate functions inaccessible by the basic rule and integration by substitution. We derive the formula for integration by parts and do one example. We shall refine the method and do more examples next time.
Discrete Math #8 | Venn Diagram | Kinza Bilal
MTH202 Discrete Mathematics Lecture 8 in Urdu / Hindi. VU MTH202 (Discrete Mathematics)Short Lecture
mth202 updated short lecture 8 | vu updated lectures 2024 | by Mahnoor
MTH202 Lecture 8 Venn Diagram (part 2) with Examples & Exercise | Be Educated VU
MTH202 Lecture 8 Venn Diagram (part 1) with Examples & Exercise | Be Educated VU
MTH001 (Elementary/Discrete Mathematics) Lecture No.8 (Sets Continued)
Math 202 Lecture 8 - L'Hôpital's rule part 2 and intro to integration by parts
Lecture 8 - MULTISETS and MULTICHOOSE // Combinatorics Discrete Math
NUMBER SYSTEM II MATH II RRB NTPC II RAILWAY RECRUITMENT 2024 II CLASS 2
MTH101 Lecture 8 | Graphs of functions | Kinza Bilal
Mth202 lecture 21, 22 recursion by Ameer Hamza
MTH301 lecture # 8 and 9 The chain rule by ameer hamza
MTH202 Discrete Mathematics Lecture 9 in Urdu / Hindi. VU MTH202 (Discrete Mathematics)Short Lecture
Mth104 lecture no 8 & 9(sets and logics)||#mth104#lecture8 #9#sets #logics#iqraazhar
MTH202 Discrete Mathematics Lecture 7 in Urdu / Hindi. VU MTH202 (Discrete Mathematics)Short Lecture
Desbloquea tu calculadora 🧐 #shorts #ingedarwin
MTH102 lecture 8 by Waqar Institute | virtual university | Sir Vicky | Short lectures by waqar
Wael || MATH 260 || Lecture #8
Business Maths - Lecture 8
Mth202 lecture # 11, 12 short lectures by Ameer Hamza |relations |
Discrete Mathematics for Computer Science
Intro to Matrices
Math 202 Lecture 18 - Areas between curves conclusion and the disk method
Mth202 practice questions lecture 1 to 6|| mid term practice questions 2023|#mth202
Комментарии
 0:36:14
0:36:14
 0:50:50
0:50:50
 0:07:42
0:07:42
 0:18:15
0:18:15
 0:18:20
0:18:20
 0:39:49
0:39:49
 1:03:00
1:03:00
 0:11:43
0:11:43
 0:47:36
0:47:36
 0:21:15
0:21:15
 0:08:17
0:08:17
 0:18:42
0:18:42
 0:52:33
0:52:33
 0:10:23
0:10:23
 0:36:35
0:36:35
 0:00:47
0:00:47
 0:15:22
0:15:22
 1:09:31
1:09:31
 1:59:15
1:59:15
 0:09:20
0:09:20
 0:03:15
0:03:15
 0:11:23
0:11:23
 1:26:08
1:26:08
 0:17:54
0:17:54