filmov
tv
Lecture 34 | Continuity and Compactness | Continuous functions on compact spaces | Analysis | Tamil
Показать описание
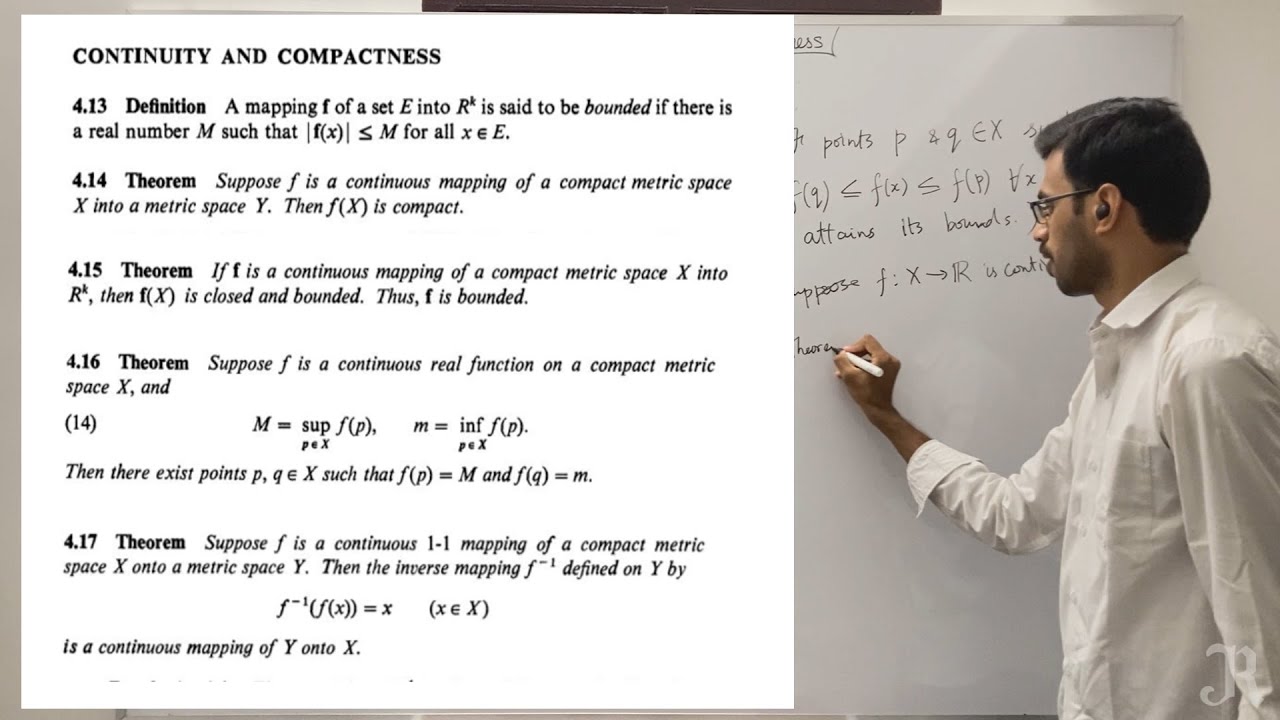
* Definition of bounded functions.
* Continuous image of a compact set (metric space) is compact with proof.
* Continuous real valued function on compact sets attains its maximum and minimum.
* Continuous function on compact set into R^k is bounded.
* If f is a one-one, onto, continuous function defined on compact set, then its inverse is continuous.
================================================
* Reference book: Walter Rudin, Principles of Mathematical Analysis (Third edition).
* For PG students studying "Analysis I".
* Especially for M.Sc. Mathematics (First semester) students under Manonmaniam Sundaranar University (M S University), Tirunelveli.
* Useful for SET/NET/GATE/other competitive examinations.
* Continuous image of a compact set (metric space) is compact with proof.
* Continuous real valued function on compact sets attains its maximum and minimum.
* Continuous function on compact set into R^k is bounded.
* If f is a one-one, onto, continuous function defined on compact set, then its inverse is continuous.
================================================
* Reference book: Walter Rudin, Principles of Mathematical Analysis (Third edition).
* For PG students studying "Analysis I".
* Especially for M.Sc. Mathematics (First semester) students under Manonmaniam Sundaranar University (M S University), Tirunelveli.
* Useful for SET/NET/GATE/other competitive examinations.
0:02:44
Lecture 34, Equation of Continuity
0:09:06
Maths Continuity & Differentiability part 34 (Second Order Derivative) CBSE Mathematics XII 12
0:09:27
Kerala State 12th math -Ch. 5 - Continuity & Differentiability -Part 34 - Mean Value Theorem
0:14:48
Lecture 34 | Second Order Derivative | Continuity And Differentiability | Class 12
0:06:06
Class XII Chapter 5 Continuity And Differentiability (Lecture 34)
0:28:42
Exercise 5.1 class12 maths ncrt |Q.no25 to 34| Continuity 4 marks important questions
0:25:29
34 Continuity of entropy
0:13:46
Continuity of an absolute value function | Q10 to Q13 | @HDMATHEMATICSBYHDSIR || Lecture 34
0:10:15
Lecture 34/class 12 /maths/continuity and differentiability
0:31:11
Lecture 34 | Continuity and Compactness | Continuous functions on compact spaces | Analysis | Tamil
1:18:35
Fluid Mechanics: Continuity Equation, Bernoulli Equation, & Kinematics Examples (10 of 34)
0:19:12
CSIR NET Mathematics Solution Dec 2019 |Question 34|Complex Analysis|Continuity|Holomorphic|Analytic
0:49:59
Mis. Chapter 5 | Lecture 34 | NCERT | Continuity and Differentiability | Revised syllabus 2020
0:28:31
Ch#1| Continuity of a Function at a number with Examples 34 to 37 |Calculus by SM Yusuf lec24 Hindi
0:21:08
Lecture-34/1-Sem-Maths /Unit_III:Uniformly Continuity and problems by Ramanjan sir.
0:53:04
Solution of exercise 5.1 | Class 12 | Q20-Q34 | Continuity and Differentiability | Lecture 5
0:41:11
Class 12 Continuity and Differentiability 5.1 Part7-Qs#31-34 (In English)- NCERT CBSE
0:04:01
Lec 34-Continuity Equation & Relaxation Time,Electromagnetic Fields Theory|| Electrical Engineer...
0:06:26
12th Maths (NCERT) Ex. 5.1 Q 34, Class 12 Maths Continuity & Differentiability
0:59:20
Intro Real Analysis, Lec 34: p-Norm, Sup Norm, Continuity & Preimages, Images of Compact Sets
0:19:56
Lecture on Continuity
0:32:15
STD_12TH SCIENCE#CH-5:CONTINUITY AND DIFFERENTIABILITY#LECTURE-34
1:02:51
Ex-5.1(QNo 21 to QNo 34) Continuity and differentiability || Class 12 Maths NCERT
0:26:49
L-34: Real Analysis (Definition of limit and continuity)
Комментарии