Bound for Supremum of the Intersection of Sets | Real Analysis Exercises

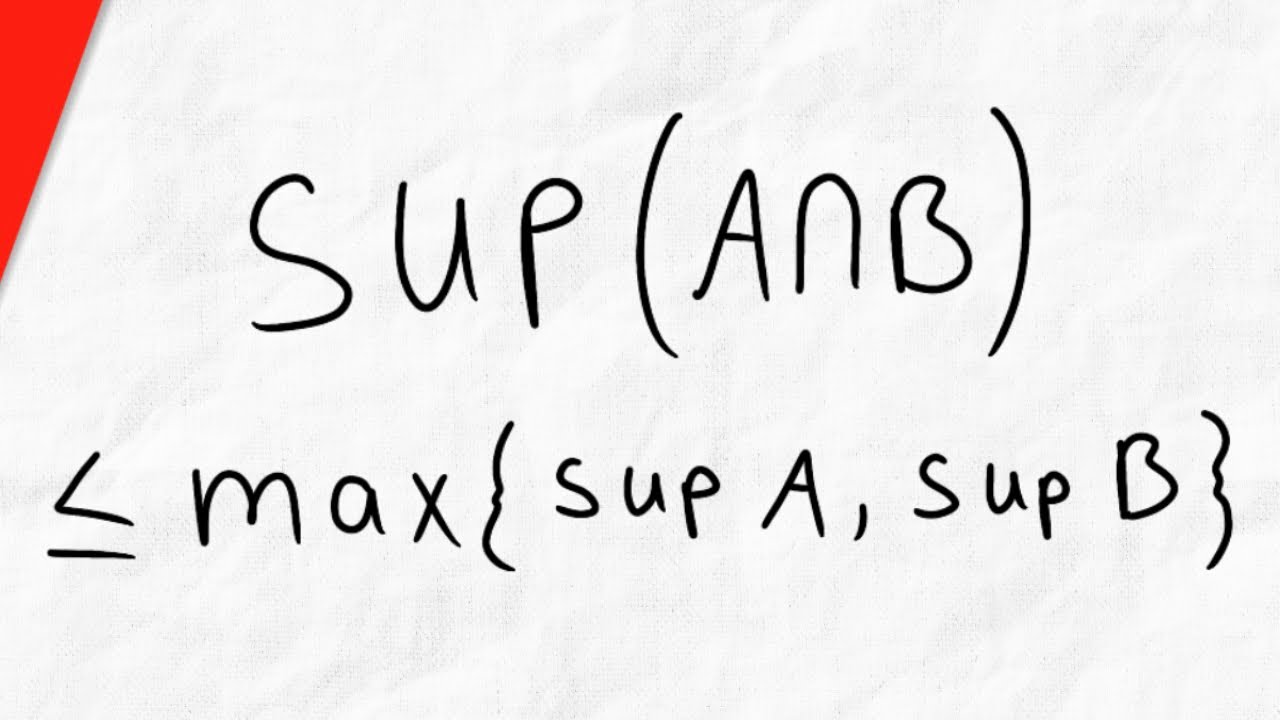
Показать описание

If A and B are two bounded and nonempty subsets of the real numbers, then what is the supremum of their intersection? What is sup(A intersect B)? We cannot say for sure in general, but we can place an upper bound on the supremum. If A and B are bounded nonempty subsets of the reals then we know they both have supremums by the completeness axiom. Similarly, their intersection must have a supremum. We'll prove that the supremum of A intersect B must be less than or equal to the maximum of supA and supB. This result will follow pretty easily from the definition of supremum being a least upper bound.

★DONATE★

Thanks to Robert Rennie and Barbara Sharrock for their generous support on Patreon!

Follow Wrath of Math on...

Рекомендации по теме

Комментарии

I had a problem with understanding real analysis, but I found things so simple with you. would you please make more videos explaining real analysis?

mrymfarhat

thank you so much, i really appreciate it, you make me love math, even though i'm an IT student :p

anonymousvevo

Shouldnt it be min( sup(A), sup(b)) i think this is wrong

thehardlife

go up more exercises are level exercises

samuelporras

Bound for Supremum of the Intersection of Sets | Real Analysis Exercises

Definition of Supremum and Infimum of a Set | Real Analysis

Real Analysis 6 | Supremum and Infimum

Real Analysis | The Supremum and Completeness of ℝ

7.3 The supremum and the infimum of a set

Supremum of the Union of Sets | Real Analysis

Proof: Supremum and Infimum are Unique | Real Analysis

The Supremum Approximation Theorem

2.4 Maximum, upper bound, supremum

Proof: Supremum of {n/(n+1)} = 1 | Real Analysis

Lemma An upper bound u of a nonempty set S in R is the supremum of S if and only if for every

Supremum, Infimum: Definition and Explanation

Least upper bound or Supremum of a set, if it exists, is unique || set theory || video no. -20 ||

Epsilon Definition of Supremum and Infimum | Real Analysis

Definition of the Least Upper Bound or Supremum of a Set

Difference between supremum and upper bound/ set bounded above

Supremum of a set

Examples of upper bound, lower bound, supremum and Infimum

How to find lub and glb || find infimum and supremum of a set

Upper Bounds, Lower Bounds, Supremum, Infimum, Bounded and Unbounded Set| Real Analysis Topology-1

Bounded sets (lower/upper bound, supremum/infimum)

What is the relationship between upper bounds and supremum?

2.5 Supremum: proof - sup(0,1)=1

Fundamental Properties of Supremum and Infimum, Including sup(A + B) = sup(A) + sup(B)

How to use the epsilon definition of sup in a proof