filmov
tv
Trigonometry: Amplitude, Reflection & Period/Vertical & Horizontal Translations (Sections 4.2 & 4.3)

Показать описание
Examples graphing sine and cosine functions that have changes to their amplitude and period, have been reflected and/or shifted vertically/horizontally (phase shift). Step by step breakdown of how to find all relevant characteristics and graph one period using five key points. #amplitude #period #phaseshift #translation #mathtvwithprofessorv #trigonometry #graphing #triggraphs #sine #cosine #domain #range #mathvideos #youtubemath #mathprofessor #calculus #precalculus
Don't forget to LIKE, Comment, & Subscribe!
xoxo,
Professor V
Calculus 2 Lecture Videos on Integration:
Trig Review:
Calculus 3 Video Lectures:
#mathwithprofessorv #integration #partialfractions #partialfractionmethod #partialfractiondecomposition #trigonometricsubstitution #trigintegrals #trigsubstitution #integrals #integralcalculus #calculus2 #calculusvideos #calculus2videos #integralvideos #trigonometricintegrals #math #youtubemath #mathvideos #mathtutor #mathprofessor #calculusvideos #integrationbyparts #integralcalculus
Join this channel to get access to perks:
Socials:
IG: @mathwithprofessorv
TikTok: @mathwithprofessorv
I'm also an Amazon influencer, so feel free to support and shop:
EXCITING NEWS: You can now sign up for my Patreon at the link below!
My Patreon is a place for students to have access to exclusive ad-free content not available on my YouTube channel! Access to the library of additional videos, worksheets, and more is available with the "Star Pupil Package" tier for just $9.99/month (USD).
*This video is copy protected and cannot be downloaded or used in any capacity without my permission.*
Don't forget to LIKE, Comment, & Subscribe!
xoxo,
Professor V
Calculus 2 Lecture Videos on Integration:
Trig Review:
Calculus 3 Video Lectures:
#mathwithprofessorv #integration #partialfractions #partialfractionmethod #partialfractiondecomposition #trigonometricsubstitution #trigintegrals #trigsubstitution #integrals #integralcalculus #calculus2 #calculusvideos #calculus2videos #integralvideos #trigonometricintegrals #math #youtubemath #mathvideos #mathtutor #mathprofessor #calculusvideos #integrationbyparts #integralcalculus
Join this channel to get access to perks:
Socials:
IG: @mathwithprofessorv
TikTok: @mathwithprofessorv
I'm also an Amazon influencer, so feel free to support and shop:
EXCITING NEWS: You can now sign up for my Patreon at the link below!
My Patreon is a place for students to have access to exclusive ad-free content not available on my YouTube channel! Access to the library of additional videos, worksheets, and more is available with the "Star Pupil Package" tier for just $9.99/month (USD).
*This video is copy protected and cannot be downloaded or used in any capacity without my permission.*
Комментарии
 0:31:40
0:31:40
 0:07:55
0:07:55
 0:01:59
0:01:59
 0:04:50
0:04:50
 0:05:44
0:05:44
 0:02:47
0:02:47
 0:10:07
0:10:07
 0:05:22
0:05:22
 0:03:54
0:03:54
 0:03:03
0:03:03
 0:02:44
0:02:44
 0:05:25
0:05:25
 0:01:32
0:01:32
 0:10:47
0:10:47
 0:10:41
0:10:41
 0:06:25
0:06:25
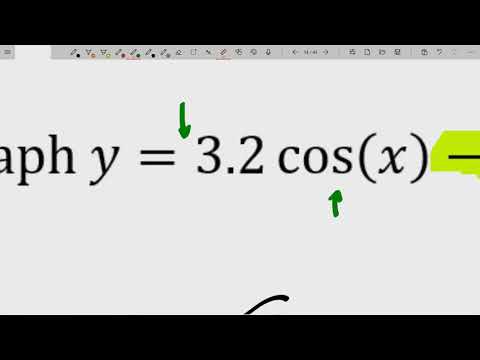 0:24:08
0:24:08
 0:03:50
0:03:50
 0:13:55
0:13:55
 0:08:14
0:08:14
 0:00:40
0:00:40
 0:11:54
0:11:54
 0:14:18
0:14:18
 0:00:14
0:00:14