filmov
tv
Bounded and Continuous Linear Transformations

Показать описание
In this video definitions bounded and continuous functions are given on a normed linear space.
Further, the following Theorem is given
Theorem:Let T : N to N' be a linear transformation from a normed linear space N to normed linear space N'. then the following statements are equivalent:
1. T is continuous
2. T is continuous at the origin
3. T is bounded
4. If S is a sunset of N containing all those elements whose norm are less than 1, then T(S) will be a bounded subset of N'.
Further, the following Theorem is given
Theorem:Let T : N to N' be a linear transformation from a normed linear space N to normed linear space N'. then the following statements are equivalent:
1. T is continuous
2. T is continuous at the origin
3. T is bounded
4. If S is a sunset of N containing all those elements whose norm are less than 1, then T(S) will be a bounded subset of N'.
Bounded and Continuous Linear Transformation Part 1 by Dr. Bharti Kapoor
Functional Analysis Module II Class 8 Linear operator is continuous iff its bounded
Example of bounded operator
Lec - 10 Continuous (Bounded) Transformation In Normed Space | Important Theorems on Continuity
Equivalent Theorem T is continuous at 0 if and only if T is bounded linear transformation
Bounded and Continuous Linear Transformations
Bounded and Continuous Linear Transformation Part 2 by Dr. Bharti Kapoor
Functional Analysis/Continuous Linear Transformation/Class-8
Bounded linear transformation and Bounded linear operator.
Banach space and continuous linear transformation
Lec - 15 Space Of Bounded Linear Transformation | Space of Bounded Transformation Is Banach Space
Bounded linear transformation and norm of bounded linear transformation
definition of bounded linear transformation, continuous transformation. bounded transformation.
lec10def of norm of continuous linear transformation nd composition of bdd operator is a bdd oper
Theorem on contiuity and boundedness of linear transformation on normed spaces part 1
T is continuous on N if and only if T is bounded and two other part #functionalanalysis
bounded linear operator.(part13)
Theorem on Continuous linear transformation UNIT-II
Bounded and Continuous Linear Operator || Definition || Functional Analysis || M.Sc 4th Semester ||
Normed Linear Space theorem Linear Transformation is continuous, bounded | by Sapna |
Every linear operator on a finite dimensional normed space is bounded#Functional Analysis - MGU
1.4: Continuous linear maps
Bounded linear functionals..Video
Functional Analysis 13 | Bounded Operators
Комментарии
 0:23:29
0:23:29
 0:28:08
0:28:08
 0:10:51
0:10:51
 0:24:48
0:24:48
 0:21:30
0:21:30
 0:43:46
0:43:46
 0:13:51
0:13:51
 0:14:13
0:14:13
 0:02:34
0:02:34
 0:06:05
0:06:05
 0:24:49
0:24:49
 0:10:59
0:10:59
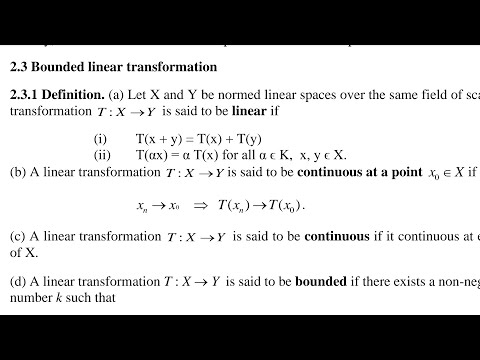 0:02:42
0:02:42
 0:13:56
0:13:56
 0:08:30
0:08:30
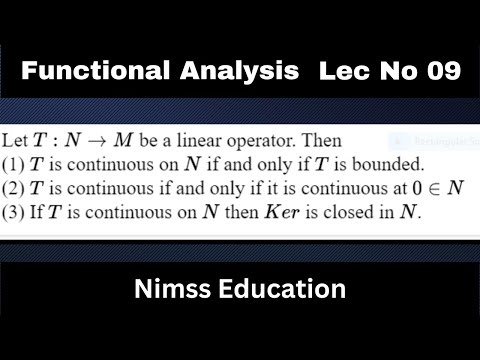 0:21:20
0:21:20
 0:00:05
0:00:05
 0:06:03
0:06:03
 0:09:18
0:09:18
 0:05:38
0:05:38
 0:06:34
0:06:34
 0:28:08
0:28:08
 0:14:34
0:14:34
 0:10:46
0:10:46