filmov
tv
5.3 Von-Neumann stability anslysis
Показать описание
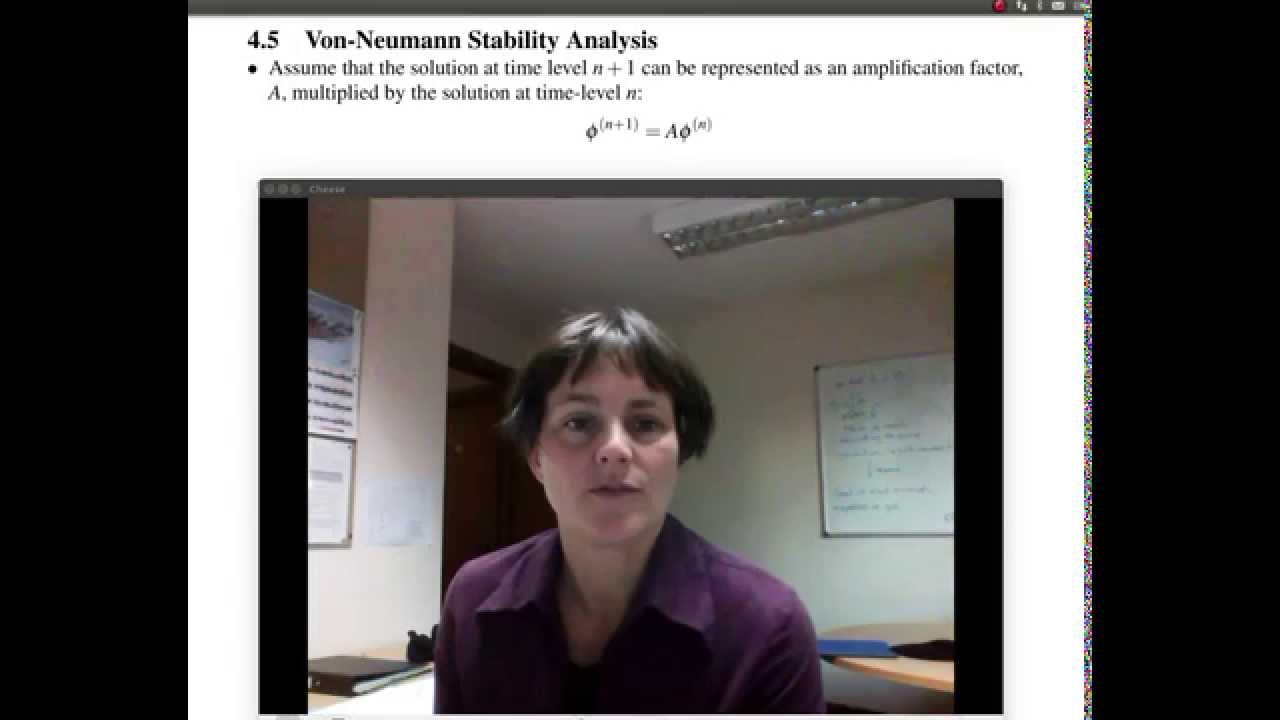
Von-Neumann stability analysis of linear advection schemes
Download the notes from
Download the notes from
5.3 Von-Neumann stability anslysis
6.3 Fourier-Von Neumann stability analysis
Von Neumann Stability Analysis of the FTCS Scheme | Lecture 70 | Numerical Methods for Engineers
Lecture 05 Part 3: von Neumann stability analysis of semi-discretized PDE
Stability Analysis Fourier Approach von Neuman
Numerical partial differential equation lecture 9 (Von Neumann Stability Analysis ut=auxx)
Von Neumann Stability Analysis of the Crank Nicolson Method
MIT Numerical Methods for PDE Lecture 7: von Neumann stability analysis
von Neumann Stability Analysis for Finite Differencing
Von Neumann Stability Analysis
von Neumann stability analysis and stability of time integration
Lecture 05 Part 8: von Neumann stability analysis of upwinding scheme
CFD Stability Von Neumann Stability Analysis
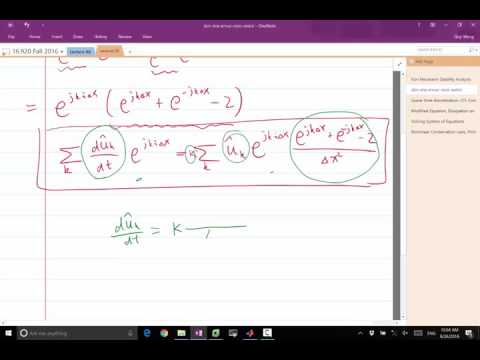
Lecture 05 Part 1: von Neumann stability analysis of continuous PDE
Lecture 05 Part 5: von Neumann stability analysis of fully-discretized PDE
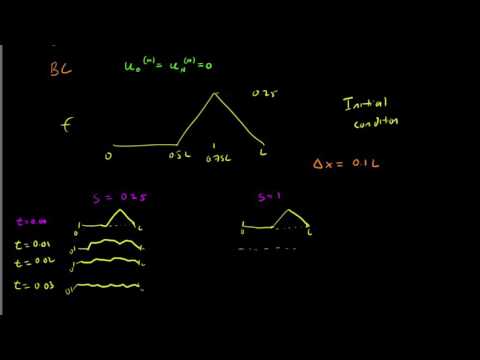
Parabolic Equations - Numerical Stability Analysis Using von Neumann Method
The Von Neumann Stability Analysis
Van Neumann Stability Analysis of the Crank Nicolson Implicit Differencing Method
Lecture 05 Part 7: von Neumann stability analysis of linear advection
Homework 4 Part 5 - Van Neumann Stability Analysis of Crank Nicolson Method
The Von Neumann Stability Analysis
ASTR 506 - Class 18- Vid 3- Numerical Hydrodynamics - von Neumann stability analysis of naive scheme
Discussions on von Neumann stability analysis
Von Neumann Stability Analysis Crank Nicolson Method
Комментарии
 0:14:05
0:14:05
 0:05:53
0:05:53
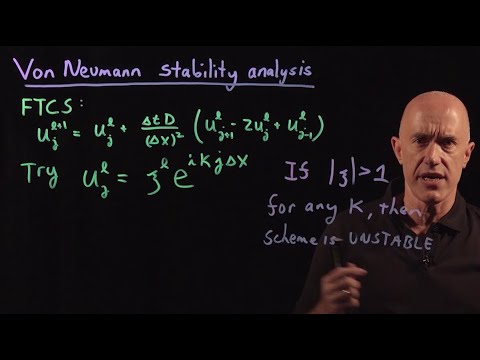 0:14:42
0:14:42
 0:10:28
0:10:28
 0:07:54
0:07:54
 0:07:39
0:07:39
 0:10:10
0:10:10
 0:14:37
0:14:37
 0:11:33
0:11:33
 0:07:06
0:07:06
 0:14:53
0:14:53
 0:15:00
0:15:00
 0:05:35
0:05:35
 0:05:34
0:05:34
 0:11:49
0:11:49
 0:15:51
0:15:51
 0:15:42
0:15:42
 0:09:25
0:09:25
 0:06:34
0:06:34
 0:09:26
0:09:26
 0:16:06
0:16:06
 0:01:50
0:01:50
 0:07:32
0:07:32
 0:14:01
0:14:01