filmov
tv
Lecture #13: Vertex Operator Algebras (13/20); W‐algebras

Показать описание
QM Masterclass by Thomas Creutzig (University of Alberta) held at University of Southern Denmark. Organized and funded by Centre for Quantum Mathematics.
#QMsdu #QuantumMathematics #IMADAsdu #masterclass #SDUdk #VertexOperatorAlgebras #algebra
#QMsdu #QuantumMathematics #IMADAsdu #masterclass #SDUdk #VertexOperatorAlgebras #algebra
Lecture #13: Vertex Operator Algebras (13/20); W‐algebras
Evgeny Feygin — Vertex operator algebras. Lecture 13.
Chris Beem - Comments on Vertex Operator Algebras for N=2 SCFTs
Kyoto U. 'Vertex Operator Algebras and Integrable Systems' L.2
Gaetan Borot - Airy structures and W-algebra modules II / Vertex Operator Algebras
Prof. Terry Gannon | Hypergroup orbifolds of vertex operator algebras
Evgeny Feygin — Vertex operator algebras. Lecture 14.
Permutation Orbifolds of Vertex Operator Algebras
Kyoto U. 'Vertex Operator Algebras and Integrable Systems' L.4
Miroslav Rapcak - Vertex Algebras at the Corner
Leonardo Rastelli - Higgs branches, vertex operator algebras and modular differential equations
A commutative vertex algebra.
Factorization algebras from fibrations and toroidal vertex algebras - Mathew Szczesny
International School on Representation theory, Vertex and Chiral Algebras - T. Gannon - Lecture 02
Salsa Night in IIT Bombay #shorts #salsa #dance #iit #iitbombay #motivation #trending #viral #jee
Vertex Algebras and Associated Varieties (Lecture 1) by Anne Moreau
The vertex algebra of one free boson: Part 1: The field.
Lecture #17: Vertex Operator Algebras (17/20); VOAs in physics, geometry and topology
Christopher Beem - Vertex Algebras and Superconformal Field Theories in Four Dimensions
This chapter closes now, for the next one to begin. 🥂✨.#iitbombay #convocation
International School on Representation theory, Vertex and Chiral Algebras - T. Gannon - Lecture 03
1st yr. Vs Final yr. MBBS student 🔥🤯#shorts #neet
Introduction to vertex algebras and their representations I - Tomoyuki Arakawa, RIMS, Japan
Vertex Algebras | The non-negative products.
Комментарии
 0:42:20
0:42:20
 1:11:13
1:11:13
 0:57:00
0:57:00
 2:38:06
2:38:06
 1:09:54
1:09:54
 0:57:01
0:57:01
 1:08:13
1:08:13
 0:52:54
0:52:54
 2:00:33
2:00:33
 0:20:17
0:20:17
 0:58:22
0:58:22
 0:34:40
0:34:40
 0:58:54
0:58:54
 1:27:12
1:27:12
 0:00:14
0:00:14
 1:05:02
1:05:02
 0:39:00
0:39:00
 0:49:43
0:49:43
 0:27:21
0:27:21
 0:00:16
0:00:16
 1:37:49
1:37:49
 0:00:20
0:00:20
 1:07:27
1:07:27
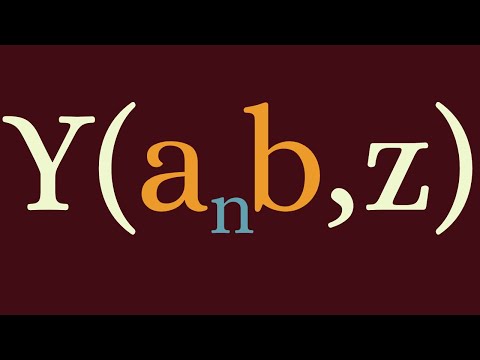 0:24:02
0:24:02