Robert Lemke Oliver - An effective Chebotarev density theorem for fibers - FRNTD Spring 2020

September 26th, 2020 Front Range Number Theory Day
Speaker: Robert J. Lemke Oliver
Title: An effective Chebotarev density theorem for fibers
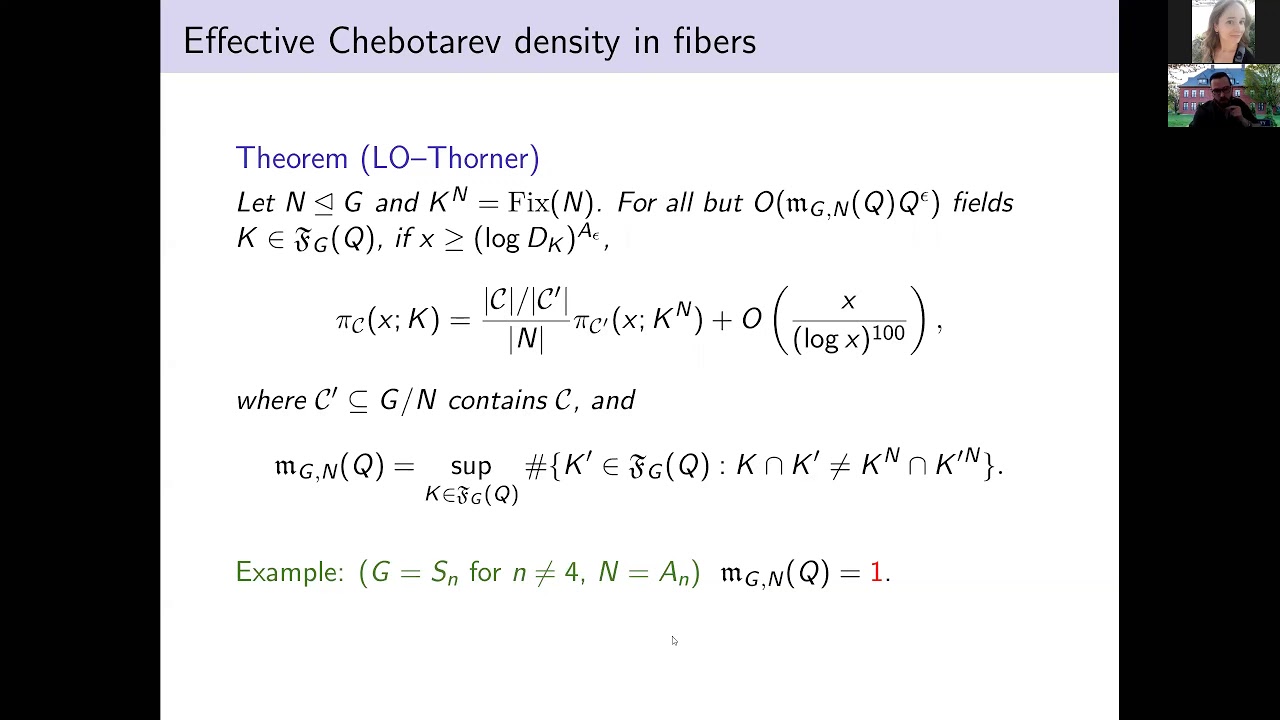
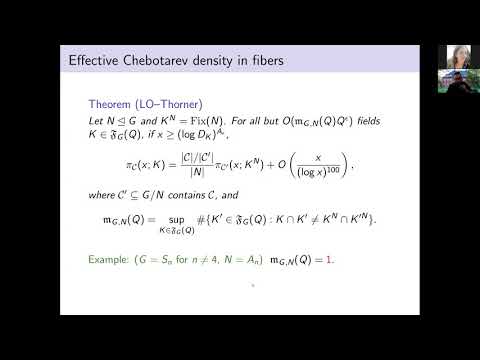
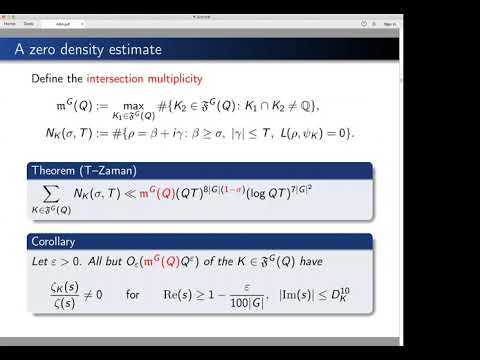
Abstract: The Chebotarev density theorem asserts that, in a normal extension K/k, the number of primes of k with norm at most x whose Frobenius element in Gal(K/k) lies in a specified conjugacy class C is proportional to the size of C. Lagarias and Odlyzko made this theorem
effective by determining a lower bound on x for which it’s guaranteed there are many primes p of k with Frobp ∈ C and norm at most x. Unfortunately, this lower bound requires x to be larger than any fixed power of the discriminant DK of K, and for this reason, in many applications where the size of the primes in question matter, one must appeal to much stronger results that are only available under the assumption of the generalized Riemann hypothesis.
In a recent breakthrough, Pierce, Turnage-Butterbaugh, and Wood showed that nearly GRH-quality results are available for “almost all” extensions K/k in a family, provided that the Artin conjecture is known for every field in the family; the dependence on the Artin conjecture was subsequently removed by Thorner and Zaman. However, an obstacle in this work is the possible presence of intermediate normal extensions, and for this reason it is also conditional upon progress toward the so-called “discriminant multiplicity conjecture” and imposes restrictions on the ramification of K/k. In forthcoming joint work with Jesse Thorner, we prove an unconditional result that allows these obstacles to be bypassed in many cases of interest. For example, we show that almost all degree n Sn-extensions have GRH-quality bounds on the ell-torsion subgroups of their class groups, and we determine a lower bound on the extremal order of the class number of degree n extensions that agrees with GRH-quality upper bounds.