filmov
tv
Periodic Functions and the Laplace Transform

Показать описание
We've seen previously in the playlist that Laplace Transforms work great with piecewise functions, functions that have discontinuities in them like the Heaviside or unit step function. In this video we're going to see that it also plays really nicely with periodic functions where f(t+p)=f(t). Indeed, you can always extend a function on an interval to a periodic function on the entire real line and we will have a nice formula from the Laplace Transform to deal with that. This is particularly useful for solving Ordinary Differential Equations (ODE) that have a periodic nonhomogeneity.
****************************************************
Other Course Playlists:
***************************************************
► Want to learn math effectively? Check out my "Learning Math" Series:
►Want some cool math? Check out my "Cool Math" Series:
****************************************************
*****************************************************
This video was created by Dr. Trefor Bazett. I'm an Assistant Teaching Professor at the University of Victoria.
BECOME A MEMBER:
MATH BOOKS & MERCH I LOVE:
Комментарии
 0:08:13
0:08:13
 0:17:35
0:17:35
 0:16:56
0:16:56
 0:07:09
0:07:09
 0:56:55
0:56:55
 0:03:57
0:03:57
 0:34:27
0:34:27
 0:12:10
0:12:10
 0:31:53
0:31:53
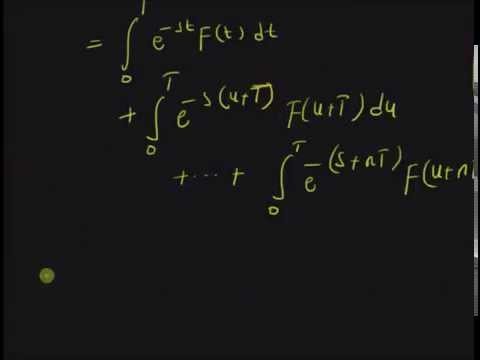 0:06:11
0:06:11
 0:12:48
0:12:48
 0:09:50
0:09:50
 0:06:40
0:06:40
 0:10:58
0:10:58
 0:10:33
0:10:33
 0:09:53
0:09:53
 0:14:35
0:14:35
 0:32:55
0:32:55
 0:08:26
0:08:26
 0:06:52
0:06:52
 0:08:06
0:08:06
 0:07:44
0:07:44
 0:06:49
0:06:49
 0:08:53
0:08:53