filmov
tv
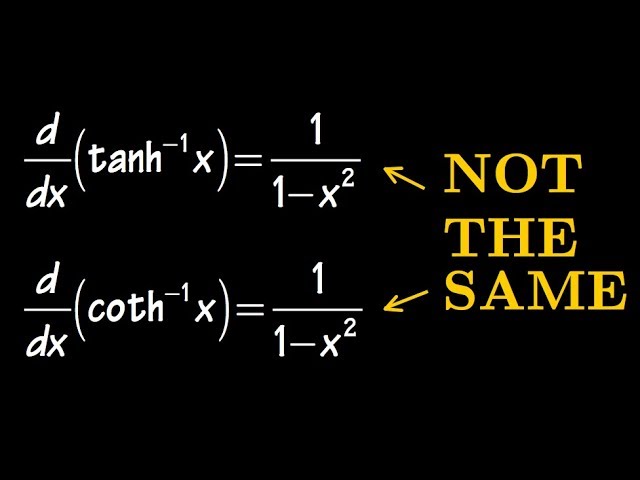
THE CONFUSING DERIVATIVES
Показать описание
So did the derivatives of tanh^-1(x) and coth^-1(x) and found out they are the same! But.... are they???
THE CONFUSING DERIVATIVES
he's a 10 but he confuses integrals and derivatives
Confused as to why this is wrong. Derivative product & chain rule. Reddit r/calculus
What's the Derivative??
Derivative of an imaginary number?
Sign of the Derivative (1 of 7: Differentiation review questions)
A Basic Introduction to Derivatives
How to Use the Product Rule | Calculus Derivatives #shorts
Challenging Math Olympiad Geometry Problem #matholympiad #math
Conceptually Learn Derivatives in 10 minutes!! [Calculus]
Power Rule and Exponential Function - Don't Get Confused! | Derivative | Calculus | Glass of Nu...
4 Potential Cases of Confusion With Derivatives
What’s the difference between average rate of change and a derivative? #shorts
Derivatives Chain Rule: Step-by-Step exercises.
Finding the Derivative of an Inverse Function Explained #maths #apcalculusab #algebra
What is a derivative?
Difference Between Partial and Total Derivative
Higher Derivatives and Their Applications
3 more Derivatives from Spivak (the middle one is so confusing:)
Master the Derivative Rules in just 30 minutes!
Know the difference between derivations Integral #shorts #mathematics #derivatives #integration
Not So Fast!! A partial derivative that makes no sense
Calculus - What is a Derivative? (7 of 8) A Function Describing a Change (In a Function)
Calculus - What is a Derivative? (1 of 8) Overview
Комментарии
 0:12:32
0:12:32
 0:00:11
0:00:11
 0:06:07
0:06:07
 0:00:29
0:00:29
 0:00:18
0:00:18
 0:04:54
0:04:54
 0:04:37
0:04:37
 0:00:12
0:00:12
 0:12:48
0:12:48
 0:09:12
0:09:12
 0:02:35
0:02:35
 0:10:28
0:10:28
 0:00:11
0:00:11
 0:17:38
0:17:38
 0:01:00
0:01:00
 0:02:17
0:02:17
 0:01:44
0:01:44
 0:07:29
0:07:29
 0:11:35
0:11:35
 0:34:43
0:34:43
 0:00:21
0:00:21
 0:11:24
0:11:24
 0:04:06
0:04:06
 0:06:49
0:06:49