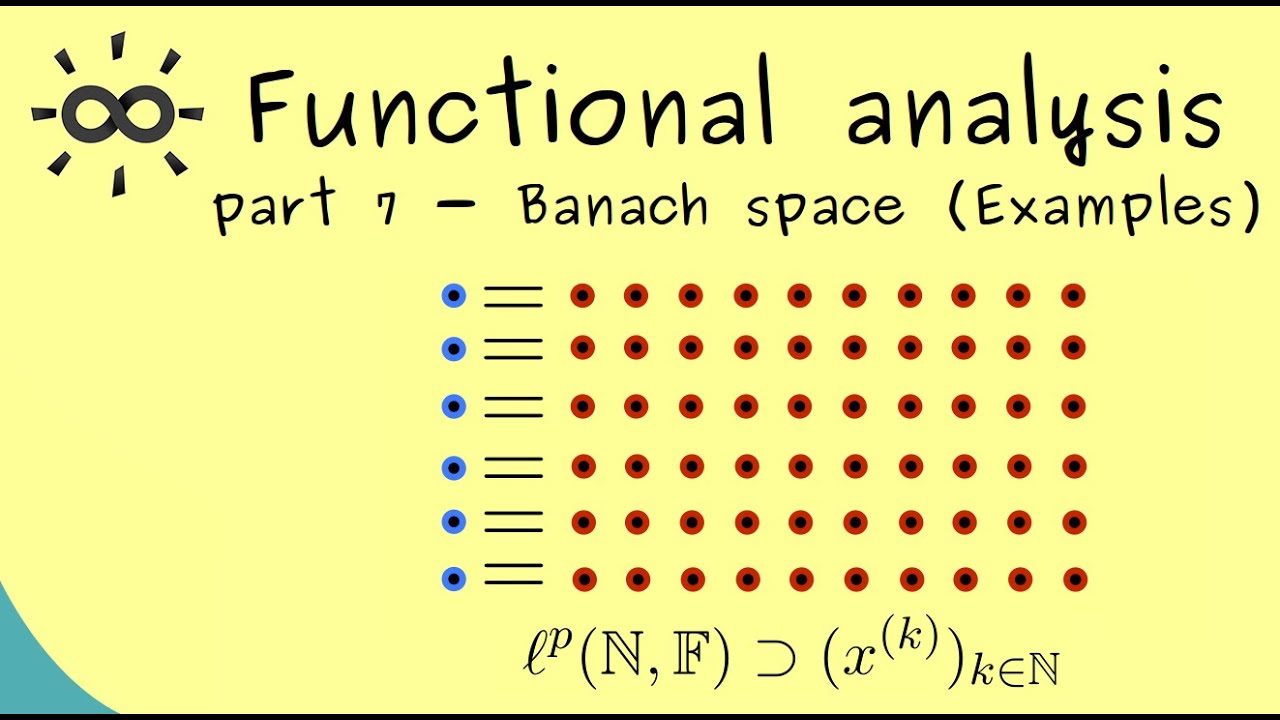
Functional Analysis 7 | Examples of Banach Spaces

Please consider to support me if this video was helpful such that I can continue to produce them :)

🙏 Thanks to all supporters! They are mentioned in the credits of the video :)

This is my video series about Functional Analysis where we start with metric spaces, talk about operators and spectral theory, and end with the famous Spectral Theorem. I hope that it will help everyone who wants to learn about it.

x

00:00 Introduction
00:30 One-dimensional example
01:21 Zero-dimensional example
02:10 l^p-space

#FunctionalAnalysis
#Mathematics
#LearnMath
#calculus

I hope that this helps students, pupils and others. Have fun!

(This explanation fits to lectures for students in their first and second year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)

[이효건-oo]

This is a masterpiece, piercing most important things in a video around 10 minutes

[evolindica]

Very helpful and concise. Thank you very much for making the series of functional analysis!

[ROni_ROmio]

Please...we hope to get one course about type of convergence ...proba..a.s....Lp ..distribution ..and relation between them😍😍😅😅🤗🤗

[rajshreejoshi]

Mathematics means headache for most of the people and MSc in mathematics is on another level.
Teachers don't take your syllabus seriously they just need their payment on time.
But tutors like you not just hope of students like us but also give us new vision to look at mathematics.
Praise from India. 🙏❤️

[stevilimfax]

I think that you lectures perfectly and always hit the center of a topic! i have just one objection that your cursor is really small and when you are showing something on a board it is really hard to find it :D, never the less keep on going!

[zhcjzry]

Thank you so much for your videos, they are very clear and pleasant! Looking forward to the next episode

[GODSONNWOSU-nbor]

Straight to the point with no ambiguity, thanks for sharing ✌️

[scollyer.tuition]

There's a tiny omission in this video: when you talk about l^p spaces, you are considering the general case of the field F being either R or C, and you say that you mentioned that Cauchy => complete with the usual norm in the first example for these fields.

In fact, the first example only states this fact for R; it is of course true for C too, but it may have been clearer to have treated C as a separate example. Of course, I'm being a little picky here.

Apart from that, nice video. I think it was very helpful to omit the proof of the l^p norm at this stage.

[ahippiething]

Thank you so much for the amazing videos! I just started taking functional analysis and I can only understand the subject if I watch your videos

[farzanahdarwish]

Amazing! These videos are really useful and comprehensive. Thank you.

[sparrowpower]

A suggestion: Try to write in the middle of the screen. You usually write in the lower part of the screen which causes difficulty to watch on youtube because most of the functions of youtube are also in the lower part of the screen.

[safayassin]

those videos are helping me a lot, thanks!

[PunmasterSTP]

Examples of Banach spaces? More like "Astounding mathematics teaching-places!" These videos are amazing; thanks so much for making them.

[arturo]

By assumptions all individual x^(k)'s themselves belong to l^p, so the horizontal sequence converge (is that right). Now I'm a bit confused about the vertical sequences, why do we assume the vertical sequences x^(k) are a cauchy sequence ? Furthermore, we want to show that all Cauchy sequence in X converge, so we assume that we can put all these sequence vertically and call it a cauchy sequence. Wouldn't we have to prove this? How do we know this set of sequences (that converge in lp by definition) form themselves together a Cauchy sequence and how do we know these form all the Cauchy sequence ?

In other words how do we know that all Cauchy sequences will appear when we iterate through x^k(s) ? Thanks a lot

[Studiacapta-qobo]

Ich jetzt versuche Deutsch zu lernen und es würde mir sehr sehr hilfreich sein wenn du diese Videos auch auf Deutsch übersetzen könntest. Danke schön immer für diese tollen Videos!

[xwyl]

I watched this at least six times to understand the proof. There are 2 obstacles:
1. The English is hard to understand sometimes. A subtitle would help a lot.
2. Crucial logical steps should be as clear and eye-catching as possible. These steps should better have at least pop-up dialogues with crucial contents in them. When some steps use previous definitions, more stress and clarity should be added (maybe pop-ups plus screenshots of the definitions with hilights).
Wish you make the Bright Side much brighter, and rescue more from the dark side.

[mathieumaticien]

I understood up to the point where you said x_n^(k) -> x*_n for all k.

But I don't understand why you did the whole thing with epsilon' and the less than or equal to part. You have that
||x^(k) - x*||^p = lim_N lim_l sum(|X_n^(k) - x_n^(l)|^p),
where each absolute values are strictly < epsilon (no prime)?

I'm just lost on where you got
||x^(k) - x*|| <= epsilon', and why that step is needed in the first place.

[ekeebobs]

Thank you very much sir! Your video's have been helpful but I've got a suggestion.


Please make use of a visible poster of maybe color the items you are talking about so we won't be misled. The pointer being used is small and I got confused sometimes when watching the video. Thanks again for the video's.

[ferry]

can I ask something about F, is F can be defined for scalar in vector space that is F is field in general? not just real and complex number

[qiaohuizhou]

2:13 Lp space
4:37 lp is Banach space