filmov
tv
Functional Analysis 21 | Isomorphisms
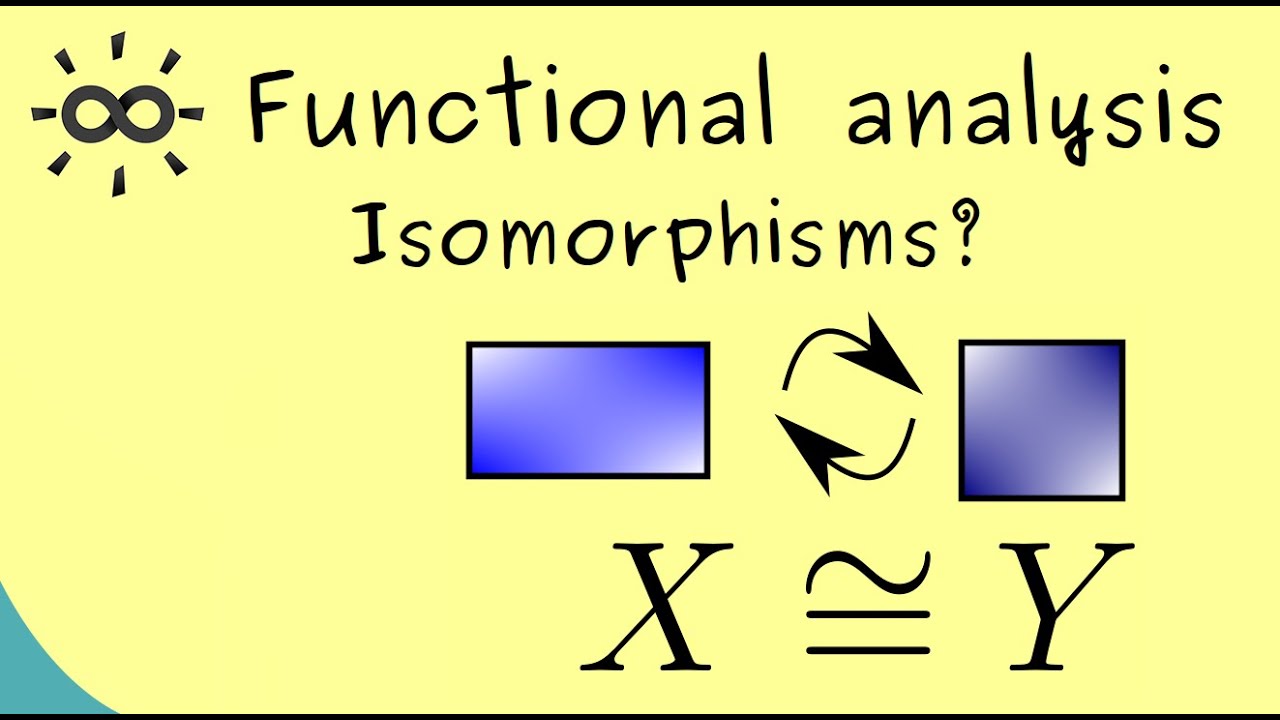
Показать описание
Please consider to support me if this video was helpful such that I can continue to produce them :)
🙏 Thanks to all supporters! They are mentioned in the credits of the video :)
This is my video series about Functional Analysis where we start with metric spaces, talk about operators and spectral theory, and end with the famous Spectral Theorem. I hope that it will help everyone who wants to learn about it.
x
00:00 Introduction
00:50 Example
04:18 Isomorphism
07:31 Examples
#FunctionalAnalysis
#VectorSpaces
#Mathematics
#LearnMath
#calculus
I hope that this helps students, pupils and others. Have fun!
(This explanation fits to lectures for students in their first and second year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)
Functional Analysis 21 | Isomorphisms
Functional Analysis Module III Class 26 Isomorphism and Hilbert dimension
Isometric isomorphism definition in m.sc functional analysis ✨
21. Definition and examples of isomorphism | if dim(V(F))=n then V(F) is isomorphic to F^n(F)
Functional Analysis Module III Class 21 Convergence of Fourier Series
M Sc II|Maths. 2019 Pat. | MTUT131 Functional Analysis |Isometric Isomorphism of B(N) into B(N*) - I
This chapter closes now, for the next one to begin. 🥂✨.#iitbombay #convocation
1.6: Isomorphisms
Functional Analysis#21,#Dual space II (Hahn Banach thm II), 3rd sem Msc Maths, #University
Anushka Ma'm dancing💃in Pankaj sir wedding 😍 | Anushka Mam physics wallah | #neetaspirant #stud...
Abstract Algebra - 6.2 Examples and Non-Examples of Isomorphisms
A-Calculus, exchange of differentiability through isomorphism, 5-22-21 part 5
I met @AmanDhattarwal bhaiya | Lovely Professional University
Abstract Algebra - 6.1 Definition and Understanding of Isomorphisms
Lecture 21 - First isomorphism theorem
A-Calculus, solving quadratic equations and isomorphism, 5-22-21 part 2
Lecture#79 Isomorphism on a Group
Isomorphism of Two Graphs #shorts
Pakistan education system what a beautiful environment WOW🤣🤣
🤔👆🤔'Isomorphism under Q' 🤔👆🤔 #mathsacademy
M Sc II| Maths. 2019 Pat. |MTUT131 Functional Analysis |Isometric Isomorphism of B(N) into B(N*) -II
Functional Analysis Overview
Group Theory 18: Definition of isomorphism
M Sc-2/Functional Analysis/Isomorphic Isomorphism/Class-11
Комментарии
 0:10:39
0:10:39
 0:37:34
0:37:34
 0:00:58
0:00:58
 0:10:05
0:10:05
 0:22:44
0:22:44
 0:16:51
0:16:51
 0:00:16
0:00:16
 0:33:05
0:33:05
 0:38:29
0:38:29
 0:00:31
0:00:31
 0:15:54
0:15:54
 0:01:42
0:01:42
 0:00:25
0:00:25
 0:20:41
0:20:41
 0:23:34
0:23:34
 0:59:51
0:59:51
 0:00:56
0:00:56
 0:00:25
0:00:25
 0:00:16
0:00:16
 0:00:23
0:00:23
 0:23:13
0:23:13
 0:49:36
0:49:36
 0:05:10
0:05:10
 0:33:47
0:33:47